Profile
Settings
Refer your friends
Sign out
Spring mass systems are a combination of a fixed heavy load tied to one end of spring. These systems follow Hooke’s law that the restoring force is directly proportional to the displacement and the restoring force acts in a direction opposite to that of the displacement. A combination of such systems can be arranged in either parallel or series arrangements.
What Are Spring-Mass Systems
A spring-mass system is a system wherein a spring is attached to a load via its free end. From the other end, the spring is attached to a support.
A spring-mass system is commonly used in simple harmonic motion to determine the time period of an object in such motion. It also has certain interesting applications explained in the later section.
If spring and a mass system are arranged in one horizontal and one vertical arrangement, the systems freely oscillate identically at an equilibrium position.
Hooke’s Law
Hooke’s law is a simple quantitative method to study simple harmonic motion. It says that the simplest oscillations occur when the restoring force is directly proportional to the distance.
It can also be depicted as
F= – kx
Where F is the restoring force, x is the displacement and k is a constant known as the spring constant or force constant, which relates to the difficulty in reforming the system. The negative sign indicates that the restoring force happens to be acting in a direction opposite to that of the displacement. The spring-mass system follows simple harmonic motion due to the fact that the restoring force is directly proportional to the displacement.
What Can Be The Possible Arrangements (Series) (Parallel)
Two or more spring-mass systems are said to be arranged in series if they are arranged end-to-end or point-to-point and in parallel, if they are connected side-by-side. The combination works as a single spring, as they are connected to each other.
More on the same, it can be said that two or more spring-mass systems are said to be connected in series when external stress applied to the ensemble gets applied to each string without any change in the magnitude. The amount of strain or deformation on the ensemble is the sum of strains on the individual springs.
The spring-mass system is said to be arranged in parallel when the strain on the ensemble is common for the two and the stress of the ensemble is the sum of the stresses of individual springs.
The formulas for the spring-mass system combinations are just in analogies to those in the capacitor combinations in electrical circuits, connected in series and parallel.
In order to understand about the systems, we first refer to the formulas used in these series and parallel arrangements of the spring-mass systems:
For the series combination
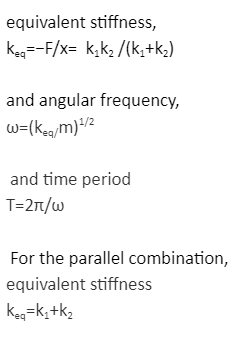
so, angular frequency,
ω=(keq/m)½
Conclusion
In conclusion, it can be noted that spring-mass systems do play an important role in the engineering domain as well as other fields too. The spring-mass systems are developed on the basis of the following simple harmonic motion or Hooke’s law. Hooke’s law states that the restoring force acting on a spring-mass system is directly proportional to the displacement the system has undergone and this force will act in a direction opposite to that of the displacement. All the systems following this law are known to have simple harmonic motion and such is the spring-mass system. Now a combination of spring-mass systems can be made as per our needs of restoring force and these combinations can be arranged in multiple ways. Two common ways are their arrangement in series and parallel, the definitions and details of which you will find in the text.
