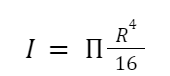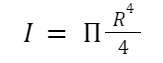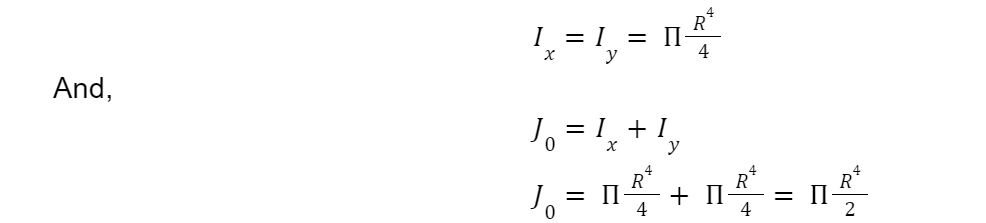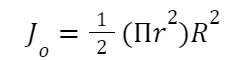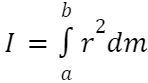Profile
Settings
Refer your friends
Sign out
First and foremost, let us define the idea of the moment of inertia in simple words. It may be deduced that inertia is proportional to a body’s mass. Whenever an object begins to rotate along a fixed axis, each component in the object moves in a circle with constant speed, indicating that each particle moves with rotational movement. Indeed, the correct meaning of the angular momentum or the MOI is the tendency of an object to resist angular momentum. It is defined as the product of the particle’s mass in the object and its length’s square from the rotational axis.
To find the moment of inertia of a quarter circle about its centroid is similar to finding the moment of inertia of a circle. The procedure for deriving the moment of inertia of a quarter circle is to find the moment of inertia of a circle and divide the result by two. In a circle, the MOI of it about the x-axis is similar to that of the y-axis.
Before deriving the formula let us first have a look at the moment of inertia of a quarter circle which is given by the equation,
Where R is the radius of the quarter circle and I is the moment of inertia.
We should also learn about the moment of inertia of a circle which is given by the equation,
Where again R is the radius of the circle and I is the moment of inertia
Derivation
Before starting the derivation of the moment of inertia of a quarter circle let us make a diagram of a quarter circle to understand the annotations and other parameters.
Consider a quarter circle having its centre of mass at location c and a radius r.
To start the derivation first let us bring the moment of inertia of a circle.
Now, as said above we will derive the equation of the moment of inertia of a quarter circle by dividing the moment of inertia of a circle by two.
As, we also know, the moment of inertia of a circle is the same as the x and y-axis.
Therefore,
To determine the moment of inertia of a circle, we can replace the mass with the area represented by A.
Therefore,
Equation becomes as,
Now, to find out the moment of inertia of a quarter circle we can divide the moment of inertia of a circle into its quarters. Moreover, the circle part revolving around a particular axis will always be symmetric therefore will have similar values on the x and y-axis both.
Therefore,
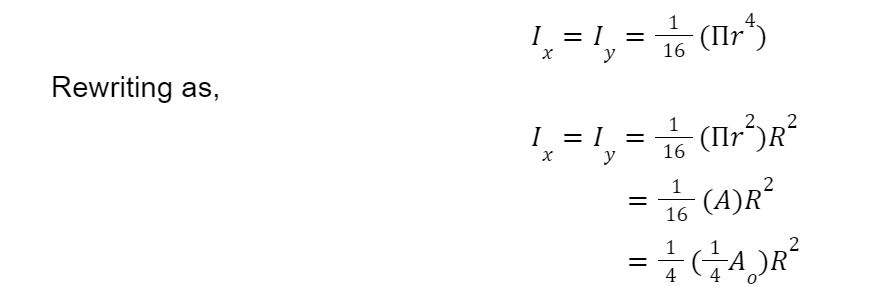
Now, the time comes for finding the moment of inertia of a quarter circle. Therefore for this purpose, we’ll add Ix and Iy.
So, the moment of inertia of the quarter circle about the origin of it,
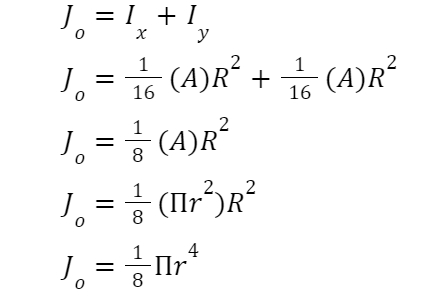
Which is the formula of the moment of inertia of a quarter circle when taken about the centroid of the quarter circle or the circle. Where Jois the moment of inertia and r is the radius of the quarter circle.
In the derivation above R and r are the same.
Conclusion
To sum up, the moment of inertia occurs when a body rotates about any particular axis of rotation. It is mainly the resistivity experienced by the body or any particle when the angular momentum or the rotation starts.
To derive the moment of inertia of a quarter circle we can first use the same derivation of a circle and then divide the results obtained from it and divide it by two. Also, a circle has the same moment of inertia about both axes.
