Determining The Moment Of Inertia Of Flywheel

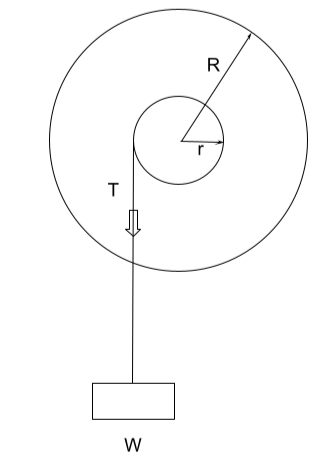
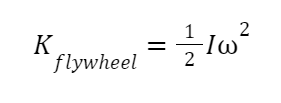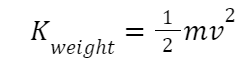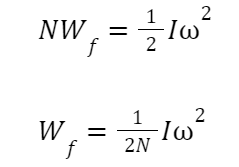The flywheel is made out of large circular ring wheels with a robust axle protruding along either end. The shaft is supported by two permanent supports and is placed on rolling elements. Also on the shaft or the axle, there consists of a little peg. The mass is carried by one end of a string that is lightly coiled all around the peg.

Speaking in terms of its usage it is a mechanical device that saves the rotational energy by saving the angular momentum. Overall, it is kinetic energy that is directly dependent on the moment of inertia of the and the speed’s square. The MOI of the flywheel always remains constant but the kinetic energy remains dynamic because of the changing rotational speed of the flywheel. An interesting fact is that in 1802 a steam locomotive engine flywheel for distributing its single cylinder’s power.

The moment of inertia of a flywheel is based on some assumptions we will determine in this article and by considering the same flywheel during the derivation.

Initially let us have a look at the formula for the moment of inertia of a flywheel. It is given by the equation,

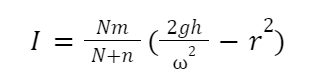
Where,

I is the flywheel’s moment of inertia, m is the mass of the ring, N determines the rotation of the flywheel, n determines the winding numbers, h is the weight assembly’s height, g is as we all know the gravity value i.e., (9.81 m/s) and r is the axle’s radius. 

Moreover, it is also determined by the expression,

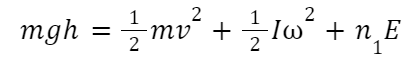
Derivation

For derivation let us initially discuss the diagram of a flywheel and consider a few parameters and annotations which are needed during the derivation.

Consider a weight of mass m hanging on a thread rolled on a rolling ring. Where the height of the ring is h. Considering it in a dynamic situation when we roll the ring the weight will come down to a new height. Subsequently, the mass will have a loss in its potential energy. And the equation for the same loss can be written as,

P_loss=mgh

At the same time when the weight came down to a new height the flywheel and the axle rotated which increased the kinetic energy of the system. The equation for which is written as,

Where,

I is the moment of inertia and is the angular velocity of the flywheel. 

Moreover, when the flywheel gained kinetic energy the weight coming down also gained some kinetic energy which is expressed as,

Where v is the linear velocity of the weight coming down

When the weight came down there was some friction produced by rotating the still flywheel, some work was done on it. Taking that into account, the equation for the work done will be,

W_friction=nW_f

As the flywheel generates when the rope slid over the circular ring and generated friction. That friction will depend on the number of windings the rope had on the ring. Therefore, in the equation above n denoted the number of winding of the rope or the string. And W_f is the work done to overcome the torque of friction.

Now, 

According to law of conservation of energy,

P_loss= K_flywheel + K_weight + W_friction

Substituting the values of the attributes in the above equation, the equation becomes as,

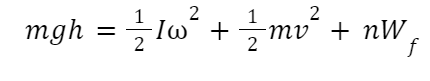
Now, once the flywheel has rotated, its assembly’s kinetic energy is used to rotate the system N times against the torque friction, the work done will be written as,

Moreover, we have a relationship between the velocity of the assembly weight and an axle’s radius in physics which is given by the equation,

v=ωr

Where v is the velocity of the weight and r is the axle’s radius 

Substituting the values of v and W_f in the mgh equation as,

Solving the equation for I we will get the required moment of inertia of a flywheel formula which is,

Conclusion

To sum up, a flywheel is a kind of device which is used to store energy in the form of kinetic energy. A flywheel’s moment of inertia always remains the same as the shape and section remain the same. However, the speed of rotation of the flywheel changes the kinetic energy of the flywheel. A flywheel works on the concept of moment of inertia.

To find or derive the moment of inertia of a flywheel one has to consider the same phenomena as happening in a flywheel, a rotating ring at a height, a weight or mass which is suspended on the ring having few loops and a wheel and axle. And consider the weight to fall down that decreases the potential energy and increases the kinetic energy of the weight. And use the same consideration on the equations.