Profile
Settings
Refer your friends
Sign out
A part of the tangent which belongs to a curve and lies between the point of tangent and the interception of a tangent with the x-axis is known as the length of the tangent. The part of the x-axis which lies between the interception of the tangent and the coordinate x of the point of tangent is called a subtangent. Hence, we can say in simple words that a subtangent is a projection of a part of a tangent that intersects on the x-axis. Whereas a subnormal can be termed as a projection of a part of normal which lies on the x-axis.
Subnormal
A part of the tangent that lies between the interception of a normal with the x-axis and the tangency point x-axis coordinate can be termed as the length of normal. A part of the x-axis which lies between the interception of a normal with the x-axis and the tangency point x-axis coordinate can be termed as the length of a subnormal. Hence, we can say that a subnormal can be termed as a projection of a part of normal which lies on the x-axis.
Subtangent
A part of the tangent which belongs to a curve and lies between the point of tangent and the interception of a tangent with the x-axis is known as the length of the tangent. The part of the x-axis which lies between the interception of the tangent and the coordinate x of the point of tangent is called a subtangent. Hence, we can say in simple words that a subtangent is a projection of a part of a tangent that intersects on the x-axis.
Practical Definition
y = f(x) is the curve that can be differentiated at point P. The point of tangent and normal to the curve be P (x, y) which intersects the x-axis at the points T and N respectively. Whereas M is the projection from the x-axis to the point P. According to the given figure, we can conclude;
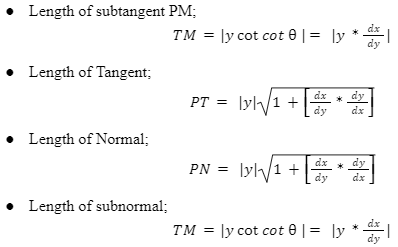
- Length of the tangent is PT
- Length of the normal is PN
- Length of the subtangent is TM
- Length of the subnormal is MN
Formulae
The formulas for subnormal and subtangent can be written as:
Example
- 2x2 + y2 = 12 is the equation of normal to the curve at (2, 2) then what will be the point at which the curve will intersect the normal again?
- (-22/9, -2/9)
- (22/9, 2/9)
- (-2, -2)
- None of the above
Solution
2x2 + y2 = 12 or dydx = -2x/y.
The slope of the normal is ½. The slope of AB is ½ and point B(-22/9, -2/9) lies on the curve. Therefore the curve intersects the normal again at (-22/9, -2/9).
- y3 − x2 y + 5y − 2x = 0, x4 − x3y2 + 5x + 2y = 0 are the two tangent to the curve which intersect at the origin at ∠θ =?
Solution
Differentiation of y3 − x2 y + 5y − 2x = 0 (w.r.t x)
We will get 3 y2 y′− 2xy − x2y′ + 5y′ − 2 = 0 (i)
Differentiation of x4 − x3y2 + 5x + 2y = 0 (w.r.t x)
We will get 4x2 − 3x2y2 − 2x3yy′ + 5 + 2y′= 0 (ii)
After plotting (i) and (ii) we get yi (0, 0) = 2/5, yii (0, 0) = -5/2, Hence the tangents intersect at right angles.
Conclusion
Subtangents and Subnormal is a very interesting topic related to geometry and we can find many sums where both the questions can be asked at the same time. Both the subtangent and subnormal are related to curves and normal. Whereas subtangent is a part of tangent and subnormal is a part of a normal. There can be various types of sums that can cover the whole topic of tangents and normal. This topic can be used to solve equations, find angels and a lot more.
