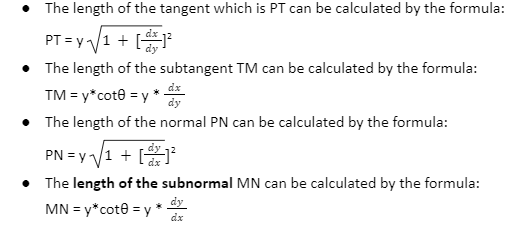
Let’s assume P = (x, y), which is a point on the given x-axis and another point C = (x, 0). Let’s take the line that intersects the x-axis be T and take the tangent as P. In this case, TA is referred to as the subtangent at the point P. And is normal of the curve point P crosses the x-axis at the point N then the point of AN is said to be as the subnormal. Here we come to the discussion that the tangent and normal are the lengths of PT and PN respectively and to get create confusion, tangent normal lines are also known as tangent and normal.
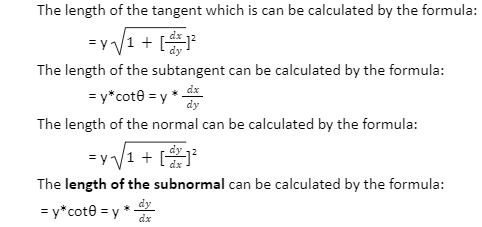
Formula of Subtangent and Subnormal
Here, let PT be the length of the tangent, PN is the length of the normal, TM is the length of the subtangent and MN is the length of the subnormal.
The formulas of subtangent and subnormal are:
For example:
The curve = ax3 + bx2 +cx +5 is touching the x-axis at the point P (-2, 0) and it intersects the y axis at Q where its slope is 3. Find a, b, c.
Solution:
Slope of the tangent to the curve at (x1, y1) is
[dy/dx] (x 1, y 1) = 3ax12 + 2bx1 + c
The point Q is (0, 5).
Since the curve passes through (-2, 0),
-8a + 4b – 2c + 5 = 0.
Since the slope of the tangent at (-2, 0) is 0,
12a – 4b + c = 0
Since the slope of the tangent at (0, 5) is 3,
c = 3.
From (1), (2) and (3), a = -1/2,b = -3/4, c = 3.
Practical Applications
- What is the equation of the tangent to the curve y = be[-x/a] at the point of intersection with the y axis?
Solution:
Here we have to apply the formula of subtangent and subnormal,
As it is given that y = be[-x/a] which is meeting the y axis at the point (0,b).
So here, y = be[-x/a] * (-1/a).
So, at (0,b) so dy/dx = be0 (-1 / a) = -b / a
So, the tangent which is required is y – b = -b/a (x-0) or x/a + y/b = 1.
- The length of the subtangent and the subnormal are given as ST and SN at a point = /2. So on the curve x = a( + sin), y = a(1 – cos ), a 1 then
- ST = SN
- ST = 2SN
- ST2 = aSN3
- ST3 = a SN
Solution:
Here we have to apply the formula of subtangent and subnormal.
dx/d = a (1 + cos θ), dy/d = a (sin)
dy/dx = / 2 = dy/dθdxdθ = a sinθa(1+cosθ = 1
Length of the given subtangent ST = ydydx = a1 = a
Length of the given subnormal SN = y*dydx= a. 1 = a
So here it proves that ST = SN.
Conclusion
The subtangent and subnormal’s definition gives us an idea of what is the difference between the subtangent and subnormal, it clearly states that a point which is stopping the tangent to go out of its axis in which the tangent is present is known as a subtangent and for subnormal it is normal which is stopped from going away from its axis.The formulas for calculating subtangent and subnormal are very important as these formulas give us the idea of how to find out the length of the subnormal or subtangent.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out