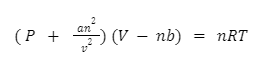Profile
Settings
Refer your friends
Sign out
The van der Waals Equation is an equation formula that computes the distance between two points. It alters the Ideal Gas Law as well as anticipates the features of real gases by defining particles of non-zero volume regulated by pairwise attractive forces. It is a thermodynamics equation of state that relies on the assumption that fluids are made up of non-zero volume particles which are attracted to each other. It could be used to determine a gas’s properties in non-ideal conditions.
Definition
The Van der Waals equation is a thermodynamics equation of state that relies on the assumption that fluids are made up of non-zero volume particles which are attracted to each other. It was derived from the work by Johannes Diderik van der Waals in theoretical physical chemistry in the late 1800s, as well as work on the attractive force that carries his name. The equation can be expressed to be based on a classic collection of derivations derived from Van der Waals’ and similar work, as well as a set of statistical thermodynamics-based derivations.
Derivation of the Van der Waals Equation
It is a state equation in chemistry & thermodynamics that expands the ideal gas law to allow for the impacts of interaction between molecules in a gas, as well as the limited size of the molecules. Gas molecules are treated as point particles by the ideal gas law, which means they don’t take up space or alter kinetic energy upon collisions
The Kinetic Theory of Gases is used to correct the pressure and volume of ideal gases, which leads to the Van der Waals equation. Another method is to utilise a derivation based on the particles’ potentials. All derivations, however, assist us in establishing the same link.
The ideal gas law says that at a temperature T, the volume V inhabited by n moles of any gas does have a pressure P determined by the following relation, wherein R is the gas constant:
PV = nRT
The Van der Waals equation substitutes V/n in an ideal gas law with Vm-b, wherein b is the volume created by inhabitation of molecules of one mole and Vm is the molar volume of the gas.
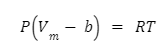
The ideal gas law has been modified once more to allow for interactions amongst gas molecules. Intermolecular interaction gained by adding to the documented pressure P, in the formula of the form a/ vm² , wherein a is the constant whose value is dependent on the gas.
As a result, the full Van der Waals equation is
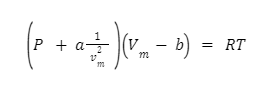
Measurements of Van der Waals Constant values Units of “a” and “b” are atm lit² mol-² and litre mol1 respectively.
It fits real-world pressure-volume-temperature data more than the ideal gas equation. Two parameters (named ” a ” and ” b “) that should be measured based on each gas are introduced to improve the fit. Since it embodies a basic physical image for the distinction among a real gas as well as an ideal gas, Van der Waals’ equation is especially valuable in our efforts to understand the behaviour of real gases.
We presume that the gas molecules need not interact while deriving Boyle’s law from Newton’s laws. Simple arguments indicate that this can only be true to an extent. Gas molecules should interact with each other in order to exist. They repel each other across short distances. They are attracted to each other over extended distances. An application of statistical thermodynamics can also be used to construct the ideal gas equation from the basic assumptions made in 10. We can use statistical thermodynamics to obtain van der Waals’ equation by making various assumptions about molecule characteristics.
The molecules must occupy a limited volume and be attracted to each other by a force that fluctuates as the reverse of a power of the length between them, according to the assumptions.
Conclusion
To conclude, the Van der Waals equation is a thermodynamics equation of state that relies on the assumption that fluids are made up of non-zero volume particles which are attracted to each other. It was derived from the work by Johannes Diderik van der Waals in theoretical physical chemistry in the late 1800s, as well as work on the attractive force that carries his name. It is expressed as:
