Profile
Settings
Refer your friends
Sign out
Centre of mass formulaThe formula for centre of mass are as stated below |
||
| Description | Formula | |
| Centre of mass of a system with n number of masses situated on a line at different positions | |
|
| Centre of mass of a system with n number of masses situated on a 2D plane |  |
|
| Centre of mass of a rectangular plate | The centre of mass of a uniform rectangular plate of length L and breadth B is given as: r_x=B/2 r_y=L/2 | |
| Centre of mass of a triangular plate | The centre of mass of a uniform triangular plate is given by the formula: r_c=h/3 Where, h is the height of the plate. | |
| Centre of mass of a semi-circular ring | The centre of mass of a semi-circular ring is given as: r_y=2R/π r_x=O Here, R is the radius of the semi- Circle. | |
| Centre of mass of a semi-circular disc | The centre of mass of a semi-circular disc is given as: r_y=4R/3π r_x=O Here, R is the radius of the semi- Circle. | |
| Centre of mass of a hemispherical shell | The centre of mass of a hemispherical shell is given as: r_y=R/2 r_x=O Here, R is the radius of the semi- Circle. | |
| Centre of mass of a solid hemisphere | The centre of mass of a solid hemisphere is given as: r_y=3R/8 r_x=O Here, R is the radius of the hemisphere. | |
| Centre of mass of a circular cone | The centre of mass of a circular cone is given as: r_y=h/4 Here, h is the height of the cone. | |
| Centre of mass of a hollow circular cone | The centre of mass of a hollow circular cone is given as: r_y=h/3 Here, h is the height of the cone. | |
Circular motionThe formula for circular motion are as stated below |
||
| Description | Formula | |
| Average angular velocity | ω_average=(θ_2-θ_1)/(t_2-t_1 )
 |
|
| Average angular acceleration |  |
|
| Tangential acceleration | a_t=dV/dt Here dV is the change in velocity over time dt. a_t=r dω/dt Here, r is the radius, dω is the change in angular frequency over time dt. | |
| Centripetal acceleration | a_c=v^2/r or a_c=ω^2 r Here, v is the linear velocity, r is the radius and ω is the angular frequency. | |
| Normal reaction on a body moving on a concave bridge | N=mg□cos cos θ +(mv^2)/r Here, m is the mass, g is the gravitational acceleration, θ is the angle, v is the linear velocity and r is the radius of the bridge. | |
| Normal reaction on a convex bridge | N=mg□cos cos θ -(mv^2)/r Here, m is the mass, g is the gravitational acceleration, θ is the angle, v is the linear velocity and r is the radius. | |
| Safe velocity of a vehicle on a level road | v_safe≤√μgr Here, v safe is the safe velocity, is the coefficient of friction, g is the gravitational acceleration and r is the radius. | |
| Banking angle | tan θ =v^2/rg Here, θ is the banking angle, v is the linear velocity, r is the radius of the curve and g is the gravitational acceleration. | |
| Centrifugal force | f=mω^2 r Here, f is the centrifugal force, m is the mass, is the angular velocity and r is the radius. | |
| Conical pendulum | T=2π√((L cos θ )/g) Here, L is the length of the pendulum, θ is the angle made by the string with the vertical and g is the gravitational acceleration. | |
De Broglie wavelength formulaThe formula for de broglie wavelength are as stated below |
||
| Description | Formula | |
| De Broglie wavelength |  |
|
| Radius of electron in hydrogen like atoms | r_n=n^2/Z a_0 Here, rn is the radius of nth orbit, a0 is a constant whose value is0.529×10^(-10) m m and z is the atomic number. | |
| Speed of electron in hydrogen like atoms | v_n=Z/n v_0 Here, Z is the atomic number, n is the orbit and v0 is a constant whose value is 2.19×106m/s. | |
| Energy in nth orbit | E_n=E_1⋅Z^2/n^2 Here, En is energy of the nth orbit, E1 is the energy of the 1st orbit and its value is -13.6 eV, Z is the atomic number and n is the number orbit | |
| Wavelength corresponding to spectral lines | 1/λ=R[1/(n_1^2 )-1/(n_2^2 )]
 |
|
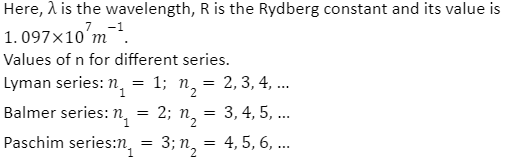| Minimum wavelength for x rays | λ_min=hc/(eV_0 ) Or λ_min=12400/V_0 ×10^(-10) m here, min is the minimum wavelength, h is the plank’s constant, c is the speed of light, e is the charge of an electron and V0 is the accelerating voltage. | |
| Radius of nucleus | R=R_0 A^(1/3) Here, R is the radius of the atom, R_0 is a constant whose value is 1.1×10^(-15)m, A is the mass number of the atom. | |
| Number of nuclei during a radioactive decay | N=N_0 e^(-λt)here, N is the number of nuclei at time t, N_0 is the initial number of nucleus and λ is the decay constant. | |
| Half-life of a radioactive sample | T_(1/2)=0.693/λ Here, T1/2 is the half-life period and λ is the decay constant. | |
| Average life | T_av=T_(1/2)/0.693here, T_avis the average life and T1/2 is the half- life period. | |
JEE Physics Important Formulas Part 2
In this article we will go through physics quick formula revision for JEE 2022. Find the important formulas of Centre of mass, Circular motion and De Broglie wavelength.
