Letter and Symbol Series are a set of letters, numbers, or both that are structured in a manner that each term in the series follows a set of rules. These principles can be based on mathematical processes, alphabetical order, or other factors.
A letter series is a logical grouping of letters from the English alphabet in a predetermined sequence. A series of letters, groups of letters, or combinations of letters and numbers are given in this section. Term is the name given to each group or single element. The series’ terms follow a specific pattern. Candidates must recognise this pattern and locate the missing term(s) in the presented series that will satisfy it.
Types of Letter Series
Letter series can be divided into three categories. The list is as follows:
Alphabet Series
In this type, the letters of the English alphabet are placed in a certain pattern, such as in reverse order, skipping letters, or alphabetical order.
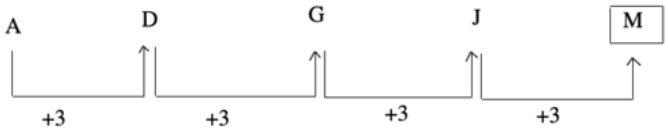
Example: Find the next term in the series.
A, D, G, J, ?
Ans. The pattern of the series is given below
Every third letter of the alphabet is the following term of the series (according to position). As a result, M is the missing term.
Continuous Pattern Series
A succession of small/capital letters are supplied in this kind that follow a specific pattern. However, the sequence is lacking a few letters. Candidates must discover the letters that should replace the blank spaces or question marks in the series, which follows a specified pattern.
Example: _cb_ca_bacb_ca_bac_d
Sol. Pattern of the series is as shown below
acbd / cadb / acbd / cadb / acbd => addddb
The sequence is made of repetition of two blocks ‘acbd’ and ‘cadb’ alternatively. Hence, the missing letters are addddb.
Mixed Series
The series primarily based on a mix of letters and numerals in this case. Each term in the series follows a pattern based on the alphabetical location of the letters or the numerical correlation of the numbers.
Example: What comes in place of question mark (?) in the series given below?
P3C, R5F, T8I, V12L, ?
Sol. Pattern of series is shown below
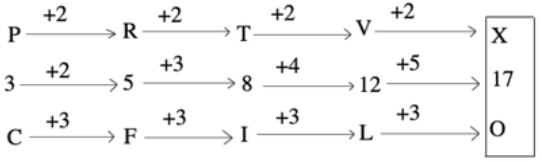
As a result, X17O is the missing word.
Questions on Letter and Symbol Series
Q1. In the alpha numeric series, what should replace the question mark (?)?
A2I, E4J, I8K, O16L, ?
Ans. The series’ pattern is seen below.
The first letter of each phrase is a series of consecutive vowels, the numbers are moved as 2, 22, 23, 24, 25, and the third letter of each term is successively growing. As a result, U32M is the missing word.
Q2. In the alpha numeric series, what should replace the question mark (?)?
9Z-20, 11A-18, 13Y-16, 15B-14, ?
Ans. The pattern of the series is shown below:
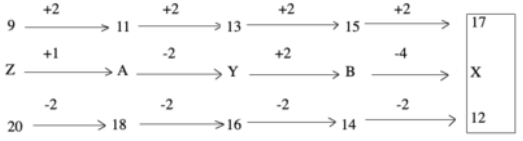
As, a result the missing term is 17X12.
Q3. Look carefully at the sequence of symbols to find the missing pattern.
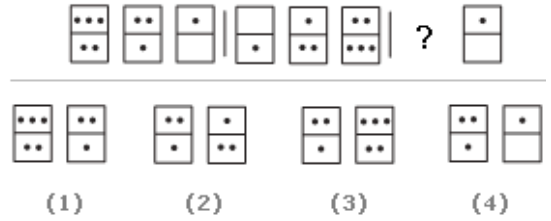
Ans. In all these segments, the count of dots in the bottom box of the previous set equals the count of dots in the top box of the current set. This eliminates options (2), (3) and (4). Thus, the correct option is (1).
Tricks to solve Letter and Symbol Series
Understanding the alphabetical series is the first trick. From A to Z, there are 26 letters in English. As a result, you must order the letters by assigning a value to each letter, such as 1 for A, 2 for B, etc., up to 26 for Z.
There will be a mixture of numbers, letters, and symbols in some questions. In such instances, you must carefully understand the question and respond.
In letter and symbol series, questions focused on consonants and vowels are popular. People regularly inquire about letter positions. So pay close attention to the letter locations.
The question will have certain letter arrangements, and you must respond with the same pattern for each arrangement.
A succession of numbers will be supplied, with some blanks in between, in a number series. You must grasp the supplied series in order to fill in the blanks.
Conclusion
Letter Succession questions are made up of a series of little letters that are arranged in a precise manner. Some letters, however, are missing from the set. The pupils must figure out which letter in the series is missing. As an alternative, the missing letters are presented in the correct order. Here in this article we have tried to cover all the topics related to letter and symbol series, the tips and tricks to solve the letter and symbol series questions.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out





