The total amount of n terms inside a sequence can only be calculated if we know which type of series it is. When calculating the sum of n terms, we usually consider arithmetic progression.

The arithmetic progression equation is used to obtain the total amount of natural numbers formula, where the basic distinction between both the preceding as well as succeeding numbers is 1. Natural numbers, also known as counting numbers, range from 1 to infinity and include the numbers 7,6,5,4,3,2,1, and so on. Let’s look at the total amount of natural digits, how the method is deduced, and how to focus on solving a few illustrations.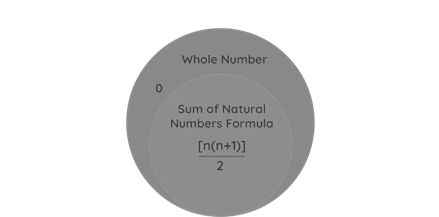

To discover 1 + 2 + 3 + 4 +…. up to n terms, use the total amount of n natural figures formula. This is organized in arithmetic order. As a result, in the arithmetic progression, we use the formula for the sum of n terms to derive the equation for the total amount of natural numbers.

Formula for Sum of Natural Numbers:1n = [n(n+1)]/2, in which n is a natural number.

Total of n Natural Figures Definition

The Sum of n natural numbers is a type of arithmetic progression in which the total amount of n terms is arranged in a series with the first phrase being 1, n is the number of terms, and the nth term being 1. [n(n+1)]/2 represents the total amount of n natural numbers. Natural numbers are those that begin with 1 and end with infinity. Except for the number 0, natural numbers contain only whole numbers.

Solved Examples

Instance 1: To find the first 20 term’s sum if AP’s first term=67 with the common difference = -13

Answer: Now, a = 67 and d= -13

Sn = n/2[2a+(n-1) d]

S20 =20/2[2×67+(20-1) (-13)]

S20= 10[134 – 247]

S20 = -1130

So, the summation of the initial 20 terms is -1130.

Example 2: An AP’s total n and (n-1) terms are 441 and 356, respectively. Find the AP’s common difference if the 1st term of an AP is 13 as well as if the common difference has been equivalenting to the number of terms.

Answer: The totality of n term Sn = 441

Similarly, Sn-1= 356

a = 13

d= n

For an AP, Sn = (n/2) [2a+(n-1) d]

To put n = n-1 in above calculation,

l is the term which is in last. It is likewise represented by an. The consequence got is:

Sn -Sn-1 = an

Thus, 441-356 = an

an = 85 = 13+(n-1) d

In the meantime, d=n,

n(n-1) = 72

⇒n2 – n – 72= 0

Resolving by factorization technique,

n2-9n+8n-72 = 0

(n-9) (n+8) =0

So, n= 9 or -8

Since number of terms could not be -ve,

n= d = 9