In a game of billiards, balls collide with each other at a certain speed. This action of two bodies coming in contact with each other due to an external force is called a collision. But what happens after a collision? Why does the momentum of one ball decrease while that of the other ball increases? Why do they move in opposite directions after collision? What is the velocity of each of the balls before and after the collision? Let us discuss the meaning and importance of the velocity of colliding bodies.
Collision
When two masses interact with each other for a short time, the energy of the colliding masses and momentum change. This event is called a collision.
Collision Types
Here are the different types of collisions:
- Elastic collision
A collision between two bodies, during which the total kinetic energy of the colliding bodies remains constant, is known as an elastic collision. In the case of perfectly elastic collision, kinetic energy does not get converted into other forms of energy like heat or sound.
- Inelastic collision
An inelastic collision refers to the collision in which a portion of the kinetic energy of the colliding particles converts into a new form of energy.
Collision in One Dimension
In this collision, both the initial and final velocities of the masses lie in one line after the collision of masses. Let’s look at the colliding bodies’ velocity in a single dimension.
1.Elastic One-Dimensional Collision
Elastic collisions take place only with microscopic particles like electrons, protons or neutrons. In such collisions, the momentum and kinetic energy are conserved.
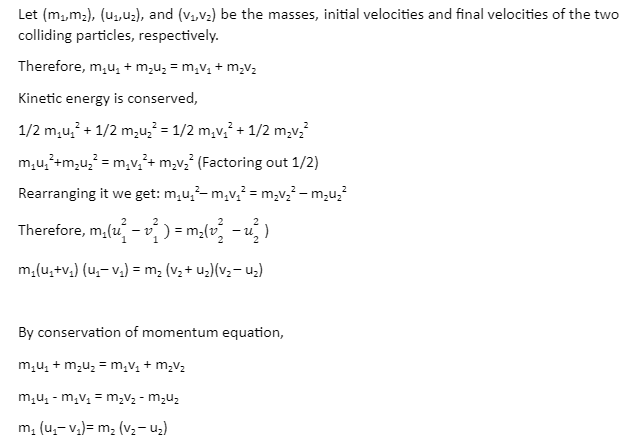

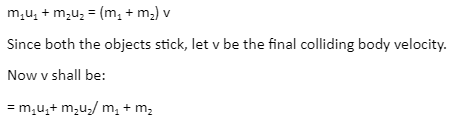
2.Inelastic One-Dimensional Collision
In this collision, the momentum is conserved and kinetic energy changes into different forms of energy. The law of conservation equation for this type of collision is,
Colliding Bodies Velocity Meaning
If the bodies have equal mass, then after the collision, they interchange their velocities with each other. Therefore, v1 = u2 and v2 = u1. The same masses will be v1 = 0 and v2 = u1.
If the second mass is at rest when the first mass collides with it, then after the collision, the first mass comes to rest, and the second mass moves with a speed equal to the first mass.
By any chance if m1 < m2 then, v1 = -u1 and v2 = 0
This means that the lighter body will bombard its velocity, but there will be no momentum in the heavier mass. However, if m1 > m2, then v1 = u1 and v2 = 2u1. The condition of the colliding bodies’ velocity and its importance can, thus, be explained.
Conclusion
We can conclude that, in an elastic collision, there will be no change in kinetic energy. In the inelastic collision, the kinetic energy changes to different forms of energy, but the momentum is conserved. Thus, we can understand the importance of colliding bodies’ velocity in determining whether the collision type is elastic or inelastic. This also proves that the total energy is always constant or conserved in the universe.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out




