An accident usually involves colliding two bodies while we can see the world because photons, the particles of light, collide with objects and then come back to our eyes. In physics, a collision can be classified into different types of collisions based on the total energy of the system and the change in direction. A collision involves two essential things: a change in the velocity of the bodies colliding and a change in the direction of the bodies colliding.
Elastic Collision
Two important quantities dictate if the collision is elastic or not—the momentum of the system and the total kinetic energy of the system. For an elastic collision, the condition is that the system’s total momentum shall be unchanged before and after the collision. At the same time, the total kinetic energy of the system shall also be unaltered.
Consider two bodies, A and B, with masses ma and mb. Let us assume that the two bodies travel in a single direction but with distinct velocities. Let the two bodies be initially travelling at velocities ua and ub. After a while, these bodies collide with each other. Let the bodies exit the collision with velocities va and vb. The momentum of any body can be defined as the product of the body’s mass and velocity.
For an elastic collision, the relation between the total momentum of the two bodies before and after collision can be given as;
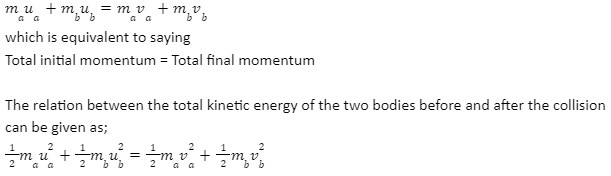
which is equivalent to saying
Total initial kinetic energy = Total final kinetic energy
Inelastic Collision
The law of conservation of momentum, which is a result of Newton’s third law, forbids the total momentum of an isolated system from changing. A collision is called an inelastic collision if the momentum is conserved between two bodies after they collide, but the total kinetic energy of the system changes.
It is a universal truth that energy can neither be created nor be destroyed. But it can be transformed from one form to another. Similarly, when two bodies are in an inelastic collision, the total kinetic energy of the system after the collision is reduced compared to the total kinetic energy of the system before the collision. The difference in energy usually gets transformed to either heat, sound or might even manifest itself as material deformation.
Consider two bodies, A and B, with masses ma and mb. Let us assume that the two bodies are travelling in a single direction but with distinct velocities. Let the two bodies be initially travelling at velocities ua,and ub. After a period of time, these bodies collide with each other. Let the bodies exit the collision with velocities va and vb. The momentum of any body can be defined as the product of the body’s mass and velocity.
For an inelastic collision, then the relation between the total momentum of the two bodies before and after collision can be given as;

which is equivalent to saying
Total initial kinetic energy > Total final kinetic energy, this is because energy is lost in the form of heat, sound, deformation or other forms.
Collision in one dimension and two dimension
Collisions can also be classified based on the number of dimensions involved in measuring the collision. When a collision occurs such that all the variables that are involved in the collision can be measured in a single dimension, it is called a collision in one dimension. In simple words, when the initial and final velocities of the masses that are colliding both lie on one it is called a collision in one dimension.
When two bodies collide such that they travel in different directions to each other after the collision but the trajectories they follow form an angle between them not equal to zero or 180 degrees, then the collision has to be measured in two dimensions. That is the velocities of the two bodies before and after collisions will have two components. Such a collision is called a two dimensional collision since there is directional vector and velocity both involved.
Conclusion
For an elastic collision, the condition is that the total momentum of the system shall be unchanged before and after the collision. At the same time the total kinetic energy of the system shall also be unaltered. A collision is called an inelastic collision if the momentum is conserved between two bodies after they collide but the total kinetic energy of the system changes.
When a collision occurs such that all the variables that are involved in the collision can be measured in a single dimension, it is called a collision in one dimension. In simple words, when the initial and final velocities of the masses that are colliding both lie on one it is called a collision in one dimension. When two bodies collide such that they travel in different directions to each other after the collision but the trajectories they follow form an angle between them not equal to zero or 180 degrees, then the collision is called a two dimensional collision.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



