Projectiles

Introduction:

What is Projectile Motion? As the name suggests, projectile motion is the movement of an object that is launched into the air. Given the initial velocity and angle of projection, it is a problem that has been studied since ancient times. The solution is not obvious and can be approached in various ways. Galileo was one of the first people to have ever described projectile motion. This article will go through a basic introduction of Projectile Motion, it’s important terms and definitions, projectile motion equations, applications, and formulae.

Body: 

Gravity is the most important force at work when a projectile is involved. Other forces are also at play, but they have a negligible impact compared to gravity. A projectile’s trajectory is the path it takes as it travels through space. A baseball hit by a bat or thrown by a player is an example of a projectile.

Parabolic Projectile Motion 

Consider the following scenario: a ball is projected at some angle with the horizontal x-axis with an initial velocity of u.

The point of projection is considered as origin; the projection angle is θ; the distance travelled by the projectile along the x-axis is called Horizontal Range, often known as simply Range. The time it takes for a particle to travel from the point of origin to the surface is called flight time.

To determine various parameters associated with projectile motion, it is possible to employ differential equations of motion:

v = u  + g t             (where g represents vector quantity)

s  = u t + 12g t²

v² = u² + 2g.s        ( g.s  represents dot product of the two vectors)

( For the sake of simplicity let ‘g’ represent the magnitude of g or |g|)

Here,

u is the initial velocity 

g is the acceleration due to gravity

t is the time 

s is the displacement 

v is the final velocity 

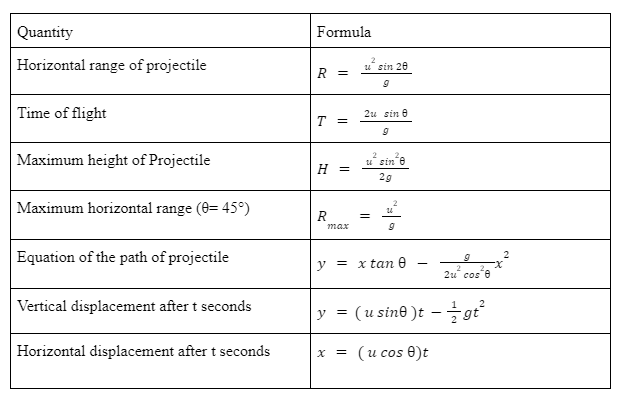
Total time of flight

In the vertical direction, the resultant displacement (s) equals zero. Therefore, the following equation calculates the time of flight formula.

Total time of flight(T) = 2using

Horizontal Range 

The horizontal range is equal to the horizontal component of velocity multiplied by the total flight time (T)

R= u cosθ 2u sin/g

Therefore, in a projectile motion, the horizontal range is given by (R):

Horizontal range(R) = u²sin2g 

Projectile’s Maximum Height

After establishing what a projectile is and what projectile motion is, we should determine the maximum elevation. The highest vertical position along the object’s trajectory corresponds to the maximum height reached. The projectile’s horizontal displacement is referred to as the projectile’s range in this context. The beginning velocity of the object affects the range of the projectile.

u denotes the starting velocity, g represents the acceleration due to gravity, and H is the maximum height in metres, where θ indicates the angle of the initial velocity with the horizontal plane.

The formula for calculating the Projectile’s Maximum Height is as follows:

H = u²sin² θ 2g

Important Points Related to  Projectile Motion

• At the highest point, linear momentum is m(u cos θ), and the kinetic energy is  12m(u cosθ)2.

• The projectile has a parabolic path.

• At the starting point, Kinetic energy= 12mu2

• At the starting point, Linear momentum= mu

Important Formula Related to Projectile