The symbol A-1 denotes the inverse of a matrix. It is only when the whole matrix is A. Many formulas are there that will help you calculate the matrix inverse. But before getting the inverse of a matrix, we must know about the points, i.e., adjacent and determinants. The matrix inverse is the type that will give completely different results from the first matrix. One finds the inverse of a matrix to get about the answers for the linear equations and many more. Here we provide you with the inverse of a matrix note that will help you know about the matrices.
What does the term inverse of a matrix stand for?
The matrix inverse can be calculated with different formulas. These different formulas will help you determine the inverse of a matrix. For getting the inverse of a matrix, there is the presence of prescribed formula. And while calculating the matrix inverse, you have to use that formula in a specified manner.
- Firstly, we have to see the adjoint and determinant of the matrix.
- Once we find these both, we have to move to the next step. We have to divide the adjoint of a matrix with its determinants.
- And lastly, we have to divide the adjoint by the determinants to get the inverse of a matrix.
NOTE: The formula for the A matrix is adj (A)/ |A|; |A| is not equal to zero. We have to find the transpose of the matrix to solve the inverse of a matrix.
The inverse of matrix problems
Here the inverse of a matrix notes will discuss the inversion of a matrix using a specific formula. We provide you with the things that will help you know when you have to do the inverse of a matrix. Many simple things will help you determine these. Such main things are given below:
- When the determinant of a matrix is equal to zero, you can do the inverse of a matrix.
- Pay due consideration when the matrix is not equal to zero. In such a case, the matrix is degenerative or singular.
Examples: Here, we look at the inverse of matrix problems.
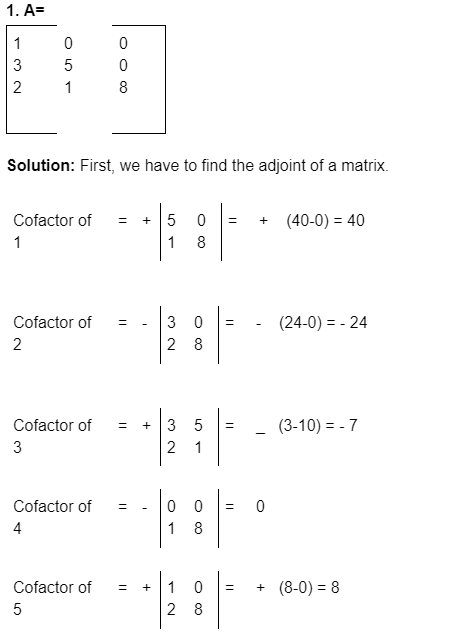



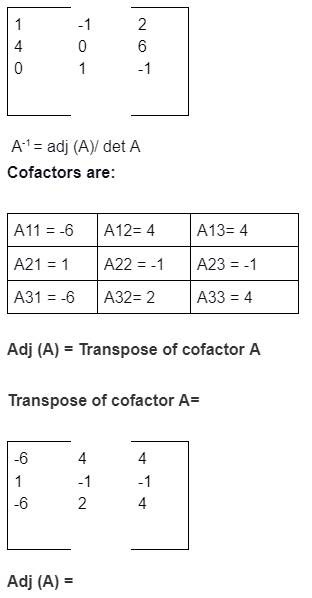

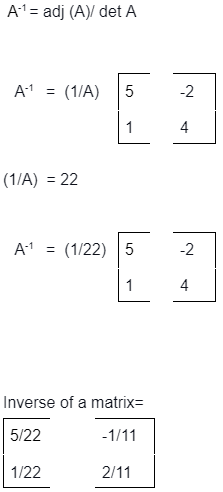
Conclusion
From here, we can see that there are many formulas to determine the matrix’s inverse. The inverse of a matrix is different from the existing matrix. The whole set of the matrix gets displaced compared to the earlier matrix. The inverse of a matrix also uses the multiplication of things which completes the whole set of matrices. To get the inverse of a matrix, we have to perform the different operations, which as a result, will make the total inverse of a matrix. Such operations are row operations or column operations for the inverse of matrix problems. To calculate the inverse, it is a must that you follow each step of the operations carefully.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



