The uncertainty principle, also known as the Heisenberg uncertainty principle or the indeterminacy principle, is a statement made by German physicist Werner Heisenberg in 1927. It states that an object’s position and velocity cannot be measured precisely at the same time, even in theory. In reality, the concepts of precise position and exact velocity have no application in nature.
This principle is not obvious from everyday life. Because the uncertainties suggested by this concept for common objects are sufficiently small to be noticed, it is simple to measure both the position and velocity of, say, a car. The full rule states that the product of location and velocity uncertainties must be equal to or larger than a minuscule physical quantity, or constant (h/4π), where h is Planck’s constant, or approximately 6.6×10-34J.s). Only for atoms and subatomic particles with extremely small masses can the product of uncertainty become important.
Heisenberg uncertainty principle
It is difficult to correctly measure or calculate an object’s position and momentum, according to Heisenberg’s uncertainty principle. This principle is based on matter’s dual nature of wave and particle. Although Heisenberg’s uncertainty principle can be ignored in the macroscopic world (uncertainties in the position and velocity of objects with relatively large masses are minimal), it is extremely important in the quantum world. Since atoms and subatomic particles have such little masses, any improvement in location accuracy will be matched by a rise in the uncertainty associated with their velocities.
Heisenberg’s uncertainty principle is a fundamental idea in quantum mechanics that explains why it is impossible to measure more than one quantum variable at the same time. Another implication of the uncertainty principle is that measuring the energy of a system precisely in a finite length of time is difficult.
Formula and Application of Heisenberg uncertainty principle
In this theory, Δxrepresents a measurement mistake in location, whereas Δprepresents a momentum measurement error. As a result of this principle, we can write:
Δx x Δp≥h/4π
As we know momentum is p=mv, Heisenberg’s uncertainty principle formula can be expressed as:
Δx x Δmv≥h/4π
The Heisenberg principle only applies to dual-natured microscopic particles, not to a macroscopic particle with a very minor wave nature.
Derivation of Heisenberg uncertainty principle
Understanding that every time a measurement is to be conducted, an operator acts on the state vector representing the quantum system is the first theoretical milestone. The operator for position is (where the hat denotes that it’s an operator):
x^=x
When you multiply the state of a quantum system by x, you get its position. The operator for momentum is as follows:
ρ^=-ñ*∂/∂x
This is where the debate over incompatible observables comes into the picture. You can’t observe both with certainty if the operators associated with the observables have a non-zero commutator. For two operators, the commutator is defined as follows:
[A^,B^]=A^B^-B^A^
The generalised uncertainty principle formula supports this commutator of the two operators corresponding to the observables we’re trying to measure. Let’s look at how to find the commutator for position and momentum:
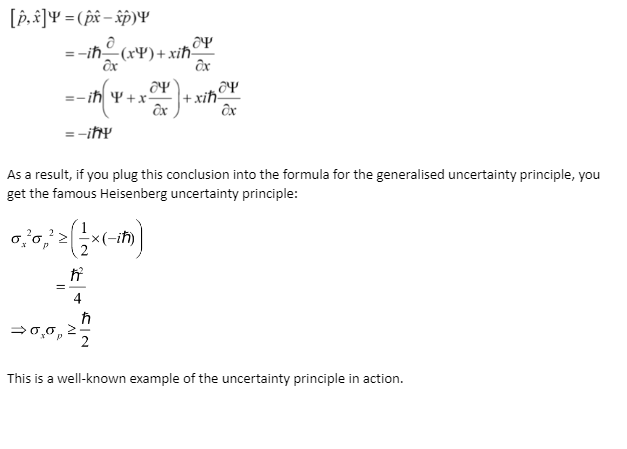
Heisenberg Uncertainty Principle Equations
Heisenberg’s uncertainty principle is a mathematical statement that accurately reflects the nature of quantum systems. As a result, we frequently analyse two common uncertainty principle equations. They’re as follows:
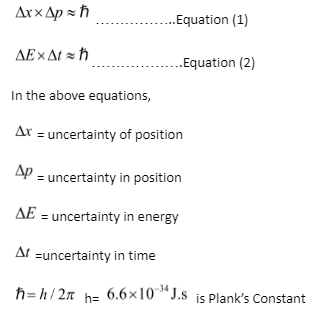
Explanation of Heisenberg Uncertainty Principle using an example
The mass/ momentum and wave characteristics of electromagnetic radiations and tiny matter waves are both present. Macroscopic matter waves’ position and velocity/momentum can be correctly calculated at the same time. A moving car’s location and speed, for example, can be determined simultaneously with minimal error. However, it will not be feasible to fix the particle’s position and detect its velocity/momentum at the same time in minuscule particles.
The mass of an electron in an atom is9.91×10-31kg . Such tiny particles are invisible to naked eyes. The electron may be illuminated by a bright light colliding with it. The position of the electron can be identified and measured with the help of illumination. While assisting in identification, the collision of the bright light source increases the momentum of the electron, causing it to travel away from its initial position. As a result, the particle’s velocity/momentum would have altered from its original value when the position was fixed.
As a result, while the positioning is precise, a mistake in the measurement of velocity or momentum arises. The precise measurement of momentum will affect the position in the same way.
As a result, only precise measurements of position or momentum may be made at any one time.
If you measure both of them at the same time, you’ll have an error in both position and momentum. Heisenberg measured the inaccuracy in simultaneous measurements of location and momentum.
Conclusion
The Heisenberg Uncertainty Principle is among the most interesting and important consequences of quantum physics’ statistical character. The most famous realisation of the uncertainty principle asserts that the position and momentum of a quantum system cannot be measured with absolute certainty. This is the most widely accepted realisation in popular science. The uncertainty principle, on the other hand, is significantly broader. Inequality is another feature of the full uncertainty concept.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



