Gravitational Field Intensity and Gravitational Potential

The form of energy that an object has due to its position in a gravitational field is known as gravitational potential energy. The most typical example of gravitational potential energy is an object at the earth’s surface with a constant gravitational acceleration of 9.8m/s. The gravitational potential energy zero can be chosen at any point (like the coordinate system zero). The potential energy at a height h above the threshold equals the effort necessary to lift the object to that height with no relative change in kinetic energy.

Relation between gravitational field intensity and gravitational potential 

Gravitational field intensity = gradient of gravitational potential = dV/dr 

The gravitational potential gradient dV/dr can be defined as the rate of change of gravitational potential with distance in the field. This is equal to gravitational field intensity at that point. 

U = gravitational potential energy = -GMmr

Gravitational potential V = UM=-Gmr 

Gravitational field intensity I=dVdr=Gmr2

The expression of gravitational potential energy at a point in a gravitational field 

Let M be the mass of earth and P be the point at a distance of ‘r’ where (r > R; it is the distance of the body from the centre of the earth) at which gravitational potential energy can be calculated. Let A be the point at a distance of ‘x’ from the centre of the Earth O. Let us consider a mass ‘m’ at A. The force acting on the unit mass is given by

F=GMmx2

Let the mass move from A to B without acceleration through a minimal distance dx. The work done is given by: 

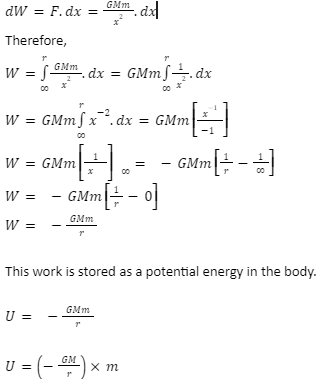
Thus, the gravitational potential energy = gravitational potential at a point x mass of the body at that point, where G is universal gravitation constant and M is the mass of the earth and r is the distance of the body from the centre of the earth. 

Characteristics of gravitational potential energy

• The gravitational potential energy of an object decreases in proportion to its descent from one point to another inside a gravitational field

• The work done in elevating an object through a height h is the product mgh near the earth’s surface. Therefore, U = mgh.

• The gravitational potential energy U of a system of mass m1 and m2 at a distance r using gravitational constant G is U = −Gm1m2/r 

• The gravitational potential energy is influenced by its mass, height in relation to a reference point, and the strength of the gravitational field it is in

• At infinity, the gravitational field intensity is 0, and it gradually decreases as the test mass approaches the attracting body. As a result, the body moves from a higher to a lower potential, resulting in a negative gravitational potential

• It can be compared to the electrical potential. As the current flows from a higher to a lower electrical potential, mass moves from a higher to a lower electrical potential

• Equipotential surfaces are those that have the same value at all places on their surface

• It reaches its peak at infinity zero

• It is a scalar quantity

Gravitational potential energy formula 

The equation of gravitational potential energy is 

U = m⋅g⋅h

where, 

• m is the mass in kilogram

• g is the acceleration due to gravity 

• h is the height above the ground in metres 

Conclusion 

The strength of a gravitational field delivered to a unit test mass is called gravitational field intensity. The intensity of a gravitational force is determined by the source mass and the distance between the source mass and the unit test mass. An item at the earth’s surface with a constant gravitational acceleration of 9.8 m/s is the most common example of gravitational potential energy. The gravitational potential energy zero can be chosen anywhere (just like the coordinate system zero), and the potential energy at a height h above the point is equal to the effort required to lift the object to that height with no change in kinetic energy.