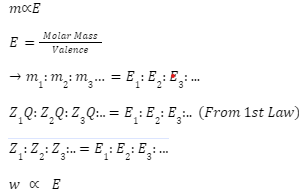Faraday’s laws of electrolysis

Two quantitative rules used in chemistry to quantify the magnitudes of electrolytic effects, originally described by the English scientist Michael Faraday in 1833 and published in his journal Faraday’s laws of electrolysis in 1834. Following the laws of chemistry, it is stated that the amount of chemical change produced by the current at an electrode-electrolyte boundary is directly proportional to the amount of electricity used and that the amounts of chemical change produced by the same quantity of electricity in different substances are directly proportional to their equivalent weights. 

What is the definition of an Electrode? 

An electrode may be described as the point at which current enters or exits the electrolyte or circuit, depending on the definition used. When current departs an electrode, it is referred to as the cathode, and when current enters an electrode, it is referred to as the anode. 

Electric current flows via electrodes, which serve as the primary component of electrochemical cells. An electrode must be a good conductor of electricity to function properly. Although there are inert electrodes that do not participate in the reaction, they are rare. The electrode might be made of gold, platinum, carbon, graphite, metal, or any combination of these materials. During oxidation-reduction processes in the cells, the electrode serves as a surface for the reactions to occur. 

What is an Electrolytic Cell, and how does it work? 

Electricity is converted into chemical potential energy by electrolytic cells, which are electrochemical cells that convert electrical energy. Because we have already examined electrolysis, it is reasonable to assume that electrolytic cells are involved in the electrolysis process. Secondary cells, also known as electrolytic cells, are rechargeable, which implies that in these cells, reversible chemical processes take place. The anode of these cells is always positive, but the cathode is always negative in these cells. 

First law

According to Michael Faraday, the mass of elements deposited at an electrode is exactly proportional to the charge in ampere seconds or coulombs applied to the electrode. 

m∝Q

mQ=Z

• m denotes the mass of a material (in grams) that has been deposited or released at an electrode. 

• Q is the quantity of charge (measured in coulombs) or energy that has flowed through the device. 

When the proportionality in equation (1) is removed, the result is – 

 m=ZQ

In this case, Z represents the proportionality constant. The unit of measurement is grams per coulomb (g/C). It is referred to as the electrochemical equivalent in certain circles. Z is the mass of a material deposited at electrodes during electrolysis while avoiding the application of a single coulomb of electricity. 

The electrochemical equivalent (e.c.e.) of the substance is referred to as the constant of proportionality in this context. In this case, the equivalent charge equivalent might be defined as the mass of the material deposited or freed per unit of charge. 

Second Law

Faraday discovered that when the same amount of electric current is passed through different electrolytes/elements connected in series, the mass of the substance liberated/deposited at the electrodes in g is directly proportional to the chemical equivalent/equivalent weight of the electrolytes/elements in question. According to the calculations, this is equal to the molar mass (M) divided by the valence (v). 

In where w denotes the mass of the material  

E = the substance’s equivalent weight in kilograms.  

It is also possible to phrase it as – w1/w2=E1/E2

When considering a substance’s equivalent weight or chemical equivalent, it may be defined as the ratio of the substance’s atomic weight to its valency. 

Equivalent weight=Atomic weight/Valency 

Further explanation of Faraday’s Second Law of Electrolysis may be provided by the following example – 

Let’s consider three distinct chemical reactions occurring in three different electrolytic cells that are linked in series with one another. Assume that in the first electrolytic cell, sodium ion obtains electrons and undergoes a transformation into sodium. 

Na+ + e-→ Na

In 2nd electrolytic cell following reaction occurs –

 Cu+2+ 2e-→ Cu

In 3rd electrolytic cell following reaction occurs –

Al3++ 3e-→ Al

When y moles of electrons are transferred through three cells, the mass of sodium, aluminum, and copper released is 23y grams, 9y grams, and 31.75y grams, respectively, assuming that the electrons are all positive. 

To reduce one mole of ions, one mole of electrons must be used to accomplish the reduction. As we all know, the charge on one electron is equivalent to the charge on another electron. 

1.6021×10-19

One mole of carbon and one mole of electrons is equivalent to 

6.023×1023

 electrons. As a result, the charge on one mole of electrons equals – 

 (6.023×1023)×(1.6021×10-19C)=96500 C

1 Faraday is the unit of charge equal to 96500 C. 

If we transmit one Faraday of charge through an electrolytic cell, then one gram of the substance’s equivalent weight will be deposited in that cell. As a result, we may write — 

w=(Q / 96500)×E

When we combine the first and second laws, we obtain the following result: 

Z= E / 96500

Chemical Equivalent or Equivalent Weight 

By Faraday’s laws of electrolysis, it is possible to calculate the chemical equivalent or equivalent weight of a material, which is defined as the mass or weight equivalent of the subtenancy that will combine with or displace the unit weight of hydrogen. 

As a result, the chemical equivalent of hydrogen is number one. Given that the valency of a substance is equal to the number of hydrogen atoms that it may replace or combine with, the chemical equivalent of a substance can be defined as the ratio of the atomic weight of the material to the valency of the substance. 

Chemical equivalent= Atomic WeightValency

Conclusion

The amount of chemical that is deposited as a result of electrolysis has so far been determined to be proportional to the amount of electricity that flows through the electrolyte. Electrolysis deposits a mass of chemicals that is not only proportional to the amount of power that goes through the electrolyte but also relies on many other factors. Every material will have its atomic weight, which will be unique. Different substances will have different masses even though they contain the same number of atoms. 

Again, the amount of atoms deposited on the electrodes is dependent on the number of valencies present in the electrodes. A higher value of valency means that for the same amount of power, the number of deposited atoms will be smaller; on the other hand, when the value of valency is lower, a greater number of deposited atoms will be achieved for the same amount of energy. 

The mass of the deposited chemical is directly proportional to the atomic weight of the chemical and inversely proportional to the valency of the chemical when the same amount of power or charge is passed through various electrolytes of the same type. 

Faraday’s second law of electrolysis says that when the same amount of electricity is transmitted through the same number of electrolytes, the mass of the substances deposited is proportionate to the chemical equivalent or equivalent weight of the chemicals deposited in the electrolytes.