JEE Exam » JEE Study Material » Physics » Determining Area Moment of Inertia

Determining Area Moment of Inertia

The different shapes and geometrical figures tend to change physically after rotating around a fixed line in the plane. Thus, the area moment of inertia is observed and evaluated for some of the generic figures.

The tendency of any physical body to resist changes when rotated concerning a specific fixed line in the 3-D plane is the area moment of inertia. This change can be evaluated using the centroid, where the overall impact of forces is zero. Thus, the tendency for different shapes to change physically can be assumed to depend on the centroid. 

Centroid – This is the physical point present on the body, wherein the overall body concentrates its weight and balances itself to cancel out all kinds of forces. The centroid can be calculated depending upon the dimensions of the physical body. The centroid of the physical body is not affected by the size, as it is the balancing point of the whole body. Thus, if we take a knife and place the body on its tip, it is in equilibrium and balances itself without tumbling. The point is assumed to be the centroid of the given physical body. 

Thus, the area moment of inertia can be evaluated for any physical body using the centroid.  

Factors affecting the Moment of Inertia

There can be infinite factors affecting it, but the orientation is significant. If we have two physical bodies of the same area and shape, but the orientation is different, then the moment of inertia would depend on how the body is placed. 

The shape of the physical body is considered to evaluate the area moment of inertia. Now, the centroid changes for different bodies, and so does the area moment. For a body to be rectangular, the other to be circular, the centroid is different, even though we know that the centre is the centroid.

Determining Area Moment of Inertia of body

The area moment of inertia for some of the generic physical bodies is pre-defined based on their bending around the centroid. Hence, there are two principles-

Perpendicular Axis Theorem

The area moment of inertia for any physical body about a fixed line normal to the plane is given as the summation of the area moment of inertia for two axes in the same plane which must be orthogonal and passing through the axis.It is only applicable for 2D objects.

Thus, we can say that area moment of inertia is – IZ=IX+IY

Parallel Axis Theorem

The area moment of inertia is computed from the fixed line, which is parallel to the axis containing the centroid of the physical body. Now, for the overall area moment of inertia for the physical bodies, evaluate the area moment of inertia and add the product of area and square of separation from the fixed line. 

Thus, 

I=Ic+Al2

Where, 

I is the area moment of inertia,

Ic  is the area moment of inertia about the centre of mass. 

A is the area of the physical body, and

l is the separation from the fixed line.

Now, area moment of inertia for basic physical bodies are as follows – 

r1

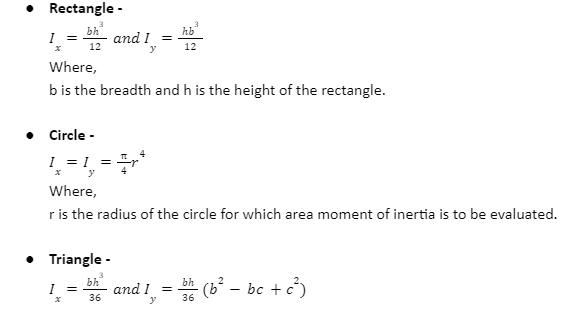
Where, 

  • b is the measurement of the base of the triangle
  • h is the height of the triangle from the base 
  • c is the distance between the top vertex to the nearest base vertex
  • For asymmetrical bodies – 

The area moment of inertia is evaluated using the centroid as usual. Thus, the x and the y coordinates are evaluated. 

First make sections of the body from basic and generic shapes. Now for each section, calculate the area using the dimensions and calculate the centroid as – 

r2

Where,

x and y are the coordinate of the centroid for the respective section made from the whole body. 

Applications

The area moment of inertia is used to compute the different mechanical design and calculation for the resistance of the bending due to external forces or rotation. This can be used in buildings, construction of bridges, and many more civil projects. 

Conclusion

The important points to remember in this concept:  

  1. The area moment of inertia for any physical body depends on the shape and the orientation.
  2. The area moment of inertia is evaluated with respect to the x and the y axis from the fixed line for bending effect.
  3. The different bodies have different centroids, and the area moment of inertia takes into consideration the point of centroid and the origin. 
  4. The composite bodies can be segregated and sectioned into general bodies for simple calculation of area moment of inertia.
faq

Frequently asked questions

Get answers to the most common queries related to the JEE Examination Preparation.

What is the most fundamental thing used to evaluate the area moment of inertia?

Ans. The most fundamental thing used to evaluate the area moment of inertia is the shape and orientation of the phys...Read full

What is the best way to evaluate the area moment of inertia of an object?

Ans. The simplest way of finding the area moment of inertia is the area and the distance of the physical body concer...Read full

Which principle uses the axis passing through the centroid of the physical body.

Ans. The axis passing through the centroid is used in the parallel axis theorem for computing the area moment of ine...Read full

Can the area moment of inertia be the same for two bodies?

Ans. Yes,the area moment of inertia can be the same for two bodies.The area moment of inertia is the same for two bo...Read full