Derivation of Bending Equation

Bending is defined as the distortion of a structure in one of the longitudinal planes owing to a force in applied mechanics. This force is also supposed to be exerted in the direction of one of the structure’s longitudinal planes. We shall derive the bending equation for a beam here. When two of a structure’s dimensions are smaller by a factor of 1/10 or less than the third dimension, the structure is considered to be a beam.

When a transverse load is given to a beam, it deforms. This force acts in a direction perpendicular to the beam’s longitudinal plane. The amount of load applied and the amount of stress experienced by the beam are assumed to remain constant over time.

A beam supported at its ends by clamps is a classic example. The top section of the beam is compressed and the lower part of the beam is extended when a force is applied perpendicular to its longitudinal plane.

The beam is under two types of stress in this case. The first is shear stress, which works in the same direction as the lateral loading. The second type of stress is compression stress in the upper region and tensile stress in the lower portion of the beam. A pair or a moment is formed by compression and tensile stress. This moment is in charge of supplying resistance to the beam sagging and deformation.

What is I in bending equation

I is the moment of inertia that is applied to the bending axis.

Assumption

• It is assumed that the beam is straight. Wooden beams used in the construction of dwellings are an example.

• The cross-sectional area of the beam is believed to be constant. This indicates that a beam’s cross-sectional area should remain constant throughout its length.

• The longitudinal planes of the beam should be symmetrical. This means that the beam’s diameters should all be perpendicular to one another.

• The vector sum of all forces acting on the load should be directed in the symmetry planes. This indicates that the forces’ resulting sum should not be in any direction other than the symmetrical planes’ direction.

• Buckling is thought to be the primary cause of failure.

• For tension and compression, E remains the same. The beam’s Young’s modulus is E.

• After bending, the beam’s cross-sectional area is considered to be the same.

Derivation of beam bending equation

We’ll use the following arrangement to derive the bending equation:

The beam is seen in the first image without any applied pressure. We can see that the beam is not strained. It’s also devoid of any flaws. A, B, C, and D are the four points on the beam. AB = CD is the length. Between the lines AB and CD, the perpendicular distance is y. The beam’s neutral axis is designated as CD. The neutral axis is the point on the beam where there is no tension.

Consider the same beam when it is pressed against anything. The shape of the beam has warped as a result of the load. It has assumed the form of an arc, with the central angle being. The radius of the provided arc is R. It is assumed that the cross-sectional area remains unaltered. The points A’, B’, C’, and D’ denote the positions of the points A, B, C, and D, respectively.

Now, strain is defined as the ratio of a locus on the beam’s change in length to its initial length. As a result, the strain in AB can be written as:

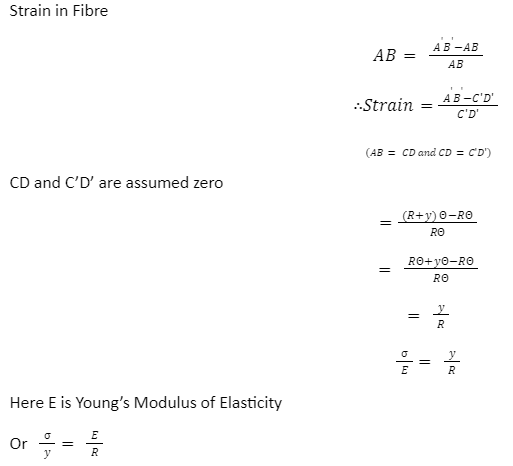
This is the bending equation.

Conclusion

Bending (also known as flexure) is the behaviour of a slender structural element exposed to an external load applied perpendicular to the element’s longitudinal axis in applied mechanics.

The structural element is assumed to have at least one dimension that is a small proportion of the other two, often 1/10 or less. The element is called a beam when its length is significantly greater than its breadth and thickness. A closet rod sagging beneath the weight of clothes on hooks, for example, is an example of a beam that is bending.