We rationalize the denominator to make any calculation on the rational number easy. When we rationalize the denominator in a fraction, we are removing any radical expressions from the denominator, such as square roots and cube roots.

Rationalizing is the process of multiplying a surd by another surd of the same kind to produce a rational number. The rationalization factor is the surd that is utilized to multiply (RF).

Rationalizing the denominator is the process of transferring a root, such as a cube root or a square root, from the bottom to the top of a fraction denominator. In this approach, the fraction is reduced to its simplest form, and the denominator becomes rational.

Simplify and rationalize the Denominator

The following is the procedure for rationalizing the denominator:

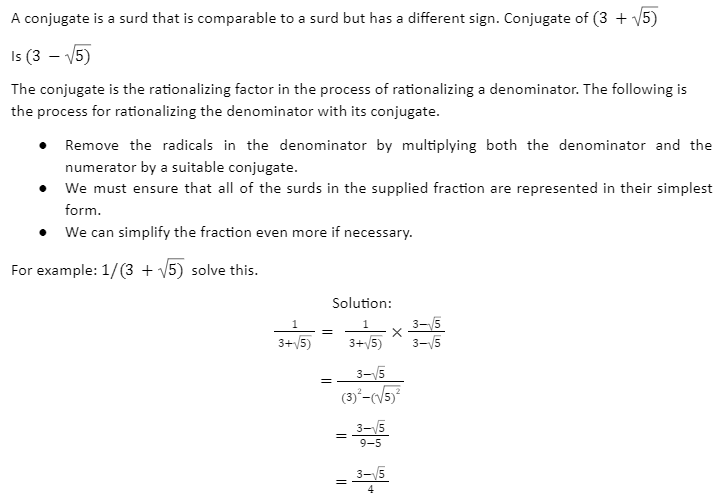
• Multiply the numerator and denominator by a conjugate that removes the radicals from the denominator.
• Verify that all of the surds in the specified fraction are in their simplest form.
• We can simplify the fraction even more if necessary.

Rationalize a denominator with 2 terms?

The steps to rationalize the denominator using two terms are as follows:

• We multiply the numerator and denominator of the fraction with the conjugate of the denominator to rationalize the denominator with two terms.
• We must shift the sign between the two terms to discover the conjugate of two terms.
• All similar phrases should be combined, and the radicals should be simplified.
• If at all possible, reduce the fraction to its simplest form.

How do you rationalize the denominator with a square root

Conjugates are used to rationalize the denominator

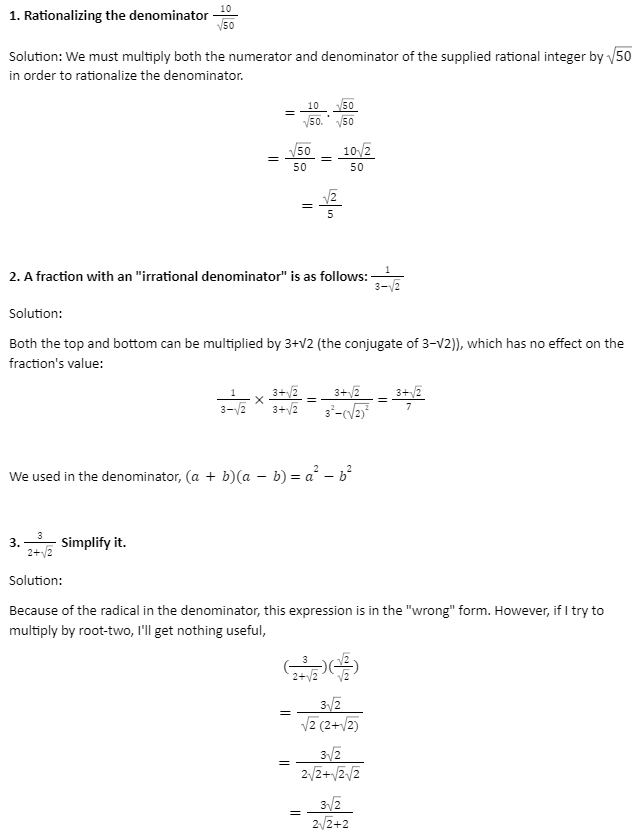
Rationalizing the denominator exam questions

A sample of questions that may appear on the exam are given below.

Conclusion

We study that, Rationalization can be defined as the process of removing a radical or imaginary The denominator of an algebraic fraction is a number. To put it another way, remove the radicals in a fraction until the denominator is only a rational integer.

When you have a fraction with a radical in the denominator, you can eliminate the radical by using a technique called rationalizing a denominator. By removing radicals from the denominators, rationalizing a denominator makes it easier to understand what the quantity really is.