Profile
Settings
Refer your friends
Sign out
A point, often known as the focus, and a line are two components of one definition of a parabola (the directrix). The directrix is not the major focus of attention at this stage in the performance. The parabola is the set of points in that plane which might be the equal distance from the directrix as they may be from the focus. These points are evenly spaced.
Parabola Equation
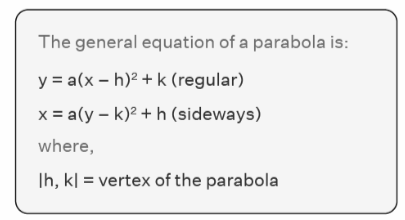
The general equation for a parabola is written as follows: y = a(x-h)̣² + k or x = a(y-k)² +h, where (h,k) represents the vertex of the parabola. y² = 4ax is the equation that is used to describe a parabola that is regular.
Properties of Parabola
The tangent is a line that touches the parabola, and its name comes from the word “tangent.” At the point of contact with the parabola, the equation of a tangent to the parabola is y² = 4ax.
The normal is defined as the line that is drawn perpendicular to the tangent and travels through both the point of contact and the focus of the parabola. The equation of the normal that passes through the point on a parabola is y² = 4ax.
The chord that is drawn to unite the point of contact of the tangents that are drawn from an external point to the parabola is referred to as the chord of contact. The equation of the chord of contact at a point outside the parabola with coordinates (x1, y1) is as follows:
yy1=2x(x+x1).
Pole and Polar
The locus of the points of intersection of the tangents formed at the ends of the chords drawn from this point is referred to as the polar for a point that lies outside the parabola. This point is also referred to as the pole. In the case of a pole with the coordinates.
(x1,y1), and the equation of the polaris is y1=2x(x+x1), which is for the parabola y²=4ax.
The point with coordinates a and 0 is known as the focus of the parabola.
Directrix
The directrix of the parabola is the line that is drawn parallel to the y-axis and passes through the point that is labelled with a negative value and a zero. The directrix of the parabola is oriented along a path that runs in a direction that is perpendicular to the axis.
The distance between two points is referred to as the focal distance.
The focal distance is denoted by the coordinates (x1,y1) on the parabola, measured from the focus. This point’s perpendicular distance from the directrix is likewise equal to the focal distance, hence the two concepts are equivalent.
The latus rectum is the focal chord that is perpendicular to the axis of the parabola and is passing through the focus of the parabola. It is also known as the focal chord of the focus. The length of the latus rectum is taken as LL’ = 4a. The ends of the latus rectum are (a) and (2a), respectively (a, -2a).
Eccentricity of Parabola
The set of points P that define a parabola are those in which the distances from a fixed point F (the focus) in the plane are equal to the distances from a fixed line l (the directrix) in the plane. To put it another way, the distance from a plane’s fixed point bears a constant ratio that is equal to the distance from the plane’s fixed line.
As a result, the eccentricity of the parabola is equal to 1, which may be written as e equals 1.
The standard equation for a parabola is x² = 4ay, and its eccentricity is equal to one.
Parabola Formula
With the assistance of the Parabola Formula, one is able to express the overall shape of the path that a parabolic curve takes in the plane. The following is a list that demonstrates the formulas that can be applied in order to derive the parameters of a parabola.
The value of a will tell you which way the parabola will point when it is plotted.
The vertex is equal to (h,k), where h equals -b/2a and k equals f. (h)
Latus Rectum = 4a
The emphasis is on: (h, k+ (1/4a))
The directrix is as follows: y = k – 1/4a
The Focus of Parabola
If you know the axis of the parabola as well as the vertex of the parabola, you can figure out where the focus of the parabola is located. The vertex of the parabola is located at the origin, and the axis of this particular parabola is the x-axis. A standard form of the parabola equation looks like this: y² = 4ax. As a result, the emphasis of this parabola is on (a, 0).
Conclusion
The general form of the parabolic path in the plane can be represented with the assistance of the Parabola Formula. The formulas that are used to obtain the parameters of a parabola are shown in the following list.
The direction of the parabola is determined by the value of a.The coordinates of the vertex are written as (h,k), where h is equal to -b/2a and k is equal to f. (h)
Latus Rectum = 4a
The stress should be placed on: (h, k+ (1/4a))
The following expression represents the directrix: y = k – 1/4a.
