Ordered pair

Introduction

An ordered pair is made up of two numbers written in a specific sequence. As a result, an ordered pair may be defined as a pair of items that appear in a specific sequence and are contained in brackets. An ordered pair is made up of the x and y coordinates, as well as two values expressed in a certain order between parenthesis.

Ordered Pair Overview

A number written in a specific order is referred to as an ordered pair. The “x” (horizontal) value is first, and the “y” (vertical) value is second, in an ordered pair to display the location on a graph. The ordered pair is also used to locate a location in the co-ordinate system. In Arithmetic, pairs are indicated by the symbol (,) and are usually assumed to be ordered.

Explanation of Ordered Pair

Ordered Pair = (x, y), where x is the abscissa or distance from the x-axis, and y is the y-axis. 

And y = ordinate, which is the distance between a point and the y-axis.

To graph a point, we must place a dot at the ordered pair’s co-ordinates. The x- co-ordinate indicates how many steps are required to reach the x-axis. The y- co-ordinate indicates that we will be moving along the y-axis in a large number of steps.

Example of an Ordered Pair

The ordered pair (2, 5) is a pair of two integers that are strictly in order, with 2 in the first place (abscissa) and 5 in the second position (ordinate). Because (2, 5) is not equal to (5, 2), the ordered pair (2, 5) is not equivalent to (5, 2). (5, 2). As a result, the order of the pieces in a pair is crucial.

Set of Ordered Pairs 

A set of ordered pairs is a pair of components that appear in a specific sequence and are wrapped in brackets. If ‘a’ and ‘b’ are two elements, then (a, b) and (a, b) are the two distinct pairings (b, a). The first element in an ordered pair (a, b) is referred to as the first element, whereas the second element is referred to as the second element.

If A and B are two sets with the properties an A and  B, then the ordered pair of elements is (a, b), with ‘a’ being the first element and ‘b’ being the second member in the ordered pair.

Ordered Pairs’ Characteristics

Ordered Pairs Equality

Two ordered pairs are said to be equal if and only if their initial elements are the same and their second elements are the same.

Consider the following two ordered pairs (a, b): (c, d). If a = c and b = d, they are equivalent, i.e. (a, b) = (c, d).

Co-ordinate Geometry Ordered Pair

The location of a point on the co-ordinate plane with respect to the origin is represented as an ordered pair in co-ordinate geometry. Two perpendicular intersecting lines, one horizontal (x-axis) and the other vertical (y-axis) constitute a coordinate plane (y-axis). The origin is the place where both axis cross. Every point on the co-ordinate plane is given by an ordered pair (x, y), with x as the x- co-ordinate and y as the y- co-ordinate. More distinctions between the components of the ordered pair used in Geometry may be seen here.

Ordered Pairs Graphing

In co-ordinate geometry, we now know the difference between the x- co-ordinate and the y- co-ordinate of an ordered pair. Let’s look at how to graph ordered pairs.

Step 1: Always begin at the origin and work your way horizontally by |x| units to the right if x is positive and to the left if x is negative. Keep going.

Step 2: Move vertically by |y| units up if y is positive and down if y is negative, starting where you stopped in Step 1. Keep going.

Step 3: Mark the spot where you stopped in Step 2 with a dot, and that dot indicates the ordered pair (x, y).

The absolute values of x and y are represented by |x| and |y| in these steps.

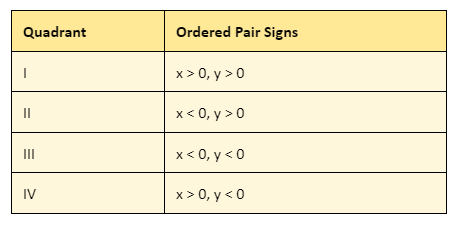
Different Quadrants with Ordered Pairs

The x and y axes split the co-ordinate plane into four pieces, as seen in the diagram above. Each of these four sections is referred to as a quadrant. The signs of x and y in an ordered pair of points (x, y) vary depending on the quadrant, as illustrated in the table below.