Harmonic Mean

Harmonic Mean is one of the measures of average calculation. Harmonic Mean is the reciprocal of the Arithmetic Mean of the reciprocals. Just like Arithmetic Mean, Harmonic Mean is very simple to calculate. The Harmonic mean of given numbers is equal to the number of observations divided by the sum of reciprocal of the given numbers. Let’s discuss in detail the concepts revolving around the topic and derivation formulas.

The harmonic mean of numbers:

The harmonic mean of two given numbers x1 and x2 are equal to the number of observations divided by the sum of reciprocals of the given two numbers.

Mathematically, we can write it as follows:

For two numbers, no. of observations will be equal to 2.

Sum of Reciprocal of given observations/numbers will be = 1/x1+ 1/x2

Putting the values derived above in the formula, we will get:

HM of x1, x2 = No. of observations/ the sum of reciprocal of the given two numbers

HM of x1, x2 = 2 / (1/x1+ 1/x2)

Taking L.C.M., we will get final result as below:

= 2 x1 x2 / x1 + x2

Similarly, Harmonic mean of three numbers x1 , x2 and x3 will be

HM of x1, x2, x3 = 3 / (1/x1+ 1/x2 + 1/x3)

Taking L.C.M., we will get as follows:

= 3 x1 x2x3 / x1 x2+ x2 x3+ x1 x3

The same pattern can be followed further to get the Harmonic Mean of n numbers as well.

Single Harmonic Mean of n ‘positive’ numbers:

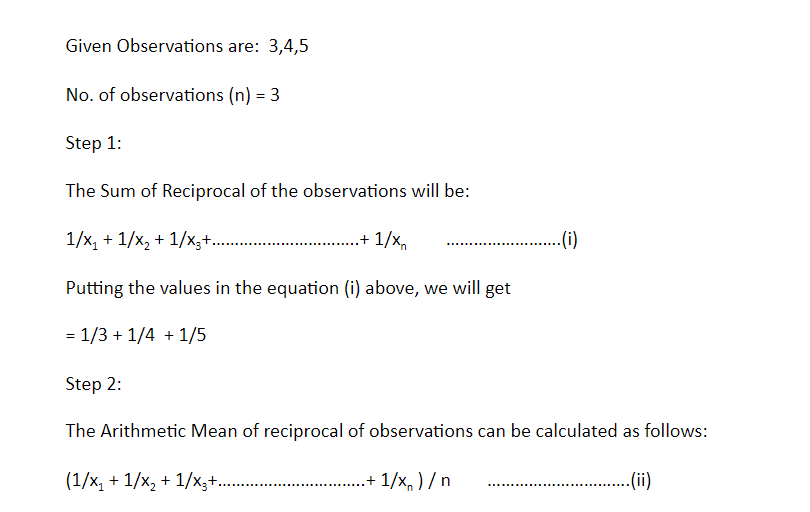
Single Harmonic Mean of n ‘positive’ numbers can be defined as the reciprocal of the Arithmetic Mean of the reciprocal of the observations. Now, let us break the definition of Harmonic Mean stated here to get a deep insight of the meaning of the terms used therein:

Let us assume that we have n number of observations and the observations are x1, x2, x3,…………………xn

The Sum of Reciprocal of the observations will be:

1/x1 + 1/x2 + 1/x3+…………………………..+ 1/xn         …………………….(i)

Now, the Arithmetic Mean of the reciprocal of observations can be calculated as follows:

( 1/x1 + 1/x2 + 1/x3+…………………………..+ 1/xn ) / n        ………………………….(ii)

Now, the reciprocal of Arithmetic Mean of the reciprocal of the observations will be simply the reciprocal of what we just calculated above in step (ii):

n / (1/x1 + 1/x2 + 1/x3+…………………………..+ 1/xn ) ………………………(iii)

The resulting equation (iii) is called the Harmonic Mean.

‘n’ Harmonic Mean between two numbers a and b:

H1 is called one Harmonic Mean between two numbers ‘a’ and ‘b’ if a, H1, b forms a Harmonic Progression (H.P.).

Similarly, H1 and H2 are called two Harmonic Means between two numbers ‘a’ and ‘b’ if a, H1, H2, b forms a Harmonic Progression (H.P.).

Conclusion:

In this article, we discussed the concepts related to Harmonic Mean. First, we saw the definition of Harmonic Mean and then understood all the subsections or parts of the definition to get to the final result i.e. how Harmonic Mean is actually derived using multiple steps. We saw implications of Harmonic Mean further with the help of practical examples. We hope this study material will be helpful for you to get a deep insight into the topic.