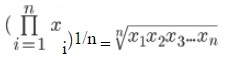Profile
Settings
Refer your friends
Sign out
In mathematics, the geometric means is the mean or the average of a set of numbers that indicates the central tendency or typical value in the set of numbers by using the product of their values. Compared to the arithmetic mean that uses the sum of all values, the geometric means is calculated by using the product of the values.
The geometric means of a series that has n numbers is also defined as the nth root of the product of all the n numbers. If there is a set of numbers x1, x2, x3,… xn, the geomertric means is defined as
That is, for any two numbers, the geometric means can be found by multiplying the two products and then taking their square root. For three numbers, the process will be similar, except this time, we will be taking the product of the three numbers and then taking its cube root. Geometric means can be found using only positive numbers.
Importance of Geometric Means
Geometric means has its uses in a lot of mathematical concepts. The geometric means is frequently used when the values in a set of numbers are supposed to be multiplied together or are exponential in nature. An example of this is perhaps the human population, the interest rates of a loan or a financial investment. The geometric means is also used for benchmarking purposes, a popular usage is in calculating the means of the speedup ratio.
Geometric Means in Terms of Geometry
In terms of geometry, if we need to find the geometric means of two numbers a and b, then it is equal to the length of one side of a square whose area is equal to the area of a rectangle with sides a and b. That means if we have a rectangle of sides with length a and b then the area of the rectangle should be ab. On the other hand, we create a square such that its area is
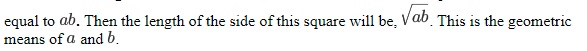
In case we have three numbers a,b and c, then the geometric means of these numbers is equal to the length of one side of the cube whose volume is equal to the volume of the cuboid that has sides of lengths a,b and c. Therefore, if the cuboid has sides of length a,b and c, then its volume is given as abc. If the cube has a volume of abc then the length of one edge will be
Relation between the Pythagorean means
We will also cover the relation of geometric means with Pythagorean means. For a non-zero positive series of numbers, the arithmetic mean, geometric means, and the harmonic mean form the Pythagorean means. For a set of numbers that has only non-zero positive values with at least one unequal value, the relation between the harmonic mean, arithmetic mean, and geometric means is given as
H.M<G.M<A.M
Relation between Arithmetic Mean and Geometric Means
Amongst the three means, the relationship between arithmetic and geometric means is of greater importance as it can be used to find quadratic equations and their solutions.
Consider the arithmetic mean of two numbers, a and b, to be A and the geometric means to be G. Then,
Inserting Geometric Means Between Two Numbers a and b
Let us discuss insertion and progression. To form a geometric progression between two
Conclusion
Geometric means share a very close relation with our real-life situations. Be it measuring the human population or the interest rates, geometric means have a very prominent role.
We have taken a look at geometric means along with its relation with arithmetic means and Pythagorean means. This will also help in understanding the ‘how’ factor of applying geometric means across various other fields.
