Beta function

The Beta function, often known as the first kind of Euler’s integrals, is a one-of-a-kind function. The beta function is specified using real-number domains. The symbol “β” is used to denote it. (p,q) represents the beta function, and p and q are both real numbers.

The beta function is important in calculus and analysis because of its close link to the gamma function, which is an extension of the factorial function. Many complicated integrals can be simplified using the beta function.

Beta function formula

The beta function is a mathematical formula for calculating the chance of an event occurring. It’s also used to figure out how likely two events are to happen at the same time. The formula for the beta function is:

As a result, we can conclude that the beta function is symmetric B(x,y)=B(y,x)

Relation with Gamma Function

In the sense that the beta function is a particular instance of the gamma function, the two functions are connected. The beta function is a tool for calculating the lower division a curve. The gamma function is a formula for calculating the value of a series of terms. The factorial of an integer is also calculated using the gamma function.

 We have,

Proof

Recall the gamma function’s definition.

Hence proved

Incomplete beta function

A generalization of the beta function is the incomplete beta function, which is defined as

Bx;a,b=0xta-1(1-t)b-1dt

The incomplete beta function and the complete beta function are the same when x = 1. The two functions have a relationship similar to that of the gamma function and its generalization, the incomplete gamma function.

The incomplete beta function and the complete beta function are used to define the regularized incomplete beta function:

Ixa,b=Bx;a,b/Ba,b

The cumulative distribution function of the beta distribution is the regularized incomplete beta function, which is connected to the cumulative distribution function F(k;n,p) of a random variable X with probability of single success p and number of Bernoulli trials n

Fk;n,p=Pr X≤k =I1-Pn-k,k+1=1-IP(k+1,n-k)

Beta function properties

Beta function properties are given below:

• This function is symmetric, which means its value is independent of the order of its parameters,

i.e. B(p, q) = B(p, q) 

• Bp, q= Bp, q+1+ B(p+1, q)  

• Bp, q+1= Bp, q.[q(p+q)]

• Bp, q.BP+q,1-q=psin (πq)  

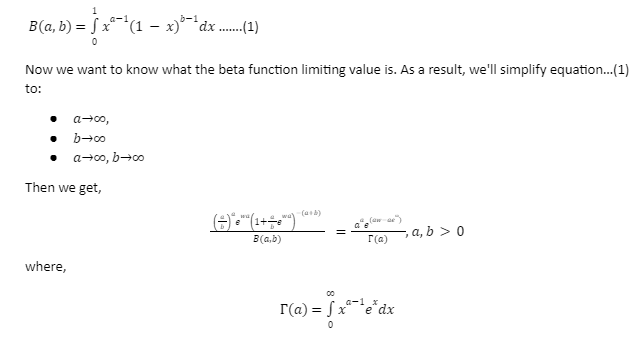
Beta function limits

As we know that the Beta function is defined as:

Beta function solved examples

Applications of Beta function

The beta function can be applied in a variety of ways. The following are some of the most prevalent applications:

• The beta function can be used to calculate the likelihood of two independent occurrences occurring at the same time in probability theory.

• It can also be used in statistics to determine a population’s variance and standard deviation.

• In engineering, the beta function can be utilized to determine a system’s impulse response.

• The beta function can be utilized to address problems involving integrals and derivatives in mathematics.

Conclusion

We study, The Beta function, commonly known as the first kind of Euler’s integrals, is a unique function. In the domains of real numbers, the beta function is defined. “β” is the symbol for it. (p, q) denotes the beta function, with p and q being real numbers. It explains how the inputs and outputs are linked. The beta function has a strong link between each input value and a single output value. In many mathematical processes, the beta function is very important.

The probability of an event occurring is calculated using the beta function. It’s a mathematical function that can be used to figure out the chances of two or more occurrences happening at the same time.