Watch Free Classes

A polygon is defined as a closed curve made up of only line segments is called a polygon. It is a closed 2-dimensional figure with two or more line segments. Polygon in Greek word ‘poly’ means many and ‘gon’ means angle.

There are many different types of the polygon. The polygons are classified according to the number of vertices they have.

The different types of polygons are as follow:

  • Triangle
    • Number of vertices of a triangle = 3.
  • Quadrilateral:
    • Number of vertices of a quadrilateral = 4.
  • Pentagon:
    • Number of vertices a pentagon = 5.
  • Hexagon: 
    • Number of vertices of a hexagon = 6.
  • Heptagon:
    • Number of vertices a heptagon = 7.
  • Octagon:
    • Number of vertices an octagon = 8.
  • Nonagon:
    • Number of vertices a nonagon = 9.
  • Decagon:
    • Number of vertices a decagon = 10.

Diagonals of a Polygon

A diagonal is a line segment connecting two non-consecutive vertices of the polygon. There are two types of boundaries interior and exterior diagonals of a polygon.

Convex and Concave Polygon

  • Polygons that are convex have no portion of their diagonals in their exterior or any of their line segment joining two different points.
  • The interior of the polygon lies wholly in the interior.
  • In a concave polygon, all interior angles are less than 180 degrees.
  • A concave polygon is a polygon in which it has more than one interior angle which is greater than 180 degrees.

Regular and Irregular Polygon

  • A regular polygon is both equiangular and equilateral, for example, a square has the same sides and same angles so it is called a regular polygon.
  • An irregular polygon is defined as a polygon in which their sides are not equiangular and equilateral.
  • Regular polygon is denoted as regular frequently.
  • Regular polygon is also known as convex polygon.
  • Irregular polygon is known as concave polygon.
  • A polygon that doesn’t have the same sides or angles at vertices is known as irregular polygon.

Angles of Regular Polygon:

There are two types of angles in a polygon, they are as follows

  • Interior angles of Polygon:

    • It is formed on the adjacent side of the polygon and is equal to each other in a regular polygon.
    • The numbers of sides are equal to the number of interior angles.
    • The interior angle value can be calculated when interior sides are known using the following formula:

Interior angle = 180 – (n – 2)/n

Where n = number of sides.

  • Exterior angles of Polygon:

    • The angle between the extension and adjacent sides are measured by extending the one sides of the polygon either in clockwise or anti-clockwise.
    • The Sum of the exterior angle of the polygon with is 360 degrees
    • All exterior angles of the regular polygon are equal.
    • If number of adjacent sides are known then exterior angle of a polygon can be calculated using following formula

Exterior angle = 3600 / n

Where n is equal to number of sides.

Polygon Formulas

The basic formulas of a polygon are as follow:

  • Area of Polygon
  • Perimeter of Polygon

Area of Regular Polygon

Based on the formulas associated with each polygon we can calculate the area of the regular polygon.

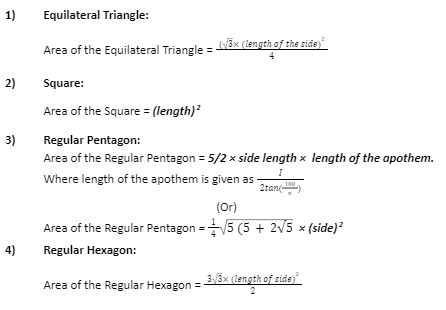
Area of Irregular Polygon

The polygon ABCD is an irregular polygon, we divide ABCD into two triangles ABC and ADC. The area of these triangles can be obtained by

Area of polygon ABCD = Area of triangle ABC + Area of Triangle ADC.

Perimeter of Polygon:

Distance around the polygon which can be calculated by getting the length of all sides is known as the perimeter of polygon.

Perimeter of the Polygon = length side 1 + length side 2 + length side 3 + ……… + length side n.

It is expressed in the units like meters, centimetres, feet, etc.

Properties of Polygon

The properties of the polygon are as follow:

  • Sum of the all interior quadrangles = 3600.
  • 1 interior angle is greater than 1800 it is called concave polygon.
  • It doesn’t cross over itself, it has only 1 boundary, so it is called simple polygon and otherwise, it is called a complex polygon.

How to find the Area of the 12-sided Polygon

  • Area of the 12-sided Polygon is as follow:
  • Sin (15) = m / r and solve for m
  • M = r × sin (15).
  • Area of each triangle
  • ½ × base × height
  • ½ × s × m
  • ½ × (s × r × sin (15)).
    • There are 12 sections so multiply 12 with result to find the area of the 12-sided polygon.
  • Area of the Regular Decagon = 6 × (s × r × sin (15))

Conclusion- 

We have discussed about the polygon in detail and talked about the different types of polygon in detail. We have talked about the concave and convex polygon. We have even talked about the properties of the polygon too.