Profile
Settings
Refer your friends
Sign out
One of the most fundamental chapters in basic mathematics is algebra.Algebraic Identities are introduced to students in the lower grades, at the high school level, and then in the upper grades, where they acquire greater levels of algebraic Identities.Algebraic identification is a vast concept that can be applied to many different aspects of a student’s life.An algebraic identifier is a set of algebraic equations that apply to all of the variables in the set.An algebraic equation is a mathematical expression made up of numbers, variables (unknown values), and mathematical functions (addition, subtraction, multiplication, division, and so on). It’s primarily used to discover polynomial elements.An algebraic identifier is an algebraic equation that refers to all of the values of a variable in it.It is also employed in the factoring of polynomials.As a result, algebraic identifiers are utilized to calculate algebraic expressions and solve various polynomials. In your younger classes, you must have learned about Algebraic Identities. An algebraic identifier is an algebraic equation that refers to all of the values of a variable in it.It is also employed in the factoring of polynomials. As a result, algebraic identifiers are utilized to calculate algebraic expressions and solve various polynomials.
Algebraic Identities from Binomial Expansion Formula:
The binomial expansion, which is based on the binomial theorem, may be used to deduce all algebraic identities.Let’s get a quick overview of the binomial theorem expansion, which will come in handy for deriving higher-degree algebraic identities.Binomial expansion is typically used for expansions with two variables and n degrees.The binomial expansion has a total number of terms of (n + 1), and in the expanded version, the power of the first variable decreases while the power of the second variable increases in successive terms. A coefficient is connected with each term in the binomial expansion.
The algebraic identities for sum and difference of variables, as well as for a maximum power of 3, have been derived from the binomial expansion and are listed below as formulas. The above binomial expansion formula can also be used to create algebraic identities with higher degrees and more variables.
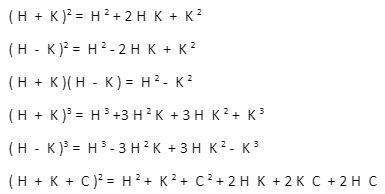
Algebraic Identities for Factorization:
Algebraic identities are extremely useful for factoring algebraic expressions quickly. Using the algebraic identities below, the given expression representing the expanded form of the formula can be translated and represented as a set of factors.Higher algebraic identities like a4 – b4 can be easily deduced from the basic algebraic identities a2 – b2. The list below contains a number of algebraic identities that can be used to factor polynomials.
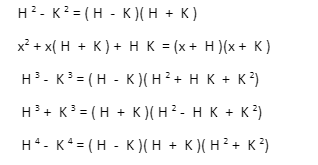
Algebraic Identities for Three Variables:
The binomial expansion formula was also used to generate the algebraic identities for three variables. Furthermore, these identities are useful in reducing the number of steps required to work across algebraic expressions.
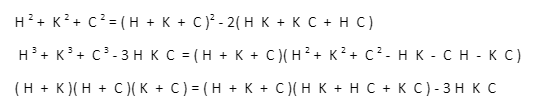
Important points:
- The algebraic expression can be defined as the set of constants and variables linked by the signs of the basic operations of addition, subtraction, multiplication, and division.
- A monomial, binomial, trinomial, or quadrinomial is an algebraic expression with one, two, three, or four terms, respectively.
- When adding or subtracting polynomials, seek for like terms first, then add or subtract them. Finally, deal with the unlike terms.
