The term “addition” refers to the joining of two or more vectors. We add two or more vectors together by utilising the addition operation to create a new vector that is equal to the sum of the two or more vectors that were previously added. Vector addition has applications in the physical sciences, where vectors are used to express physical quantities such as velocity, displacement, and acceleration.

This article will discuss vector addition, addition formulae, their characteristics, and numerous rules, provided with solved examples.

What is Vector Addition? 

A vector represents direction and magnitude. So, it is usually represented with an arrow above the quantity. For example, Vector a is written as a and so on. When you add two vectors, the resultant is written as a+b

There are some basic principles to keep in mind for the addition of vectors:

• Vector addition is only possible when the vectors are of the same nature. For instance, you can add acceleration only with acceleration and not any other quantity.
• Scalars and vectors cannot be added.

Basic Properties for Addition of Vectors

Whether you are adding vectors graphically or using any other method, you must remember that algebraic addition is quite different from vector addition. Some basic properties will come in handy to help you arrive at the correct answer.

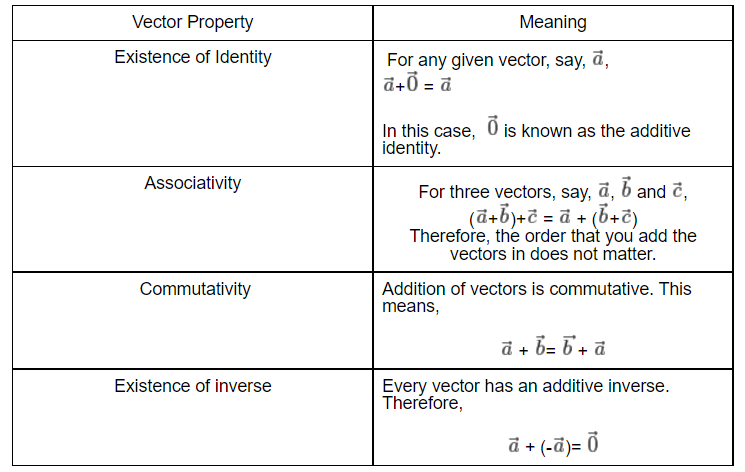
The first aspect that you would have to consider about adding two vectors together is to remember a few rules related to the addition and subtraction of vectors. The following tips will enable you to do it graphically as well.

• You have to use geometry to add two vectors and not algebra
• The calculation may be about finding the sum of each vector, but the vectors behave independently and follow specific laws individually
• The final result equals the count of vectors acting on a particular body at any given point in time
• The result of vector addition is cumulative. In other words, the resultant number/direction is not dependent on the order of the vectors that are being calculated.

You may illustrate the process of summation of two or multiple vectors by providing vector addition and subtraction examples.

Some of the other laws to consider before attempting addition and subtraction of vectors are:-

Triangle Law

Adding two vectors represented by a  and b can be done by drawing a line AB where A forms the tail and B is the head.

• Draw a second line BC where B forms the tail and C its head
• Join the points A and C by another line. The resulting line AC will give you the sum of the two vectors.

Parallelogram Law

The addition and subtraction of vectors, particularly the addition procedure, can be explained with a parallelogram. The law elaborates the process in the following manner:-

• P and Q represent two sides of a parallelogram that are adjacent to each other. Each side points in an external direction
• A diagonal line drawn from the intersecting point of P and Q will give the result, one diagonal will be addition of two vectors, while the other one will be their subtraction.

Polygon Law

This particular law considers multiple counts of vectors. When their magnitude, along with the direction of each is considered in an order of the sides of a polygon, the result is evident by the magnitude and the direction considered in the opposite direction of its sides. The addition and subtraction of vectors by using a polygon can be depicted by the following method:-

Name the four vectors as A, B, C, and D consecutively. You now need to obtain the results by adding all four-vectors. You may want to segregate the sides A and B and form a triangle. The opposite direction of both vectors will provide you with the result of adding these two vectors. Now consider the opposite triangle created out of the polygon in the same manner. You will be able to figure out the total of A through D by tracing the direction and taking the total displacement into account.

Conclusion:

The addition of vectors becomes simpler when you understand addition formulae. As a revision, here are some principles that you should remember when adding vectors:

• You can only add vectors that represent the same quantity. For instance, you can add two vectors that represent acceleration. But not when one vector represents acceleration, and the other represents force.
• Vector addition is both associative and commutative in nature.
• You cannot add vectors and scalars.

• The triangle law of vector addition is considered the first rule of vector addition.