Hund’s Rule
Introduction
Hund’s rule can be defined as a largest total spin state of an atom which increases the stability of an atom. Hund’s rule is very reliable for determining the phase of a given excited electronic configuration. Friedrich Hund discovered this rule in 1925. Hund’s rule states that:
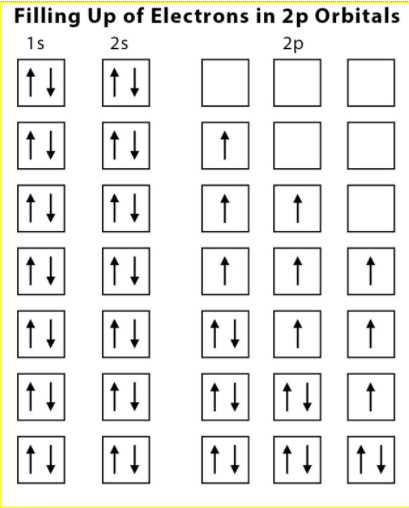
- Pairing of electrons in the orbitals to the same subshell (p, d,f ) does not take place until each orbital belonging to that subshell has one electron in each.
- Every electron in all the subshells must be present with the same spin.
Primary rule is that pairing should be done when all the orbitals of the same shell are filled by one electron. After each orbital fill by the same spin electron pairing should be done. Quantum-mechanics,states the electrons are occupied in orbitals and are less effectively shielded from the nucleus.
Secondary rule is that electrons in singly occupied orbitals that are unpaired have an equivalent amount of spins.
Body
Hund’s Rule Of Maximum Multiplicity
According to Hund’s Rule of Maximum Multiplicity, a given electron configuration falls lowest in energy. Consistent with this rule, electron pairing in p, d and f orbitals cannot occur until each orbital of a given subshell contains one electron each or is singly occupied. Before pairing up, the electrons enter an empty orbital. The electrons repel one another as they’re negatively charged. The electrons don’t share orbitals to scale back repulsion.
When we consider the second rule, the spins of unpaired electrons in singly occupied orbitals are equivalent.The theory behind this rule is that, for a stated electron configuration, the best value of spin multiplicity has the bottom energy term. It says if two or more orbitals having an equivalent amount of energy are unoccupied then the electrons will start occupying them individually before they fill them in pairs. It’s a rule which depends on the observation of atomic spectra, which is useful in predicting the bottom state of a molecule or an atom with one or quite one open electronic shell.
Electron Configuration and its Purpose
Electron Configuration
The valence shells of two atoms that are in contact with one another will interact first. When valence shells aren’t full then the atom is the least stable. The chemical characteristics of a component are largely hooked into the valence electrons. Similar chemical characteristics are often seen in elements that have similar valence electron numbers.
The stability also can be predicted by the electron configuration. When all the orbitals of an atom are full, it means it is most stable. The orbitals that have a full energy state are the foremost stable, like noble gasses. These sorts of elements don’t react with other elements.
When atoms inherit contact with each other, outermost electrons of those atoms, or valence shells, will interact first. An atom is least stable (and therefore most reactive) when its valence shell is incompletely full. The valence electrons are largely liable for an element’s chemical reactivity. Elements that have an equivalent number of valence electrons often have similar chemical properties.
Electron configurations also can predict stability. An atom remains in its most stable form ( unreactive state ) when all its orbitals are full. The foremost stable configurations are those that have full energy levels. These configurations occur within the noble gasses. The noble gasses are very stable elements, they don’t react easily with the other elements. Electron configurations
help in making predictions about ways in which certain elements will react and therefore the chemical compounds or molecules that different elements will form.
The multiplicity of a state of an atom can be defined as 2S + 1. Here, S is the total electronic spin. A high multiplicity state is, therefore, an equivalent to a high-spin state. If the spin of every electron is 1/2 then the entire spin is one-half the number of unpaired electrons. Thus the multiplicity is the (number of unpaired electrons + 1). The nitrogen atom state has three unpaired electrons of parallel spin and the entire spin comes 3/2. Therefore the multiplicity is 4.
Due to the low energy and increased stability of an atom, the high-spin state has unpaired electrons of parallel spin, which reside in several spatial orbitals consistent with the Pauli exclusion principle. An early explanation that was regarded as incorrect about lower energy of high multiplicity states that the various occupied spatial orbitals create a larger average distance between electrons. And this reduces the electron-electron repulsion energy. However, quantum-mechanical calculations with accurate wave functions showed a particular physical reason for the increased stability might be a decrease within the screening of electron-nuclear attractions. Thus, the unpaired electrons can approach the nucleus closer than the electron-nuclear attraction gets increased.
Hund’s Principle
This law states that an orbital cannot have all the electrons within the same spin motion, and the electrons are going to be in either positive half spin (+1/2) or negative half spin (-1/2). Hence, argon’s electron configuration can be written as 1s2 2s2 2p6 3s2 3p6. The 1s level can accommodate two electrons with the same n, l, and m quantum numbers. Argon’s pair of electrons in 1s orbital satisfies the Pauli exclusion principle as they need opposite spins. This determines different spin quantum numbers, One spin is +½. The opposite is -½. The 2s level electrons have a separate principal quantum number to those within the 1s orbital. a few of 2s electrons differ from one another because they need different spins. The 2p level electrons have a special orbital angular impulse number from those within the s orbitals, hence the letter p instead of s. There are three p orbitals of comparable energy, Px, Py, and Pz. These orbitals are different from each other. All the Px, Py and Pz orbitals have a pair of electrons with opposite spins. The 3s level rises to a higher quantum number, and this orbital contains an electron pair with opposite spins. The 3p level’s information is analogous for 2p, but the principal quantum number is higher: 3p lies at better energy than 2p.
Conclusion:
Hund’s Rule will help predict the properties of atoms, as paired and unpaired electrons have distinct properties (particularly with magnetic fields). The outer shell electrons of atoms or valence shells interact when they come closer to each other. An associated atom is highly unstable (most reactive) when its valence shell is incompletely full. The valence electrons are most liable for an associate element’s chemical reactivity. Parts have the same range of valence electrons, and they have similar chemical properties.
An associate atom is most stable (unreactive) once its orbitals are filled with electrons. These configurations are found within the noble gasses, which are extremely stable and don’t normally react with one another
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



