The term adsorption signifies the process of accumulation of particles referred to as adsorbate at the surface of another material referred to as adsorbent. In the case of adsorption, the interaction between the adsorbent and adsorbate does not result in the accumulation of both in bulk. The term isotherm refers to mapping points where the temperature remains constant over time. Adsorption Isotherm is a graphical representation of the adsorption rate of the adsorbate on the surface of the adsorbent with changing pressure under constant temperature. The primary methods used to calculate the capacity of material adsorption are Freundlich and Langmuir adsorption isotherms.
What is adsorption?
The term adsorption is not to be confused with absorption. Adsorption is a process of deposition of one molecular substance on the surface of another substance. This process does not involve the interaction of both substances into the molecular bulk. There are two substances involved in the process:
Adsorbate: The substance that gets accumulated on the surface of another substance
Adsorbent: The substance on the surface of which an adsorbate is accumulated
The reason for the adsorption of substances on the surface of another substance is the difference in the environment of the surface particles and bulk particles. In the bulk of a substance, the atoms or molecules are in balance and are surrounded by similar particles. However, the atoms or molecules are not surrounded by similar particles on all sides of the surface. Due to this difference in surface and bulk of a substance, it causes an unbalanced attractive force. These residual forces attract the adsorbate on the surface of the adsorbent.
During adsorption, the residual forces on the surface of the adsorbent are decreased and released in the form of heat. Thus adsorption is an exothermic process; that is, the reaction releases energy into the surrounding in the form of heat. The measurement of heat released to adsorb one mole of adsorbate on the adsorbent is known as the enthalpy of adsorption. With the process of adsorption, the molecular movement of the adsorbate is restricted, and thus, the entropy of the adsorbate is decreased. Thus H and S are negative in the case of adsorption, where H represents the change in enthalpy of the reaction and S represents the entropy of the reaction. Adsorption capacity can be represented by the Langmuir and Freundlich isotherm.
Types of adsorption:
Physical Adsorption or Physisorption:
The process of adsorption of gasses on solid surfaces due to weak van der Waals forces is known as physisorption or physical adsorption
Chemical Adsorption or Chemisorption:
The process of adsorption where the adsorbate is held to the surface of the adsorbent by chemical bonds, either covalent or ionic, then is called chemical adsorption or chemisorption
Freundlich Adsorption Isotherm:
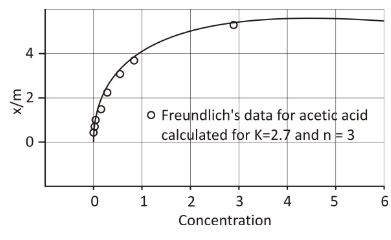
In 1909, Herbert Freundlich came up with a relationship between the amount of adsorbate (gas) adsorbed by the unit mass of adsorbent and pressure at a given temperature. The equation to calculate for the adsorption isotherm proposed by Freundlich was:
x/m = k*p(1/n) —– (eq:1)
Where,
x represents the mass of the adsorbate or the gas
m represents the adsorbent’s mass on which the gas is adsorbed
P is the pressure
n and k are constants depending upon the nature of gas and adsorbent at a given temperature
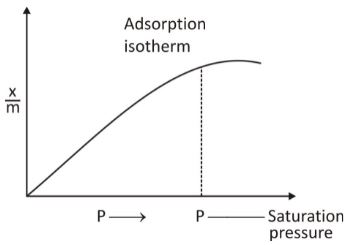
This representation of adsorption is often plotted as a graph showing the mass of gas adsorbed per unit mass of adsorbent against pressure. The graph shows that there is a decrease in adsorption with increasing temperature. The plotting on the graph shows that at the highest pressure, saturation is achieved.
On taking the log of the above-mentioned equation, we get:
log x/m = log k + (1/n) log P —-(eq:2)
The Freundlich Adsorption Isotherm can be validated by a graph where log x/m is plotted along the ordinate or y-axis, and log p is plotted on the abscissa or x-axis. If the graph shows a straight line, then the isotherm is validated. The slope of the plotted graph represents the value of 1/n, and the intercept represents the value of log k in the y axis.
In Freundlich Adsorption isotherm, the value of 1/n is limited between 0 and 1. Thus the log of eq:1, i.e., eq:2, will only apply to a minimal pressure range.
When the 1/n value computes to 0, the adsorption becomes independent of pressure, and when the 1/n value computes to 1, then x/m = k*p, thus adsorption is directly proportional to pressure. At the same time, Freundlich isotherm cannot explain that saturation is achieved at the highest pressure point. Thus, this isotherm does not hold applicable to very high pressure.
Langmuir Adsorption Isotherm:
Langmuir Adsorption Isotherm defines the equilibrium of adsorption where there is a single layer of adsorbate molecules on a homogeneous surface of the adsorbent when a relative pressure of unity is achieved. This isotherm is represented as
Θ = Kp/1+Kp
Where,
Θ represents the surface of the adsorbent
K is adsorption coefficient which is an equilibrium constant
P is the pressure
This is a theoretical representation of the process of adsorption.
Difference between Freundlich and Langmuir adsorption isotherm:
Adsorptions from the solution phase:
Adsorption can also take place by a solute in a solution. When a solute is mixed with a solvent, the solute is partly absorbed by the solvent. For example: if diluted acetic acid (acetic acid mixed in water) is added to charcoal and mixed, a part of the acid is absorbed by the charcoal leading to a decrease in the solution’s acidity.
The process of adsorption in the solution phase has the following characteristics:
1. With the increase in temperature, the adsorption is decreased. In other words, the extent of adsorption is inversely proportional to temperature
2. The nature of the adsorbate and adsorbent directly impact the extent of adsorption
3. With increasing surface area of adsorbent, adsorption is increased. That is, the extent of adsorption is directly proportional to the surface area of the adsorbent
4. The adsorption depends on the concentration of the solute into the solution
Freundlich’s equation can represent the adsorption capacity in the solution phase by replacing pressure with a concentration of the solution.
x/m = k*C1/n —- (eq:3)
Where,
C represents the concentration of solution after completion of the adsorption process.
Like the Freundlich Adsorption Isotherm equation, if the log of eq:3 is plotted in a graph with log x/m plotted against log C, a straight line will validate the Freundlich’s isotherm.
Conclusion:
Adsorption Isotherm is the process to compute the adsorption capacity of a particular substance. Using Freundlich and Langmuir Adsorption isotherm, we can validate the change in adsorption with a change in pressure at a constant temperature. We can also represent the adsorption process in the solution phase. The solute is adsorbed in the solution by taking different solutions in different concentrations and applying the Freundlich Adsorption Isotherm formula to plot the graph. We can measure the adsorption changes in different circumstances through the empirical and theoretical representation of Freundlich and Langmuir adsorption isotherms.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



