Covariance formula
Covariance states the relationship between two random variables. The formula is applied to get the variance between two variables. It states that till what range with the change in one variable changes together. If one variable is of a higher range other will also have a higher range and lower the one variable other would be of a lower range. Covariance is measured in units.
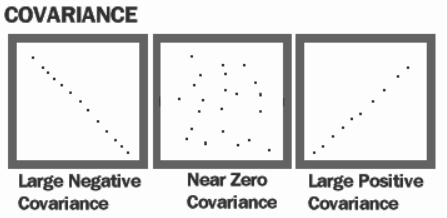
Positive covariance-
When both the variables move in the same direction it is called positive covariance.
Negative covariance-
When both the variables move in opposite directions it is said as negative covariance.
In the formula of covariance, the covariance between two variables X and Y can be denoted as Cov(x,y). The formula of covariance varies in some circumstances. Formulas for the covariance of population and sample are different.
Covariance formula for population
Cov(x,y) = ∑(xi – x ) × (yi – y)/ (N)
Covariance formula for sample
Cov(x,y) = ∑(xi – x ) × (yi – y)/ (N – 1)
Where, Xi –values of the X-variable
Yi – values of the Y-variable
X̄ – mean of the X-variable
Ȳ – mean of the Y-variable
n – number of data points
The covariance formula can be also written as
ρ(X,Y)=Cov(X,Y)/σX * σY
ρ(X,Y) – correlation between the variables X and Y
Cov(X,Y) – covariance between the variables X and Y
σX – standard deviation of the X-variable
σY – standard deviation of the Y-variable
Example
1. Find covariance for following data x = {6,5,3,4,2}, y = {12,10,8,6,4}
Given data, x = {6,5,3,4,2}, y = {12,10,8,6,4} & N = 5
X̄ = (6 + 5 + 3 + 4 + 2) / 5
= 20 / 5
= 4
Ȳ = (12 + 10 +8 + 6 + 4) / 5
= 40 / 5
= 8
Using the sample covariance formula
Cov(x,y) = ∑(xi – x ) × (yi – y)/ (N – 1)
= [(6 – 4)(12 – 8) + (5 – 4)(10 – 8) + (3 – 4)(8 – 8) + (4 – 4)(6 – 8) + (2 – 4)(4 – 8)] / 5 – 1
= 8 + 2 + 0 + 0 + 6 / 4
= 16 / 4
= 4
Using the Population covariance formula
Cov(x,y) = ∑(xi – x ) × (yi – y)/ (N)
= [(6 – 4)(12 – 8) + (5 – 4)(10 – 8) + (3 – 4)(8 – 8) + (4 – 4)(6 – 8) + (2 – 4)(4 – 8)] / 5
= 8 + 2 + 0 + 0 + 6 / 5
= 16 / 5
= 3.2
2. The standard deviation of X is 15 and the standard deviation of Y is 20. The correlation between X and Y is 5. Find covariance of X and Y.
Given data, σX =15, σY =20 and ρ(X,Y) = 5
ρ(X,Y) = Cov(X,Y)/σX * σY
5= Cov(X,Y)/ 15*20
Cov(X,Y)= 1500
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out