Types of Numbers- Perfect Numbers

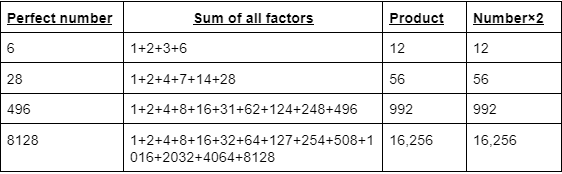
3 is not a perfect number because the factors or 3 is 1 and 3 whose sum is 4 so if it is more than the number 3 or if we exclude 3 then the factor 1 is less than the number 3 so it is not a perfect number. Likewise, 4,5,7 and so on is also not a perfect number.

Perfect Numbers Definition:

Perfect numbers are the special type of positive integer number in which the sum of all factors of that number excluding will be equal to that number or the sum of all factors including itself is the multiple of 2 of that number. For example; 28, the sum of its factors is 1+2+4+7+14+28=56 which is the multiple of 28 or we can say the sum of factors excluding itself is 1+2+4+7+14=28 which is equal to 28 so it is a perfect number.

 The aliquot number is the number whose sum of all factors excluding the number itself is equal to that number:

 for example: 6 sum of factors excluding itself is 6 so it is an aliquot composite number.

History of perfect numbers:

The history of perfect numbers is not yet known accurately. It has been said that Pythagoreans studied these numbers for mystical properties which was continued by Nicomachus of Gerasa, the Neo-Pythagorean philosopher in around AD 100 who divided the numbers on the basis of the sum of their divisors i.e. either less than, equal to or greater than that particular number and are termed as deficient numbers, perfect numbers and superabundant numbers respectively. Euclid and Leonhard Euler gave their theorem around 300 BC.

The early Greek mathematicians and Nicomachus know the first four perfect numbers; 4, 28, 496 and 8128.” On the creation” the first book which mentions the perfect numbers written in a first-century book by Philo of Alexandria who claimed that the moon orbits were created in 28 days and that the world was developed in 6 days therefore 6 and 28 are the perfect numbers.

Origen, then followed by Didymus the Blind states that there are only 4 perfect numbers below 10,000. Further, in the early 5th century AD, St Augustine described the perfect number in his book “City of God”. Ismail ibn Fallūs, the Egyptian mathematician stated 33,550,336; 8,589,869,056; and 137,438,691,328 as next three perfect numbers after 4, 28, 496 and 8128.

Euclid-Euler theorem:

It is the theorem that connects the perfect numbers and Mersenne primes. This theorem states that if and only if even number has 2p−1×[2p− 1]  form, only then an even number is a perfect number where 2p −1 is a prime number and p represents the positive integer.

Mersenne prime is the prime number having formula M = 2n − 1 where n represents the integer. Euclid and Leonhard Euler were the mathematicians who proved this theorem and named it.

Types of perfect numbers:

There are two types of perfect numbers; odd perfect numbers and even perfect numbers.

1. Odd perfect numbers: It has been known that there are no odd perfect numbers that satisfy the conditions like it should not be divided by 105 should be greater than 10^1500.
2. Even perfect number: Euclid-Euler theorem applies for even perfect numbers which states that if and only if even number has  2p−1×[2p− 1] form, only then an even number is a perfect number where 2p −1 is a prime number and p represents the positive integer. For example: if p=2,

2p−1×[2p− 1]

=22−1×[22− 1]

=21×[4 − 1]

= 2×3 

= 6, an even perfect number.

Conclusion:

Perfect numbers are the special and unique numbers that are equal to the sum of all the factors of that particular number excluding itself. Euclid-Euler theorem gives formula 2^(p−1)*[2^(p)− 1] for even perfect numbers and it also links the Mersenne primes and the perfect numbers. Odd perfect numbers are not yet known. 4, 28, 496, 8128, 33,550,336 8,589,869,056; and 137,438,691,328 are some of the examples of perfect numbers. The aliquot number is the perfect number. Pythagoreans were the first who uses the term perfect numbers which are then followed by Nicomachus of Gerasa.