A Brief Study on Syllogisms

Syllogism questions are an important part of aptitude test papers these days. It is a subcategory of logical reasoning. As a result of its baffling character, syllogisms have gained prominence in aptitude tests.

The subject of a Syllogism is frequently covered in government examinations, and candidates should expect to find questions on it. As a result, a thorough comprehension of what is Syllogism in reasoning and the relation between logical reasoning and Syllogism is necessary. 

The syllogism question should be tackled last because it requires a high level of patience and logical reasoning. But not at all difficult if you understand the common patterns in the Syllogism-based questions, which will be discussed in this article. 

What is Syllogism in Reasoning?

One can infer or conclude by looking at the root of the term, syllogism, which is Greek for “conclusion.” Deductive reasoning is used in syllogisms to come to a conclusion by a logical argument of assertions. Aristotle is credited with making the most significant contribution to the field of syllogisms.

This section has two or more statements, and two or more conclusions follow these statements. One must determine which of these inferences logically follows the provided statements. Although they may appear to contradict widely accepted truths, the claims must be regarded to be truthful.

Syllogisms can be solved in a variety of ways. Using a Venn diagram is the most effective and efficient approach of all. One should design all feasible diagrams based on the supplied assertions and then solve each one individually. In the end, the solution that appears in all of the diagrams is determined to be correct.

Relation Between Logical Reasoning and Syllogism

The relation between logical reasoning and Syllogism is such that it is a part of logical reasoning, or more specifically, analytical reasoning. Candidates must draw inferences from supplied statements to pass this test. When completing Syllogism problems, the candidate must assume that the following propositions are 100% true. Candidates are tested on their ability to conclude from supplied statements using step-by-step approaches.

Common Patterns in the Syllogism-Based Questions

There are certain common patterns in the Syllogism-based questions, which can significantly help students perform better at them if recognised. Each Syllogism question is divided into three parts: 

1. Major premise – A statement or a concept that the author believes in
2. Minor premise – An example of the major premise
3. Conclusion – A specific statement that logically follows both major and minor premises.

Example 1 (Solving Using Venn Diagram)

Major premise – All men are strong

Minor premise – Vijay is a man

Conclusion – Vijay is strong

Let us conclude with the help of a Venn diagram.

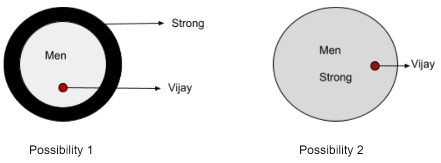
Major and minor assertions are combined in two distinct ways in the above. “All men are strong” is in the Venn diagram of strong. It is, therefore, logical that the main claim is correct. Because he is a man, Vijay’s Venn diagram belongs inside the Man one. Venn diagrams of Men and strong overlap in the second scenario, which differs from the first one. Because there is a second option, because Vijay is a man, he is a part of it. The conclusion that “Vijay is strong” can be drawn from both circumstances; thus, the statement is true. 

Example 2 (Using Analytical Thinking)

Directions: Three assertions are presented in the following question, followed by three conclusions, I, II, and III. You must accept the offered claims as true, even if they appear to contradict generally held beliefs. Read through all of the conclusions and determine which of the following logically follows from the supplied assertions, taking into account frequently known facts.

Statements

• All cars are black.
• Some bikes are white. 
• Not all bikes are black.

Conclusion

1. All bikes are white.
2. Some cars are white.
3. Some bikes are black.

Solution

1. All bikes are white. → False statement (only some bikes are white)
2. Some cars are white. → False statement (not cars are white, all black)
3. Some bikes are black → True (since some bikes are white, it is possible that some bikes can be black also)

Thus, only conclusion III follows. 

Please note that the conclusion must be true in all feasible scenarios. Before drawing any conclusions, it is prudent to consider every conceivable scenario. 

Types of Syllogism Questions Asked

As we know about the Syllogism reasoning portion, we can prepare better. We’ll look at some questions that may be asked.

1. Basic Syllogism – There can be no exceptions to the rule of absolute truth when using Syllogisms of this sort. False conclusions will be evaluated even if they are 99% accurate.
2. Either-Or Situation- The either-or situation is generated in Syllogism reasoning when the conclusions aren’t 100% true, but the two offered conclusions are 50% correct.
3. Coded Syllogism – Statements and conclusions are encoded in this Syllogism reasoning. One can find a solution by deciphering statements and conclusions.
4. Sequential Syllogism – Choices follow statements in this kind of Syllogism reasoning. The first and second assertions must be logically connected to get at the third statement.

Conclusion

Syllogisms are fundamental to the process of logical thinking. Various entrance exams commonly include questions on this subject. Syllogism is an analytical method that relies on evaluating two or more assertions or propositions to arrive at a conclusion. Deductive reasoning is used instead of inductive reasoning. Even if the supplied claims contradict well-established truths, you must accept them as true.