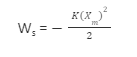A spring exerts a force against the force we apply while extending the spring to attain an equilibrium position. Every spring has its stiffness, known by the spring constant (k). Springs with large spring constants are stiff and are difficult to compress.
According to Newton’s Third Law of Motion, when force is applied to the spring, it exerts back an equal magnitude force but in the opposite direction. Therefore, to know the exact magnitude of energy required to stretch or compress for displacement in the spring, we should use the work done by the spring formula. By plotting the equation on the graph, we get a linear correlation between the force (y-axis) and the spring’s displacement (x-axis) by plotting the equation.
Hooke’s Law
Hooke’s Law states that the force required for changing the spring’s shape is proportional to the displacement in the spring. Here, displacement is how far the spring is stretched or compressed from its original shape.
Mathematically, Hooke’s Law equation can be written as F = -kx
Where,
F = Force required to change the spring’s shape
k = spring constant
x = displacement
The spring constant is different for different springs. It depends on factors such as the thickness of the spring’s coiled wire and the material of the spring. Hooke’s Law is negative because it is a restoring force, but the positive version is a valid representation. The unit of measurement is Nm-¹.
Potential energy of the spring
Springs regain their regular shape despite putting force on them by compressing or stretching them. It is because the spring stores potential energy. Whenever a spring is compressed or stretched, it returns to its standard shape when the stress is relieved. It is possible because of its stored elastic potential energy.
As a result of deformation, the potential energy stored in an elastic object is Elastic Potential Energy. For example, the elastic potential energy of a spring is equal to the work done for stretching the spring. The work done depends on the spring constant k and the distance stretched.
Calculating the potential energy of a spring
We use Hooke’s Law for calculating the potential energy of a spring. The potential energy is equal to the work done by the spring. It is evident from work done by the spring formula that it is the product of force and distance. We obtain our force from Hooke’s Law, and distance will be the displacement in the spring’s position.
Suppose a spring is fixed to a rigid wall and is ideal and massless. Now suppose the spring is stretched so that it displaces by a distance x from its equilibrium position and is compressed such that it displaces by a distance -x from its equilibrium position. If the resultant displacement is measured equal to Xm, work done by the spring formula can be derived as
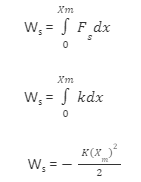
Although the work done by the spring formula is negative, the elastic potential energy of the spring is not negative. On the contrary, at the very moment when the spring is stretched or compressed, positive potential energy is stored in the spring.
The work done for pulling the spring is
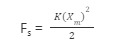
The force is positive because it overcomes the spring’s force.
Suppose a spring is compressed by a distance x. The spring is moved from an initial displacement Xi to a final displacement Xf. The work done by the spring formula is
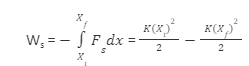
When the spring is in an equilibrium position, the potential energy of a spring is zero. On extending the spring for a displacement X, the ends are stationary, and hence the kinetic energy of the spring is zero. Thus, it is clear from above that the potential energy of a spring is equal to the work done by the spring formula.
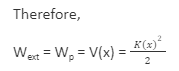
Above is the derivation of work done by the spring formula.
Work done by spring example
To understand the calculation of work done by spring, consider the following work done by spring example:
A metal piece slides into a spring of coefficient 300 N/m. It compresses the spring by 1 m. How much work did the spring do to stop the metal piece?
We know
k = 300 N/m
x = 1 m
The metal piece is stopped. Hence, all of its energy must have gone into the spring through work done on the metal piece for stopping it. Therefore, the spring’s elastic potential energy will equal the amount of work done on the metal piece. Therefore, calculating the work done by the spring formula is equal to calculating the potential energy of a spring.
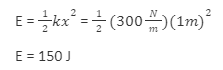
Thus, the work done by spring is around 150 J.
Conclusion
Spring is an object which extends or compresses on applying force. The force required should be more than the spring constant (k) to displace the spring from its equilibrium position. We use the work done by the spring formula to calculate this force. Spring also possess potential energy. For calculating the potential energy of a spring, we use Hooke’s Law. According to Hooke’s Law, the potential energy of the spring is equal to the work done by the spring. Thus, E = W = 1 ⁄ 2kx².
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out