A simple Harmonic motion is back and forth motion about a fixed axis or a straight line. It can be defined as a type of oscillatory motion or periodic motion. 

In SHM, the acceleration acting on the body varies directly with the displacement and is opposite to the direction of displacement. Therefore, the net force which acts on the body also varies directly with the displacement and in the direction opposite to it. A motion of a spring-mass system describes an SHM. A block suspended from a spring is termed a spring-mass system. 

There are two types of Simple Harmonic motion-Angular and Linear.

Linear SHM

A body is said to be in linear SHM when it moves to and fro about a mean position. A spring mass system, which is a block of mass suspended from a spring, is an example of a linear SHM. 

For an SHM to be categorised as linear, it should fulfil the following conditions:

• Force should be directly proportional to displacement and in a direction opposite to it, that is, F  ∝-x
• Acceleration should be directly proportional to displacement and in a direction opposite to it, that is, a  ∝-x

A differential equation for linear SHM can be obtained as follows:

• We know that for a linear SHM, F  ∝-x. Therefore, F=-kx, where k represents restoring force constant.
• We also know that force is given by F=ma, where m and a represent mass and acceleration respectively.
• Equating both equations of force, we get,

ma=-kx

or,  

a=-kx ⁄ m

• Velocity of an object is given as v=dx/dt, where dx represents displacement and dt represents time.
• Since acceleration is the rate of change of velocity, we can obtain acceleration by differentiating the equation for velocity.

a=dv ⁄ dt=d²x ⁄ dt²

• Equating both acceleration equations, we get

d²x ⁄ dt²=-kx ⁄ m

Or,

d²x ⁄ dt²+kx ⁄ m=0

• Let k ⁄ m=ω², where represents the angular velocity. Therefore, the above equation can be written as,

d²x ⁄ dt²+ω²x=0

Therefore, the differential equation for linear SHM can be written as
d²x ⁄ dt²+ω²x=0

Angular SHM

A body is said to be in angular SHM when it rotates or oscillates freely about a given axis. The motion of a mass attached and suspended by a string (simple pendulum) is an example of angular SHM. 

For an SHM to be categorised as angular, it should fulfil the following conditions:

• The angular acceleration or restoring torque (τ) acting on the system should be directly proportional to the angular displacement (θ), that is, τ  ∝-θ

A differential equation for angular SHM can be obtained as follows:

• We know that for an angular SHM, τ  ∝-θ. Therefore, =-cθ, where c represents restoring torsion constant.
• Torque is also given as τ = Iα, where I is the moment of inertia and is angular acceleration.
• Equating both equations of torque, we get,

Iα=-cθ

Or,

Iα+cθ=0

• Angular velocity of an object is given as v=dθ/dt, where dθ represents angular displacement and dt represents time.
• Since acceleration is the rate of change of velocity, we can obtain acceleration by differentiating the equation for velocity.

a=dv ⁄ dt=d²θ ⁄ dt²

Sinceα =d²θ ⁄ dt²  the equation (Iα+cθ=0) can be written as,

I d²θ ⁄ dt² +cθ=0

• Therefore, the differential equation for angular SHM can be written as,

I d²θ ⁄ dt² +cθ=0

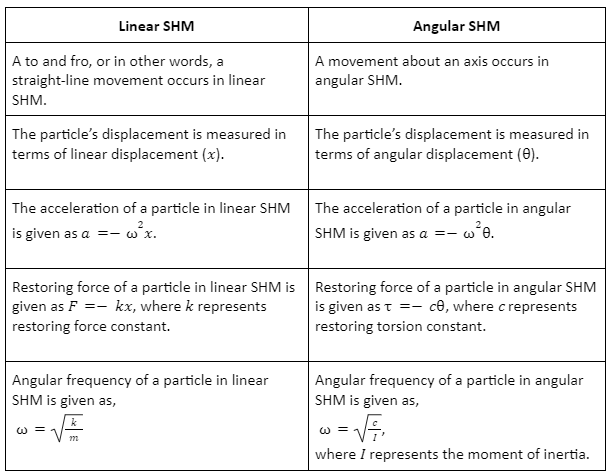
Difference between linear and angular SHM