Profile
Settings
Refer your friends
Sign out
A magnetic dipole is an arrangement of two equal and opposite magnetic poles separated by a little distance. The product of a magnetic dipole’s pole strength and magnetic length is the magnetic dipole moment. It’s a vector quantity that goes from the S-pole to the N-pole.
Ampere discovered that the magnetic line of force distribution around a limited current carrying solenoid is comparable to that of a bar magnet.
Magnetic moment: The torque on a current loop is τ = MB sin θ, where θ is the angle between magnetic moment and magnetic field.
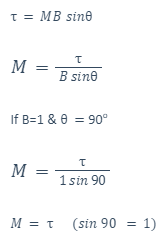
That is, the magnetic moment of a current loop is defined as the torque acting on the loop when placed in a magnetic field of 1T such that the loop is oriented with its plane normal to the magnetic field.
Also, M = NIA i.e.,
The magnetic moment of a current loop is the product of several turns (N), the current flowing in the loop (I) and the area of the loop (A). Its direction is perpendicular to the plane of the loop. The magnetic moment of Revolving Electron,
M = (evr)/2
M= magnetic moment
e= charge of electron
v=velocity of electron
r=radius of the path of the electron
Magnetic dipole:
An arrangement of two equal and opposite magnetic poles separated by a small distance is called a magnetic dipole.
Magnetic dipole moment:
The magnetic dipole moment of a magnetic dipole is defined as the product of its pole strength and magnetic length. It is a vector quantity, directed from S-pole to N-pole.
Ampere found that the distribution of magnetic lines of force around a finite current-carrying solenoid is similar to that produced by a bar magnet.
The magnetic induction at a point along the axis of a circular coil carrying current is
Hence a current loop is equivalent to a magnetic dipole of moment M = IA
The magnetic moment of a current loop is defined as the product of the current and the loop area. Its direction is perpendicular to the plane of the loop.
The magnetic dipole moment of a circular current-carrying loop, derivation
If you observe a circular current carrying loop then you will find that it behaves like a magnetic dipole. Why am I saying this? If you look at the upper face, then you will find that the direction of the current is anticlockwise, so it has north polarity. But if you look at the lower face, then you will find that the current is clockwise, so it has south polarity or south pole.
That means the current loop behaves like a system of two equal and opposite magnetic poles, hence a circular current carrying loop is an example of a magnetic dipole.
The magnetic dipole moment of a circular current carrying loop is given as-
M=IA
Where the current flow through the current loop is, is the area enclosed by the circular loop, and is the magnetic dipole moment.
The magnitude of the magnetic field due to a circular current-carrying loop of steady current I and having radius R at any point on its axis at distance x is given by
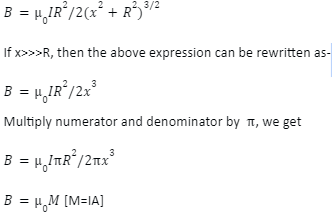
If the current loop has N turns, then the magnetic dipole moment
M=NIA
Conclusion
The magnetic dipole moment of a circular current carrying loop is defined as the product of the current flowing and area enclosed. Its direction is perpendicular to the plane of the loop and can be given by the right-hand thumb rule. The magnetic dipole moment of a magnetic dipole is defined as the product of its pole strength and magnetic length. It is a vector quantity, directed from S-pole to N-pole.
