Shear Modulus Elastic Moduli

One of the mechanical properties of solids that may be measured is the shear modulus of elasticity. Young’s modulus and bulk modulus are two other elastic moduli. The ratio of shear stress to shear strain in a body is determined by a material’s shear modulus.

It can be used to explain how a material resists transverse deformations, but it’s only useful for small deformations that don’t cause the material to revert to its previous state. This is because large shearing forces cause long-term deformations (no longer elastic body).

Shear modulus

A numerical constant that describes a solid’s elastic characteristics when applied transverse internal forces, such as those encountered when twisting a metal pipe about its longitudinal axis. Any small cubic volume within such a material is somewhat warped, causing two of its faces to slide parallel to each other a short distance and two other faces to transform from square to diamond forms.

The SI unit pascal, or Pa, is used to measure the modulus of rigidity.

Giga-Pascal is a common unit of measurement (GPa).

It can also be given in psi (pounds per square inch) (PSI).

Elastic modulus

Elastic modulus is defined as the ratio of stress to strain below the proportional limit. It is a measurement of a material’s rigidity or stiffness. The slope of the stress-strain curve in the range of linear proportionality of stress to strain in terms of the stress-strain curve is the modulus of elasticity.

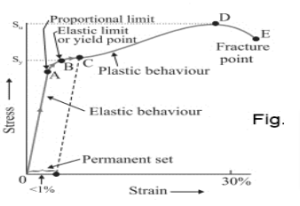
 A strain is defined as the ratio of the change in dimension induced to the initial dimension of the body. The known link between stress and strain is the modulus of elasticity.

E= StressStrain

SI unit=Nm-2

Dimensions=[ML-1T-2]

This can be used to explain how transverse deformations resist transformation. However, this is only practical for minor deformations.

There are three types of modulus of elasticity, which correspond to the three types of strain: longitudinal, volumetric, and shear. These are the following:

• Young’s modulus
• Bulk modulus
• Shear modulus

Young’s Modulus of Elasticity

The ability of a material to tolerate compression or expansion in proportion to its length is known as Young’s modulus. E or Y are used to represent it.

It’s used to describe the link between an object’s longitudinal stress and longitudinal strain. Deformation occurs whenever a load is applied to an item. If the thing is elastic, it can restore its original shape by reducing the pressure.

The formula for Young’s modulus is:

E=

σ=FA

ε=LL

Therefore,

E=σ(ϵ)=F/AΔL/L=FLAL

Here, 

E= Young’s modulus in Pascal

σ= Stress in Pascal

ε= Strain/ Proportional deformation

F= Force exerted on the object placed under tension

A= Cross-sectional area of the object

ΔL= Change in the length of the object

L= Original length of the object

The Modulus of Rigidity Formula:

G=Txyyxy=FlAx

Txy=FAwhich is the shear stress.

F= Force acting on the object

A= Area on which the force acts

Δx = Transverse displacement

l= initial length

Elastic modulus vs Shear modulus

The modulus of rigidity aids in calculating the deformation of an item when a deforming force is applied at right angles to the surface.

The modulus of elasticity aids in determining the deformations of an object when the deforming force is applied parallel to the surface.

Conclusion

The elastic limit (area OA) on the stress-strain curve below is essential to the structural and manufacturing industries because it describes the maximum stress a material can sustain before becoming irreversibly warped. Simply said, the modulus of elasticity is the stress-strain ratio. The three types of elastic moduli are Young’s modulus, Shear modulus, and Bulk modulus.

The shear modulus of elasticity is one of the mechanical properties of solids that may be measured. Other elastic moduli include Young’s modulus and bulk modulus. The shear modulus of a material determines the ratio of shear stress to shear strain in a body.

It can be used to explain how a material resists transverse deformations, however it’s only useful for tiny deformations before they may return to their original state. This is due to the fact that large shearing forces induce lasting deformations (no longer elastic body).