NEET UG » NEET UG Study Material » Physics » Equation Of State Of A Perfect Gas

Equation Of State Of A Perfect Gas

Equation of state of a perfect gas

Introduction

Here, in the topic Equation Of State Of a Perfect Gas, we are going to study about the concept of Ideal gas and the properties of an ideal gas. We will also derive the equation of the ideal gas. Also, we will study about the different gas laws in the simplest way we could with the help of different graphs. After studying all these topics, you will be able to answer all the questions related to the Equation of state of a perfect gas.

Body

The properties of the gases are entirely different from those of solids and liquids. In case of gases, thermal expansion is very large as compared to solids and liquids. To state the condition of a gas its volume and temperature must be specified.

At a given temperature for solid, liquid and gas:

  1. Internal kinetic energy should be the same for all.
  2. Internal potential energy is maximum for ideal gas (PE=0) and minimum for solids (PE= negative).
  • Internal energy is maximum for ideal gas and minimum for solids.

Notation:

µ = Molar amount of gas = M/Mw = N/N0

N0 = Avogadro constant = 6.022× 1023 molecules/moles

R = Universal gas constant = 8.31 J/mole-k

͌ 2 cal/mole-k, Dimensions = [ ML2T-2K-1]

r = Specific Gas constant (r= R/MW)

k = Boltzmann constant = (k= R/N0) = 1.38 × 10-23 J/kelvin

where;

m = mass of a gas molecule

M = mass of gas

MW= molecular weight

N = No. of gas molecules

n = molecular density (n= N/V)

= Gas density (

N.T.P

(Normal Temperature and Pressure)

S.T.P

(Standard Temperature and Pressure)

Temperature0⁰C = 273.15 K0.01⁰C = 273.16 K
Pressure

1 atm = 1.01325 × 105 N/m2

= 1.01325 × 105 pascal

1 atm
Volume22.4 litre22.4 litre

 

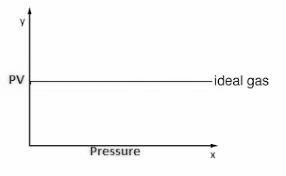
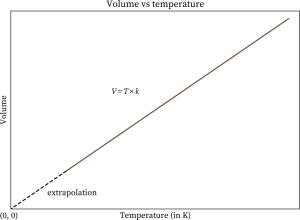
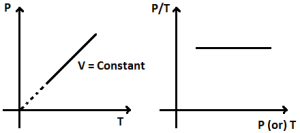
1 litre = 10-3 m3  = 103 cm3 = 103 cc = 103 ml

water = 1000 kg/m3 = 1 g / cc

ice = 900 kg/m3 = 0.9 g/cc

Define Ideal Gas/ Ideal Gas Concept

An ideal gas is one in which

●       Volume of gas molecules is negligible as compared to the volume of the container.

●       So volume of gas = volume of container (Except 0 K )

●       No intermolecular forces act between gas molecules.

Properties of Ideal Gas

●       A gas which follows all gas laws and gas equations at every possible temperature and pressure is known as ideal gas or perfect gas.

●       Ideal gas molecules can do only translational motions, so their kinetic energy is only translational kinetic energy.

●       Ideal gas can not be liquified because IMF(InterMolecular Forces) is zero.

●       Potential energy of ideal gas is zero so internal energy of ideal gas is perfectly translational K.E. of gas. It is directly proportional to absolute temperature.

So, internal energy depends only and only on its temperature.

Etrans∝T

For a substance U = UKE + UPE

UKE : depends only on T,

UPE : depends upon intermolecular forces (Always -ve)

●       Specific heat of an ideal gas is a constant quantity and it does not change with temperature.

●       All real gases behave as an ideal gas at high temperature and low pressure and low density.

●       Gas molecules have point mass and negligible volume and velocity is very high ( 107 cm/s). That’s why there is no effect of gravity on them.

Equation of state for ideal gas

We know that;

PV = µRT

=

Where;  µ= number of moles of gas

K = Boltzmann constant

Gas Laws

Boyle’s Law

According to it for a given mass of an ideal gas at constant temperature, the volume of gas is inversely proportional to its pressure, i.e.,

V

if m and T = Constant then;

P1V1 = P2V2

Charle’s Law

According to it for a given mass of an ideal gas at constant pressure, volume of a gas is directly proportional to its absolute temperature, i.e.

V

If m and p are constant then;

Gay Lussac’s Law

According to it, for a given mass of an ideal gas At constant volume, pressure of a gas is directly proportional to its absolute temperature. i.e.,

P∝T

If m and V = constant then;

Avogadro’s law

According to it, at same temperature and pressure, equal volume of all gases contains equal number of molecules, i.e.,

N1 = N2 ;  if P, V and T are same

Dalton’s Partial Pressure Mixture Law

According to it, the pressure exerted by mixture of non- reactive gases is equal to the sum of partial pressure of each component gases present in the mixture, i.e.,

P = P1 + P2 + ……

Properties / Assumptions of Ideal gas

●        The molecules of a gas are in a state of continuous random motion. They move with all possible velocities in all possible directions. They obey newton’s law of motion.

●        Mean momentum = 0;

Mean velocity

●       The average distance traveled by a molecule between two successive collisions is called as mean free path ( m) of the molecule.

●       The time during which a collision takes place is negligible as compared to time taken by the molecules to cover the mean free path. At NTP ratio of time of collision to time of motion to cover mean free path is 10-8 : 1

●       When a gas is taken into a vessel it is uniformly distributed in entire volume of vessel such that its mass density, molecular density, motion of molecules etc. all are identical for all directions, therefore root mean velocity

V2x  = v2y = v2z are equal. Pressures exerted by the gas in all directions Px = Py = Pz = P are equal.

●       All those assumptions can be justified , if number of gas molecues are taken very large i.e., 1023 molecules/cm3.

Conclusion

In this text we have discovered the equation of state of a perfect gas. We have learnt what an ideal gas is. We have seen its properties and also derived the equation for the same. Also, we looked into the different gas laws in our syllabus along with their graphs. Following we’ll also into some of the numerical fore the same.