Electric field due to a dipole

Electric dipoles are vital for understanding electrical phenomena in materials. We know that matter is made up of atoms and molecules, each of which has both positively and negatively charged nuclei. It has an internal or permanent dipole moment if the centre of mass of the positive nuclei coincides with the negative nuclei.

The dipole moments are arbitrarily oriented in the absence of an electric field, resulting in the system’s net dipole moment being zero.

When an electric field is applied to a system of charges within matter, the polar molecules align themselves in the direction of the electric field, resulting in a net dipole moment and the matter becoming polarised.

So, the electric field due to a dipole is the space around the dipole that can be affected by the dipole’s impact, so let’s talk about the electric field due to a dipole in detail.

Dipole: 

Dipoles are extremely prevalent. They are essentially two charges separated by some type of non-conducting medium (e.g., air, vacuum, etc.).
An example of a dipole can be seen in the electromagnetic wave, where a dipole in a uniform electric field is polarised. A dipole in an electromagnetic system deals with the positive and negative charges separated by a distance. It is characterised by their dipole moment, a vector quantity. 
The forces associated with electric fields are mediated by the movement of charge. In the case of a simple dipole, there will be an attractive force between the positive charge and the negative charge, even if they are very distant from one another.
The dipole moment is p = q x d
q = the magnitude of charge &
d = the separating distance.

Electric Field:

The strength of an electric field is measured in Volt/metre [V/(m)]. An electric field has both direction and magnitude, which means it can have different magnitudes, directions or even both in different regions of space.
The electric field lines are the trajectories of the electric force. Whenever an object with a charge moves through electromagnetic forces, it will follow these paths. The direction that the force is applied on determines which way the field line will be drawn (remember that like charges repel and opposites attract).

The electric field is explained as E = F/q
F = force exerts on the charge by an electric field
q = the charge

How to Calculate Electric Field Due to a Dipole?

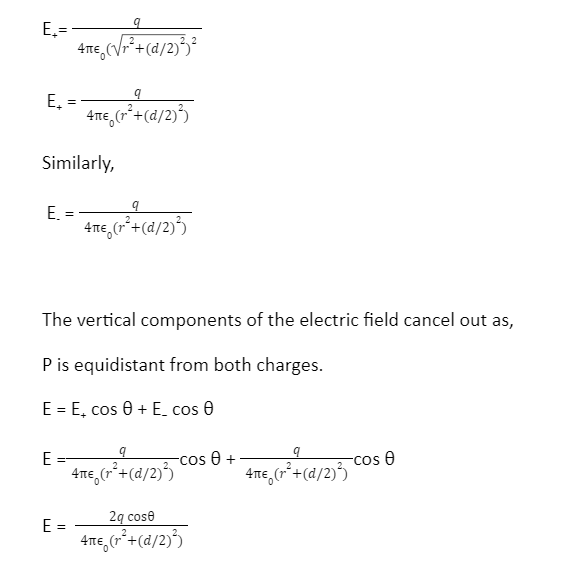
Consider a charged electric dipole +q and –q separated by a distance of d. For the purpose of simplicity, we will simply compute the fields along symmetry axes, that is, a point P along the dipole’s perpendicular bisector and a point Q along the dipole’s axis.

The electric fields due to the positive and negative charges (Coulomb’s law):

E+ = q40r+2 

E = 140pr3

Since it includes both positive and negative charges, the dipole’s field drops quicker than a point charge. Their electric fields would cancel out completely if they were brought to the same location. However, as they are separated by such little distance, they have a weak electric field.

Torque on Electric Dipole

An electric dipole is defined as an electric charge pair that are both electric and of similar magnitude but charges opposite that have the distance ‘d’ between them. A dipole happens when there is a separation in charge, like when someone says “positively charged” or “negatively charged”. Dipoles are pretty much the same thing as charge separation. Unlike other vectors, the moment of an electric dipole has a specific direction, namely from negatively charged to positively charged.

That is given as  p= qd

When there is a homogeneous electric field, the torque on an electric dipole is zero.

Now assume a dipole that has the charges +q and –q and separated by a distance of d. In this case, the dipole should be positioned in the electric field that is uniform in nature and has sufficient strength, represented by E, here the dipole’s axis generates an angle θ of the electric field.

An electric dipole in a uniform external field experiences a torque given as τ = pE sin θ, where the symbol θ  denotes the angle between p and E. The moment of the dipole p tends to align in the direction of E as a result of the torque. The symbol gives the potential energy, which is of the dipole Ue = −pE cos θ. 

Conclusion

When a dipole is far away, the electric field due to a dipole is always proportional to the dipole moment and inversely proportional to the distance cube.

The electric field due to the dipole is: