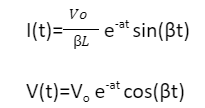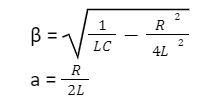Damped Oscillations

Oscillator: An oscillator is a semiconductor device that produces oscillations similar to sinusoidal waves.

Oscillation: It is a repetitive and periodic change of quantity with respect to time. It is measured with respect to some reference or central value. Mechanical oscillation is called vibration. Real examples of oscillation are AC (alternating current), pendulum, and heartbeat.

Oscillations can create waves. Some waves are visible, and some are not. The unit used for the number of oscillations per second is hertz. It is defined by quantities such as amplitude, frequency and time period.

Properties of oscillations

Frequency: It is defined as the number of oscillations per unit time.

Amplitude: It is defined as maximum displacement from its central position.

Time period: It is defined as the time taken for a complete oscillation in unit time.

The relation between time period and frequency is given by f=1/T

There are several types of oscillations, such as simple harmonic, damped, driven and coupled oscillations. In this topic, we’ll go through damped oscillations in brief.

Damping Force

If a force obstructs the motion of the body, then it is called a damping force. It acts in the opposite direction of motion.

When a damping force is applied to a damped oscillator, it is linearly dependent upon the velocity. The oscillation of the body exponentially decreases, which depends upon the damping coefficient. 

Damping force is denoted by Fd = p.v

where p = viscous Damping coefficient

 v = velocity of the object

Properties of damping force

• The damping force is directly proportional to the velocity of the object.

• The direction of the damping force is opposite to the velocity.

• The damping force opposes the motion of the body.

Damped Oscillations

In a damped oscillation, the amplitude decreases gradually and fades away with time. There are some mechanisms by which an oscillator loses energy, such as friction and radiation. For example, tuning a fork produces sound waves resulting in reducing energy. 

Due to the friction and radiation present in the circuit, amplitudes of oscillations decrease with time. The reduction in amplitude or energy of an oscillator is called damping, and this type of oscillation is called damped oscillation. Damping reduces the oscillation frequency. There are two types of damping: natural damping and artificial damping.

The damping ratio is defined as how oscillations decay due to disturbance. It is represented by £(zeta). It is a dimensionless quantity.

Examples are a weight on spring, a swinging pendulum and RLC (resistor, inductor, capacitor) circuit.

For mass M, which is weighted on spring, its period remains constant, but its amplitude decreases with time. Total forces that appear on the spring are the force of the spring and the damping force. If the amplitude of velocity is small, it oscillates slowly; thus, the damping force is proportional to velocity and acts against the direction of motion.

F = ma =  -kx

Here, the negative sign indicates the force is in the opposite direction of motion.

In an RLC series circuit, at t = 0, the time capacitor gets discharged through the resistor and inductor. In this condition, the equation of current and voltage given by

where

V=initial voltage

C=capacitance(farads)

R=Resistance (ohms)

L=Inductance(henrys)

e=base of natural log

The above current equation is for a damped sinusoidal wave. It represents a sine wave of maximum amplitude (V0/βL) multiplied by a damping factor of exponential decay. As a result, we get a waveform of oscillations bounded by a decaying envelope (gradually decreasing).

From the above equations, we can classify damped oscillations into three types.

Type of damped oscillations

Overdamped oscillation

When the R2C2-4LC term is positive, and a and b are positive numbers, oscillations are overdamped. In this case, the circuit does not show any oscillation.

Overdamped systems move towards equilibrium conditions more slowly compared to critically damped systems. For an overdamped system, the value of zeta £>1.

Underdamped oscillation

When the R2C2-4LC term is negative, and a and b are imaginary numbers, the oscillations are underdamped. In this case, the circuit shows an exponentially decreasing sinusoidal waveform. The underdamped system oscillates through the equilibrium position. For an underdamped system, the value of zeta<1. An example is a mass oscillating on the spring.

Critically damped oscillation

When the R2C2-4LC term is zero, then a and b are also zero. So, the oscillations are called critically damped. In this case, the circuit shows an exponentially decreasing narrow peak signal. The value of zeta for critically damped oscillation £=1.

Critical damping is the most desired situation as the system rapidly comes into equilibrium condition, and the force applied to the critically damped system moves the system to a new stable-equilibrium condition without undergoing an overshoot stage. 

An example of a critically damped system is the shock absorbers in a car. It is advantageous to have the oscillations decay as fast as possible. Here, the system does not oscillate but approaches the equilibrium condition as quickly as possible.

Undamped oscillations

A simple harmonic motion that is consistent at infinity without loss of energy or amplitude is called an undamped or free oscillation. For an undamped system, the value of zeta £=0.

Conclusion

In damped oscillations, forces such as friction and air resistance remove energy from the oscillator; due to that, the system response amplitude starts to decrease gradually with time. There are different types of damped oscillations, in which, depending upon the value of the damping ratio, their responses change. Thus reduction in amplitude is due to the energy loss caused by the external forces.