The term coulomb originated from a French physicist, Charles Augustin de Coulomb. The symbol of this unit is C; 1 coulomb is equivalent to 1 ampere-second.
To put this in words, an electric-powered current of 1 ampere represents 1 C of unit electric charges flowing across a selected point in 1 second. In terms of electrons, 1 coulomb of charge is the charge held by 6.24 x 1018 electrons.
Coulomb’s Law
Electrically charged particles constitute the foundation of this universe. The interaction of these charged particles gives rise to various phenomena like friction, induction, etc. Studying these laws led French physicist Charles-Augustin de Coulomb to formulate Coulomb’s law, perhaps one of the most well-known formulas. It quantifies the force that occurs when two charged particles interact. This law set the foundation for various electrostatic studies and even electromagnetic studies.
The regulation states that the significance of the electrostatic pressure of enchantment or repulsion among factor costs is immediately proportional to the magnitudes of charges and inversely proportional to the rectangular gap among them.
According to this law, there is an intended description when the two objects enact the role of point charges. Coulomb’s law as an equation:
F= k*Q1*Q2/ d²
In the equation,
F= Electrostatic force
k= is proportionality constant
Q1= Quantity of charge on object 1
Q2= Quantity of charge on object 2
d= Distance between the two objects by which they are separated.
The two Qs in the Coulomb’s charge represent the quantities of charge of two objects present in the time of interactions. These objects can be either positively or negatively charged. When there are positively charged particles, the charge is positive. When there are negatively charged particles, the charge is negative. The force value is positive when the two Qs are of the same charge and negative when they are of opposite charge.
Characteristics of Coulomb’s law
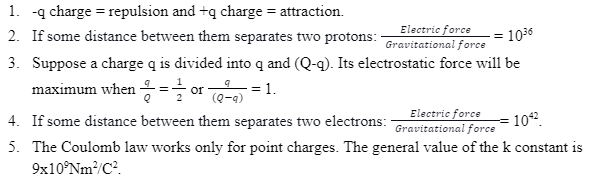
Limitations of Coulomb’s law
- The charges should be at rest and only point charges.
- The distance between the charges is supposed to be more than 10-15m(nuclear radius).
- The Coulomb Law of charges is not universal.
- If it is not possible to calculate the distance between the charges, the charges should be in an arbitrary shape.
- The equation of Coulomb’s law is applicable only when solvent molecules present between the particles are more significant than the point magnitude of the force between two point charges q1 and q2. This force will then be directly proportional to the product of the magnitudes and inversely proportional to the square of the distance between them.
Conditions for Stability
Let us consider if there are two charges placed in space, A and B, and these two charges are of equal magnitude and are of the same polarity.
The interaction of these two charges will create an electric field where the combined force will be felt due to the superposition principle. The superposition principle states that when two forces act on a body, the net force is the vector sum of the two forces. Now, if a third unit charge appears in the field of these charges, what conditions must be met such that the three charges are in equilibrium?
There are two movements that the third charge can make, an axial movement toward either of the two source charges and a perpendicular displacement between the two charges.
- When the third charge is placed such that it is at the centre of the two charges, it will experience the two forces Fa and Fb as equal.
- If the third charge appears closer to either charge, it will move from its initial position, and the magnitude of one force will increase, and that of the other will decrease.
- The third charge will not return to its initial position. Hence we can say that the axial equilibrium is unstable.
But if displaced perpendicularly, the magnitude of both the forces would change by an equal amount. Therefore, the two charges would bring the third charge back to its original position. Hence, the perpendicular equilibrium will be stable.
Superposition Principle
When several charges interact, the resultant force on a particular charge is the vector sum of the forces produced by the separate charges.
If several point-charges q1, q2, q3….qn simultaneously exert electric force on the charge q, then the net force on ‘q’ is obtained by taking the vector sum of the individual forces.
Conclusion
The Coulomb force between multiple charges is the foundation for many devices and observations. Understanding how forces operate when there are numerous sources of them is imperative.
Coulomb’s law, or Coulomb’s inverse-square law, is a practical law of physics that quantifies the quantity of force among stationary, electrically charged particles. The electric force among charged bodies at a static state is conventionally known as electrostatic or Coulomb.
The interaction of these two charges will create an electric field where we will feel the combined force due to both the charges due to the superposition principle. The superposition principle states that when two forces act on a body, the net force is the vector sum of the two forces.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



