Profile
Settings
Refer your friends
Sign out
Angular frequency can be defined as the angular displacement that takes place per unit of time. The angular frequency can also be defined as the rate at which the phase of a sinusoidal waveform changes. On multiplying frequency (v) by 2π, we can obtain the angular frequency. Therefore, it can be said that the angular frequency is greater than the frequency. The symbol of angular frequency is . It can also be termed circular frequency, radian frequency, angular speed, orbital frequency or radial frequency. Angular frequency can be expressed as radians per unit time. Since the SI unit of time is second, the SI unit of angular frequency can be written as radians per second.
Formula
The formula for Angular frequency (ω) is given by,
Deriving the formula
We know that speed (v) is given as,
v=∆D ⁄ ∆T
where ∆D denotes the change in distance, and ∆T denotes the change in time.
A particle travelling a circular path, which has a radius r, will travel a distance equal to the circumference of a circle, that is, 2πr. Also, let ∆T=t. Therefore, the equation for speed can be written as,
v=2πr ⁄ t
We also know that the velocity of an object that is travelling a circular path is given as
v=rω
where r represents the radius of the circle formed by the circular path, and represents angular velocity.
Equating both values of v, we get,
2πr ⁄ t=rω
Solving for , we get,
ω=2π ⁄ t
Problems
Example 1: Suppose that the time period of an oscillating object is 30 seconds. Calculate its angular frequency.
Solution:
Given: t=30s
We know that angular frequency is given by,
ω=2π ⁄ t
Substituting the value of t in the above equation, we get,
ω=2π ⁄ 30
Substituting the value of 2, we get,
ω=360° ⁄ 30
On simplification, we get,
ω=12°/sec
Example 2: Suppose a wheel rotates 8 times in a second. If the diameter of the wheel is 8 inches, find the angular frequency of the wheel.
Solution:
Given: f=8Hz
We know that angular frequency is given by,
ω=2πf
Substituting the value of f in the above equation, we get:
ω=2π(8)
On simplification, we get,
ω=16π
Example 3: Find the angular frequency of an oscillating mass that is tied to a string of length 2 metres.
Solution:
Given: l=2m
Difference between angular frequency and angular velocity
The rate at which angular displacement changes is termed angular velocity. Angular frequency or angular speed can be defined as the speed with which rotation takes place. Angular speed can also be defined as the magnitude of angular velocity.
Angular velocity is a vector quantity, while on the other hand, angular frequency is a scalar quantity. The formula for both angular velocity and angular frequency is the same. Also, both are represented by ω.
Types of angular frequency
- Circular motion
In a rotating object, we can obtain a relation between its tangential speed, distance from the axis and angular frequency (v=rω). Since speed is also equal to distance by time, and the distance travelled is equal to the circumference of the circular path, we can obtain another equation for speed. (v=2πr/t). When we equate both expressions for speed, we obtain angular frequency as:
ω=2π ⁄ t
- Oscillations of spring
Oscillations occur in an object attached to a spring. The formula for the angular frequency of an object suspended from a spring is given by:
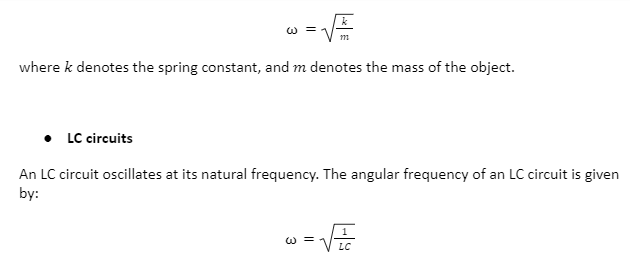
where L denotes the inductance of the circuit, and C denotes capacitance.
Conclusion
Angular frequency can be defined as the angular displacement that takes place per unit of time. It is, therefore, also termed angular speed. It is a scalar quantity. It is represented by the symbol and can be expressed as follows:
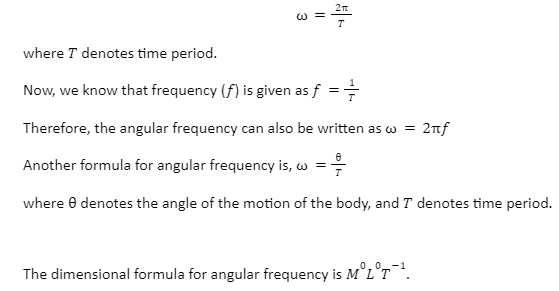
ω=2π ⁄ T
where T denotes time period.
In terms of frequency (f), the angular frequency can be expressed as:
ω=2πf
The angular frequency for an object suspended from a spring can be expressed as:
ω=√km
where k denotes the spring constant, and m denotes the mass of the object.
Also, the angular frequency for an LC circuit can be expressed as:
ω=√1LC
where L denotes the inductance of the circuit, and C denotes capacitance.
Angular frequency represents the magnitude of angular velocity. The formula for both quantities is the same; however, the former is scalar while the latter is a vector quantity.
