Profile
Settings
Refer your friends
Sign out
There are several applications of trigonometry that are used daily. An example of this could be a person standing on the roof of a tall building, and the height is known, and he sees an on the other side, calculation about the width of road can be done via the formulas constructed.
The trigonometric formulas are used in astronomy and are useful when the distance between stars and satellites is calculated. Some other fields where trigonometric applications could be used are flight engineering, construction, criminology, marine biology, etc.
DOES TRIGONOMETRY PLAY A ROLE IN EVERYDAY LIFE?
Trigonometry might not have a real amicable use in real-time and may not pertain to solving practical issues in daily life. Still, it is an active part of some activities enjoyed daily. An example that can be cited for this is music as sound has a wave pattern although not as regular as basic sine and cosine but is still amicable in the development of computer music.
A computer being a machine, cannot listen to like a human being, but what it can do is interpret and represent the sound waves in mathematical order. The calm music that soothes the soul of workers when they are on relax mode is all through the properties of trigonometry.
EQUATIONS IN TRIGONOMETRY
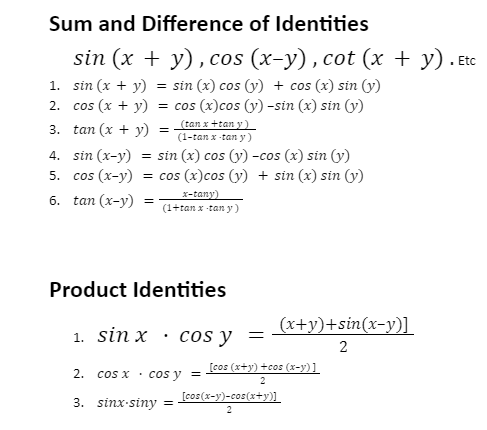
Formulae about trigonometry are as follows:
Basic Formulas
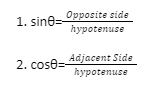
Sine and Cosine Laws
Sine laws: This pertains to the relationship between angles and sides of the triangle.
Cosine Law: It helps evaluate side length for the other two sides given lengths, and the angle included.
Example:
- a²=b²+c²–2bccosA
- b²=a²+c²–2accos B
c²=a²+b²–2abcosC
Reciprocal Identities
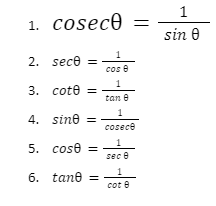
Trigonometric ratio Tables
Angles (Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
Angles (Radians) | 0° | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π |
sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
cosec | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1
|
Cofunction Identities(Degrees)
- cos (90 °–x) =sin x
- tan (90°–x)=cot x
- cot (90°–x )=tan x
- sec (90°–x) =cosec x
cosec90° –x=sec x
Real-life applications of trigonometry
Astronomy: Spherical trigonometry has been used for solar, stellar and lunar position localization. It makes it handy for eclipse prediction and observation of planet orbits.
Navigation: Trigonometry functions are applicable for Sextants, a tool actively used for measuring the angle of stars or the sun concerning the horizon. Trigonometry can also be used for the localisation of latitudes and longitudes.
Surveying: Surveying land trigonometric functions used lengths, areas, and relative angles between objects calculations. In geography, it was used for measuring the distance between landmarks.
Periodic functions: Sine and cosine functions are important for periodic function theory, particularly for describing sound and light waves. Fourier discovered that functions such as continuous periodicity could be a sum in infinite numbers about trigonometric functions. Non-periodic functions are also crucial for sines and cosines via the Fourier transform. The applications it pertains to are quantum mechanics, communications, and other fields.
Optics and acoustics: Functioning to trigonometry is also important in physical sciences such as optics and acoustics. They describe sound and light waves and solve transmission and boundary-related problems.
Criminology: Trigonometry can be a good part when the trajectory of a projectile is tracked r mapped. It particularly estimates what could cause the collision in a car accident or how the object fell, how and at which angle the bullet was shot, and others.
Other applications: Additional fields where trigonometry and its functions are quite amicably used are geodesy, music theory, audio synthesis, architecture, electronics, biology, medical imaging, chemistry, cryptography, seismology, meteorology, game development, etc. many other fields.
Conclusion
Trigonometry is used in our day to day life and is not only a part of a high particle or high-level physics. The application is real-time based and pertains not only to a right-angled triangle but also other types of triangle, in the details mentioned above and brief knowledge of trigonometric identities and functions such as basic formulas, trigonometric ratio table, co-function identities, reciprocal identities, periodic identities, sum and difference identities, half-angle, double angle, triple-angle product identities, and the sum of product identities. The above article gave the knowledge on interpreting the formulae and the Inverse trigonometry formula. A trigonometric formula list and table were also given in the above article.
