Many certain trigonometric functions can be expressed in terms of another trigonometric function. These relations are typically called inverse trigonometric functions because they reverse the original relationship between the two trigonometric functions, performing an “inverse” to one of the given elements. These inverse relationships are useful only for special purposes and not general use, with a few exceptions.
Inverse trigonometric functions are mathematical functions that reverse the sign of one of the given trigonometric functions. For example, inverses to cosine and sine are called cosine inverses and sine inverses. Inverse trigonometric relations hold between two or more functions or formulae.
Trigonometry
Trigonometry is the study of how to measure the lengths of sides and angles in a triangle.
Sine of an angle, also called “sin,” is one of the trigonometric functions. It specifies how much the side opposite to an angle differs from a line drawn from its vertex to the opposite side. The sin function is denoted with small case s.
Formula: The function formula of sine is given by
Sin(A) = opposite/hypotenuse.
Inverse Trigonometry
Inverse trigonometry is the act of reversing the sign of one element in a trigonometric function. It is also referred to as the reciprocal of an angle. The inverse trigonometry expressions are used when an angle/triangle or a trigonometric value must be reduced or simplified to one that can be easily represented using arithmetic operations. Another way that inverse trigonometry can be used is when solving a problem that relates angles to trigonometric functions.
The Relation of Trigonometry and Inverse Trigonometry
Inverse trigonometry plays a special role in trigonometry. Going from trigonometry to inverse trigonometry and back is known as the use of “trigonometry .”Therefore, inverse trigonometry is essential in using trigonometry, as it provides information about the values for the horizontal and vertical lengths of a figure.
Different methods are used to identify which function has an inverse relationship to another.
We can identify which function is the inverse of another by reversing the arrow.
The original trigonometric relationship would be written with a positive angle and an expression in terms of unknowns and variables. The inverse relationship would be written with a negative angle and known values.
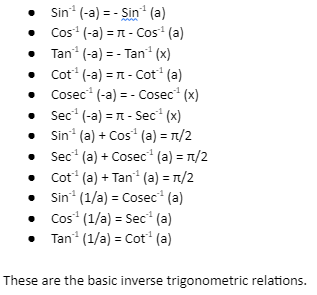
Inverse Trigonometry Relations
Inverse trigonometry relations of a triangle:
Inverse trigonometry consists of opposite operations to the actual trigonometric functions.
For instance,
- Sin-¹ performs the opposite operations of sine.
- Cos-¹ performs the opposite operations of cosine.
- Tan-¹ performs the opposite operations of tangent.
Let’s learn about the different trigonometric relations.
Finding the Trigonometric Formula of an Angle
You can find the trigonometric function of any angle when the lengths of its sides are known. In general, the sine, cosine, and tangent of an angle are related to its length in the following way:
“The sine of an angle is equal to one half of the length of its opposite side divided by the length of its hypotenuse .”The opposite side can be found by subtracting the opposite angle from 90 degrees.
Finding the Trigonometric formula of a quadratic form: You can find the trigonometric function of any quadratic if you know its height and one of its sides.
″The height or vertical distance of a quadratic is equal to one half of the sum of its horizontal distance and degree”.
Inverse Trigonometry used in the Calculation of the Area of the Triangle
Area of triangle = ½ * base * height.
Sometimes, the task is to find a side of a triangle when given its area.
The sides of the right angle triangle are mainly calculated under trigonometry. Inverse trigonometry is used to find the value of the angle in the right angle triangle. Normal trigonometry functions are used to find the sides of the triangle.
Inverse sine
If the perpendicular side of the triangle and the hypotenuse of the triangle is also given, then its angle is found using inverse sine. The formula of inverse sine is:
Sin-¹(Perpendicular / Hypotenuse) = θ
The domain of sine inverse is (-1, 1).
Inverse cosine
The reciprocal of the sine function is the cosine. Suppose the perpendicular side and the hypotenuse are given. Then, the angle of the triangle is found using the formula:
Cos-1(Hypotenuse / Perpendicular) = θ
The domain of cosine inverse is (-1, 1).
Inverse secant
If the hypotenuse and base of the triangle are given, then the angle of a triangle can be calculated using the formula:
Sec-¹(Base / Hypotenuse) = θ
The domain of secant inverse is
Inverse cosecant R – (-1, 1).
R = infinity
Inverse cosecant
Cosecant is the reciprocal of secant. Similar to secant, if the base and hypotenuse of the triangle are given, then the angle of the triangle can be calculated using the formula:
Cosec-¹ (Hypotenuse / base) = θ
The domain of inverse cosecant is R – (-1, 1).
R = infinity
Inverse tangent
If the perpendiculars and base of the triangle are given, then its angle can be calculated using the formula:
Tan-¹(perpendicular / base) = θ
The domain of inverse tangent is infinity.
Inverse cotangent
Cotangent is the reciprocal of a tangent. So, if the base and perpendicular are given. Then, the angle is calculated with this formula,
Cot-¹(base / Perpendicular) = θ
The domain of inverse cotangent is infinity.
An acute angle measures between 0° and 90°, and its sides do not share the same rays. An acute angle is the smallest angle between the ray.
The obtuse angle measures between 90° and 180°, and its sides do not share the same rays. An obtuse angle is larger than an acute angle but smaller than a right angle. The symbol α denotes obtuse angles.
The ascending side of an acute or a right triangle is shorter than the hypotenuse.
Conclusion
In simple words, inverse trigonometry is the reciprocal of normal functions of trigonometry. In trigonometry, the angle of the trigonometric functions is used to find the value of sides. On the other side, in inverse trigonometry, the sides of the triangle are used to find the value of its angle. Trigonometry is used in performing various space-related calculations. It is widely used in astronomy. The whole concept of Inverse trigonometry functions is based on the three-sided right-angled triangles as the word “Trigo” itself means three, which depicts computations with three sides. Trigonometry makes all the triangle-related calculations very easy.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



