Everything You Must Know About The Application Of Derivatives!

A common term used in Mathematics, Derivatives, may seem to be non-significant. But, in reality, it is one of the most important ones. The significance of derivatives is due to their extensive applications in the subject. In the world of calculus, derivatives are a fundamental tool. It is believed so because it is used to find numerous quantities. For example, to find an object’s velocity, the derivative of position is taken with respect to time. 

Whether we talk about Mathematics or daily life, derivatives have numerous uses. One of these uses is finding maxima and minima using derivatives. The process used to find derivatives is known as differentiation. 

What are derivatives?

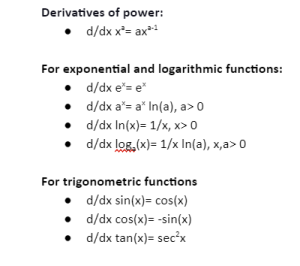
The rate of change of a quantity (y) with respect to another quantity (x) is known as a derivative. The term differential coefficient is also used to describe a derivative. To find derivatives of a function, a process called differentiation is used. 

Generally, derivatives can be known as the functions of various real variables. It means that they are reinterpreted as a linear transformation. The graph of a linear transformation is the approximation of the function’s graph to get the best linear approximation. Primarily, the derivatives are used when there is the presence of varying quantities, and the rate of change of this quantity is not constant. 

Types of derivatives

1. First-order derivatives: These are the derivatives that tell about a function’s direction and whether the function is increasing or decreasing. This derivative can be interpreted as an instantaneous rate of change. The slope of the tangent line can also be used to predict first-order derivatives. 
2. Second-order derivatives: The second-order derivatives are used to get an idea of the graph shape of a given function. The second-order derivatives use concavity to classify functions. 

Applications of derivatives

Besides finding minima and maxima, derivatives have numerous other significant applications in Mathematics and daily lives. Derivatives are popularly used in engineering, science, physics, and numerous others. In mathematics, their applications are numerous and also significant. For example, they are used to find the quantity change rate or to find the maxima and minima. Some of the standard applications of derivatives are: 

1.To find the rate of change of a quantity

Derivatives are utilized to track down the rate of quantity changes with respect to another quantity. We can track down an approximate change in one quantity when the other changes by utilizing the derivatives. 

2.For finding tangent and normal to a curve

Derivatives are also used to find the equation of a normal and tangent line to the curve of a function. In other words, if at any given point we have to find the equation of a tangent, then we can use derivatives to find the slope and equation of the tangent line. The slope at a point is equal to the curve’s derivative at that point. 

3.For approximation

Linear approximation of a function can be found with derivatives at a given value. While giving the linear method of approximation, Newton suggested finding the approximately close value to the function, finding the function’s value at a given point, and finding the equation of the tangent line. Derivatives can find this equation of a tangent line. Hence, derivatives are used to find the closest approximated value. 

4.For finding maxima and minima

With the first order-derivative test, we can find the maximums and minimums of a curve. The maxima and minima of a curve are the peaks and valleys. The test for finding minima and maxima includes equating the function’s derivative with 0, i.e., f(c)= 0, after finding it at a given point. Equating it with 0 means that the slope of the curve is zero. After this, in a given interval in which the function is defined, we can check the f (x) values at the left and right points of the curve. Also, we can check the nature of f (x). Hence, based on the following conditions, we can say if the given point is maxima and minima. 

• The point is maxima if the f (x) or slope changes from positive to negative while moving via point c, here, f(c) is the maximum value
• The point is maximal if the f (x) or slope changes from negative to positive while moving via point c,f(c) is the minimum value here

5.For finding point of inflection

Point c referred to in the above application of finding the maximums and minimums is known as the point of inflection when the sign does not change while moving via point c. It is the curve part where the curve changes its nature, i.e., from concave to convex or from convex to concave. 

Conclusion

In mathematics, derivatives are characterized as the strategy that shows the concurrent rate of change. That implies it is utilized to address the sum by which the given quantity changes at one point. The above listed are some of the numerous applications of derivatives in Mathematics. Apart from finding the maxima and minima, they are also used for finding increasing and decreasing functions.