Profile
Settings
Refer your friends
Sign out
We see a lot of enclosed figures around us. These figures can be 2-dimensional and 3-dimensional as well. All the diagrams which can be represented using the two axes are called the two- dimensional figures like – circle, square, rectangle, triangle etc. On the other hand, the figures which need 3- dimensions or 3 axes for representation are called the 3-dimensional figures like – sphere, cuboid, cube, ellipsoid etc. So, basically, whenever we have an enclosed area, an area which is surrounded by boundaries, by curves or by lines, we can always find the area of that geometrical figure. If the structure is not in any uniform shape, it is distorted at various places, we can still find the very small areas and add them together which is called the process of integration, and get the approximate area of that figure.
Area of a Triangle
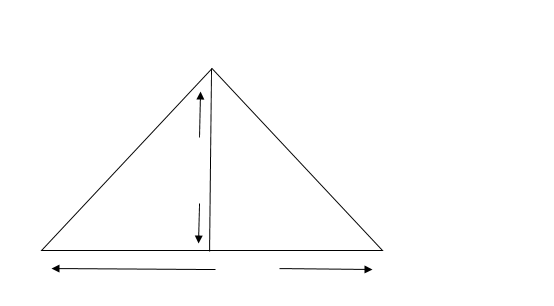
Triangle is a simple two-dimensional figure enclosed by 3 lines only. To find the area of a triangle, we need its height and base. The base of the triangle is the line on which the angle is mounted upon. When we have to measure the angle of a triangle, we always measure it by putting the base line of our protractor on the baseline of the triangle. In the following figure, we see the baseline on which the angle is mounted upon, is the line BC. The height of triangle is the line which is perpendicular to the base line.
Area of a triangle = 1 ⁄ 2 × b × h
Also, we can say that half of the multiplication of the base and height of the triangle, is the area of that triangle. The unit if the area is square unit. Because it is a multiplication of two sides. So, it can be meter square or centimeter square as per the given sides.
On the basis of length of the sides, there are 3 kinds of triangle- scalene triangle, isosceles triangle and equilateral triangle.
As per our above formula, we can derive the formula for these three triangles separately.
A scalene triangle is a triangle which has three sides and all of them are unequal with respect to each other. Also, all the angles of the scalene triangle are dissimilar to one another. The formula for scalene will be the same as we discussed above.
Formula for Isosceles triangle- A isosceles triangle is a triangle which has three sides, and of these 3 sides, two sides are equal to each other. Also, the angle made by the two equal sides, is called the ‘vertex’ and the angle by the third side is known as ‘base angle’. To find the area of isosceles triangle, we have the formula-
Area of triangle = 1 ⁄ 2 × b × h
Now, we can get the value of height by the Pythagoras theorem, h= √a²–b ⁄ 2²
Putting this value in our formula, we get, area of triangle = 1 ⁄ 2 × b × a²– b ⁄ 2²
= 1 ⁄4 b √4a²–b² sq unit
Formula for equilateral triangle- The equilateral triangle is a triangle which has three sides and all three sides are equal. Because having three equal sides, this kind of triangles also have equal angles, that’s why these are also called the ‘equiangular’ triangles. Each angle of the equiangular triangle is 60°. We know that each triangle has 180° in total. So, if we divide that in three angles, it becomes 60° itself. To find the area of an isosceles triangle, we again use our standard formula of triangle –
Area of triangle = 1 ⁄ 2 × b × h
Now, we can get the value of height by the Pythagoras theorem, h= a²–a ⁄ 2²
= 1 ⁄ 2× a ×√a² –a ⁄ 2²
= 3 ⁄ 4 × a² square unit
Area of a triangle by Heron’s Formula-
A way to find the area of a triangle is, with the help of semi-perimeter of the triangle. The semi-perimeter of a triangle is half of the perimeter of the triangle. Semi-perimeter is represented by ‘s’. So, the formula to find semi-perimeter of a triangle,
Is, s= a+b+c ⁄ 2
The area of triangle = √s(s-a) (s-b) (s-c)
Here, a, b and c are the sides of the triangle.
Perimeter of triangle–
Let us suppose, we have a triangle with 3 sides a, b, c. So, we calculate the perimeter by the formula,
Perimeter = a + b + c
We just add all three sides to get the perimeter of a triangle.
Conclusion
We have different ways and different formulas to find the area of a triangle. We use the most appropriate one as per given conditions. We have to first figure what kind of triangle is given to us and what sides are given in question to find the values which we can put in our formula. We also get to know that the standard formula of finding the area of a triangle helps us in all three triangles- scalene, isosceles and equilateral triangle.
