This article aims to help CBSE students understand the concepts of Analytical Geometry, Three Dimensions (or 3D) in Analytical Geometry, and Three Dimensions – Distance Between Two Points.

To represent (or locate) any point or object in space, the knowledge of 3D Coordinate (or Analytical Geometry) is required. Similarly, to calculate the distance between two objects (or points) in space, the knowledge and formula of three dimensions – the distance between two points is required.

The three dimensions – the distance between two points say, P (x1, y1, z1) and Q (x2, y2, z2) is the shortest distance (d) between them and is given by –

PQ = d = √ [(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2].

Introduction to the Concept of Analytical Geometry

  • Analytical Geometry in mathematics uses algebraic symbols and methods to represent and solve an equation for a problem in geometry.
  • Analytical geometry is commonly known as Coordinate geometry and sometimes as Cartesian geometry.
  • Analytical geometry is the study of geometry by using a coordinate system.
  • Applications of Analytical geometry: 
  • Its concept is used in physics, engineering, aviation, rocketry, space science, and spaceflight. 
  • It provides the foundation for most modern geometrical fields such as – algebraic geometry, differential geometry, discrete geometry, and computational geometry.
  • Analytical geometry establishes a correspondence between geometric curves and algebraic equations, making it possible to reformulate problems in geometry in terms of equivalent problems in algebra and vice versa.

Introduction to the Concept of Three-Dimensional Coordinate System

  • To represent (or locate) any point or object in space, the knowledge of 3D Coordinate (or Analytical Geometry) is required.
  • In the three-dimensional coordinate system, the coordinates of a point A are represented as A (x, y, z). The point exists in an XYZ plane where x, y, and z represent the distance of point A from the Origin in X, Y, and Z coordinate axes, respectively.
  • The coordinates of the origin in the 3D coordinate system are O (0, 0, 0).
  • The x, y, and z axes divide the XYZ plane into eight equal parts. Each part is known as an octant.

Introduction to the Concept of Three Dimensions – Distance Between Two Points

  • To calculate the distance between two objects (or points) in space, the knowledge and formula of three dimensions – distance between two points are required.
  • The three dimensions – the distance between two points P (x1, y1, z1) and Q (x2, y2, z2) is the shortest distance (d) between them.
  • The distance formula for three dimensions – distance between two points P (x1, y1, z1) and Q (x2, y2, z2) is given by –

PQ = d = √ [(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2].

  • The distance formula for three dimensions – distance between two points P (x1, y1, z1) and origin O (0, 0, 0) is given by –

d = √ (x2 + y2 + z2).

Derivation of the distance formula for Three Dimensions – Distance Between Two Points

Repeated Application of Pythagoras Theorem

Consider the figure given below. We need to find the distance (say, d) between the points P (x1, y1, z1) and Q (x2, y2, z2), represented by the line PQ.

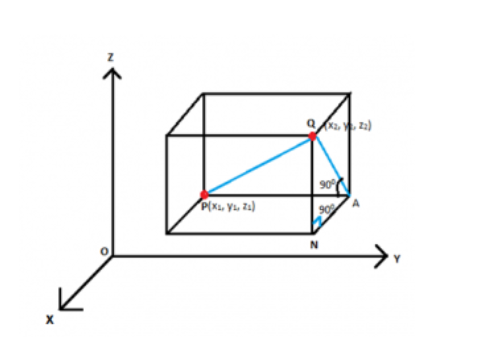
The figure shows that PQ is a diagonal of the rectangular parallelepiped and that / PAQ is a right angle.

From this, we can use the Pythagoras theorem in /\PAQ. Now, we have,

PQ2 = PA2 + AQ2 – (i)

Similarly, by using Pythagoras theorem in right-angled /\ANQ, we have,

AQ2 = AN2 + NQ2 – (ii)

Substituting value of AQ2 from (i) in (ii), we have,

PQ2 = PA2 + AN2 + NQ2

We can see that, PA = y2 – y1, AN = x2 – x1, NQ = z2 – z1.

Hence, PQ2 = (x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2

By doing square root of both sides, we have,

PQ = d = √ [(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2].

2D distance formula

Consider the figure below. We need to find the distance (say, d) between two points with coordinates (x1, y1, z1) and (x2, y2, z2) and the distance is represented by the red line.

By using the 2D distance formula, the length of the yellow line can be given as √ [(x2 – x1)2 + (y2 – y1)2]. And the length QR = (z2 – z1).

Then, by using Pythagoras theorem in right-angled /\PQR, we have,

d2 = {√ [(x2 – x1)2 + (y2 – y1)2]}2 + (z2 – z1)2

By doing square root of both sides, we have,

d = √ [(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2].

Conclusion

Through this article, CBSE students can understand the concepts of Analytical Geometry, Three Dimensions (or 3D) in Analytical Geometry, and Three Dimensions – Distance Between Two Points.

They can derive the distance formula by either repeated application of the Pythagoras theorem or by using the 2D distance formula.

Students have learnt that the three dimensions – the distance between two points say, P (x1, y1, z1) and Q (x2, y2, z2) is the shortest distance (d) between them and is given by –

PQ = d = √ [(x2 – x1)2 + (y2 – y1)2 + (z2 – z1)2].