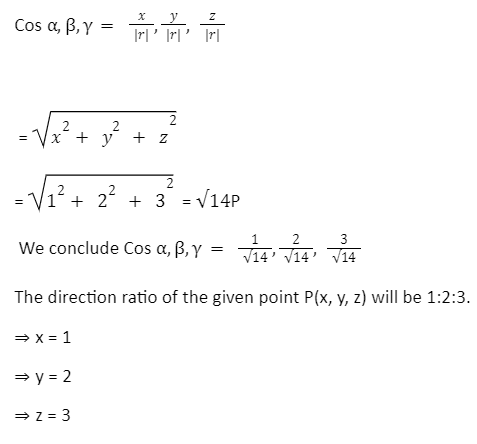DIRECTION COSINES AND DIRECTION RATIO OF LINE

DEFINE THE DIRECTION COSINES OF A LINE

• Any vector that indicates its position concerning origin is called a position vector.
• Before talking about the directional cosines, consider a position vector.
• Consider three planes named x,y,z in the 3 d space with O as the origin and OQ is the reference line, and the length of the OQ line is q.
• Q is the vector in space with variable q.
• When a directed line OQ passes through the origin makes α, β, and γ angles with the x, y, and z-axis, respectively, with O as the reference.
• α, β, and γ angles are called e direction angles of the line, and the cosine of these angles gives us the direction cosines.
• Suppose we extend the line OQ on 3 d places to know about the directional cosines. So that we can take to supplement angle β as shown in the figure.
• The direction line gets reversed if we reverse the direction of the line OQ.
• After considering the angle made by the position vector and the direction of the line in the positive quarters, we can consider the position vectors of the line OQ.

If OQ = q, then from the above figure, we can see that

x= qcosα

y = qcosβ

z= qcosγ

Where k denotes the magnitude of the vector, and it is given by,

• q – cosines of direction angles of a vector with a coefficient of the unit vector
• Unit vector q – is resolved by the rectangular components

The number that is proportional to the direction of cosine is called the direction ratio of the line. The direction ratio of the line is represented by a,b,c

The value of k can be positive or negative based on the direction of the directed line

The angle between OA and OQ is

The angle between OQ and OB is

The angle between OB and OC is

FOR EXAMPLE

Consider the point P(x, y, z) having coordinates (1, 2, 3). Find the direction cosines of the line and the direct ratio with the origin point being O(0, 0, 0) for the given values in the question

DIRECTION COSINE AND DIRECTION RATIO DOESN’T PASS THROUGH THE ORIGIN

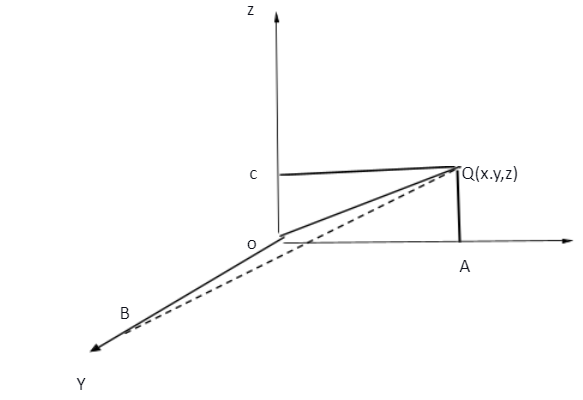
• We have seen what happens if the direction cosines and direction ratios of a line pass through the origin; what happens if it doesn’t pass through the origin.
• If it is also very simple, we can create the friction line as the 2nd line passes through the origin instead of the original line.
• Same as the above case, consider three planes named x,y,z in the 3 d space with O as the origin and OQ is the reference line, and the length of the OQ line is q
• Q is the vector in space with variable q
• When a directed line OQ passing through the origin but instead of the original line, the frictional line makes α, β, and γ angles with the x, y, and z-axis, respectively with O as the reference
• Let us assume that the magnitude of the vector is “q,” And the vector makes angles α, β, γ with the coordinate axes x,y,z

DIRECTION RATIOS WHEN THE LINES DOESN’T PASSES THROUGH THE ORIGIN

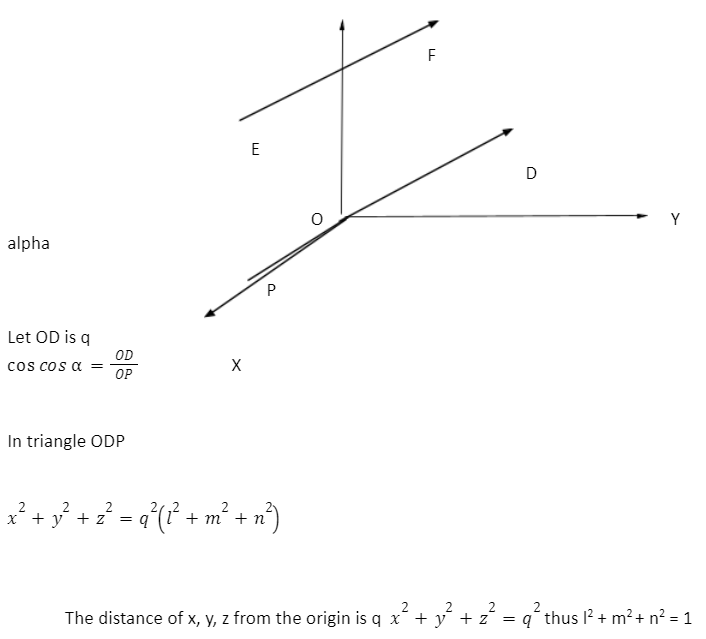
RELATION BETWEEN DIRECTIONAL COSINES

Let’s assume we have a line EF with directional cosines l, m, and n. Draw a line through the origin parallel to this line, and let’s take D(x, y, z), a point on this line. We draw a perpendicular from D to the x-axis.

Z

Conclusion

Direction cosine is a vector that represents the direction of a line in a 3-D plane. If the angle line makes with x-axis= α  with y-axis is β  and with z-axis is γ then cos2α +cos2β +cos2γ=1. The direction ratio is just another way to represent the direction of a line. Its advantage is we don’t have to deal with fractions for direction.