Profile
Settings
Refer your friends
Sign out
The rate constant is denoted by k and known as reaction rate constant or specific reaction rate. Rate constant can be calculated by using the Arrhenius equation or by using the molar concentrations of the reactants and the order of the reaction.
Characteristics of rate constant
It is the measure of the rate of a reaction. Greater is the value of the better-understood reaction that has a particular rate constant value at a specific temperature.
The value of rate, constant f, or the same reaction, changes with temperature.
The value of the rate constant for the reaction does not depend upon the concentration of the reactants.
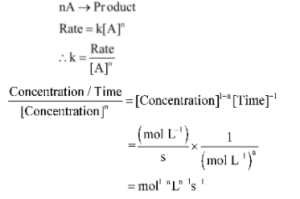
Unit of the rate constant
For the nth order of a reaction.
Thus, the dimensions of k are equal to,
The unit of the rate constant is dependent on the order of the reaction.
Units of rate constants for gaseous reactions
In the case of gaseous reactions, the concentrations are expressed in terms of pressure in the units of the atmosphere. Therefore, the rate has the units of atm per second. Thus, the unit of different rate constants would be –
For reaction with order 0, the unit of rate constant is M s-1 (or) mol L-1 s-1.
For reaction with order 1, the units of the rate constant is s-1.
For reaction with order 2, the unit of rate constant is M-1 s-1 (or) L mol-1 s-1 .
For reaction with order n, the unit of rate constant is M1-n s-1 (or) L(-1+n) mol(1-n) s-1.
Rate Law
Rate law may be defined as a mathematical expression representing the observed or actual rate of reaction. Let’s consider a reaction in which the rate equation of a reaction in which b, a, c and d are the various stoichiometric coefficients is expressed as
Rate ∝ [A]x[B]y ⇒ Rate = k[A]x[B]y;
Here, the primary equation that we considered was;
aA + bB → cC + dD.
Where,
The quantities of the reactant molecules A and B are denoted by [A] and [B].
The partial reaction orders for reactants A and B are denoted by x and y. (which may or may not be equal to their stoichiometric coefficients a & b).
The rate constant of the reaction is the proportionality constant ‘k’.
The difference between the rate law and the specific rate constant may seem obscure, but it’s really quite simple. The rate law is a general statement that describes how the speed at which a chemical reaction occurs varies with respect to a variety of factors such as temperature and pressure. However, the specific rate constant considers only one factor: the concentration of the reactants.
Reaction Orders
Reaction order is the power to which concentration is raised in a rate law equation. The order explains how and why the proportion of a reaction rate influences the rate law numerically.
There are several ways to express the rate law equation, so let’s begin with the most basic one: R = k[A]n
As a result, when the order is one, or when n = one, it indicates that the correlation between Reactant A concentration and the rate of the reaction is precisely proportional. When A rises, R rises in tandem. R doubles if A doubles.
Rate Constants
The rate constants for zero, first, second, and nth-order reactions are listed below in their units.
For reaction with order 0, the unit of rate constant is M s-1 (or) mol L-1 s-1.
For reaction with order 1, the units of the rate constant is s-1.
For reaction with order 2, the unit of rate constant is M-1 s-1 (or) L mol-1 s-1 .
For reaction with order n, the unit of rate constant is M1-n s-1 (or) L(-1+n) mol(1-n) s-1.
In a rate equation, each reaction has its own constant. The particular rate constant (k) is indeed a proportionality constant that will be distinct to each experimentation. This implies that its value is influenced by other parameters in the experiment, such as temperature, that affect the response rate. When other rate-altering variables vary, k may change even when the same molecules are utilized in the reaction.
Differential Rate Equations
Reactant concentration changes (d[R]) are utilized by differential rate law to represent reaction rate as a function of short-term variations (dt) in reactant concentrations. As a result, the differential form of the rate expression in the preceding subsection is:
-d[R]/dt = k[A]x [B]y
By putting a rate and the appropriate concentrations into a variable rate law and solving for k, we may get a rate constant.
Calculating the instantaneous rate of a reaction, which is the rate of a reaction occurring within a very short period of time, may be accomplished using differential rate equations.
Integrated Rate Equations
The concentration of the reactants in a chemical reaction is expressed as a function of time using integrated rate equations. As a result, rate equations may be used to determine how long a certain percentage of the reactants will be consumed in a chemical process. It’s worth noting that integrated rate equations for reactions of various orders differ.
For a zero-order reaction, the integral rate equation is:
k.t = [R0] – [R]
(or)
k = ([R0] – [R])/t
For first-order reactions, the integrated rate law is:
k.t = 2.303log([R0]/[R])
(or)
k = (2.303/t).log([R0]/[R])
The integral rate equation for second-order reactions is:
k.t = (1/[R]) – (1/[R0])
Integrated rate law
The integrated rate law is obtained by integrating the differential rate equation to determine the concentration at different points and the rate constant. It is complicated to decide on the reaction rate from the concentration-time graph. For reactions of other orders, we observed other integrated rate laws.
Conclusion
In this article, we learned about rate constant, its units and the factors affecting the rate constant and the rate of a reaction. Rate law is defined as a mathematical expression that represents the rate of reaction in terms of concentrations of the reacting species. We also learned about the order of reaction and zero, first, second and nth order reaction. By integrating the differential rate equation, we obtain the integrated rate law as determining the rate of reaction from concentration – the time graph is very difficult.
