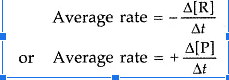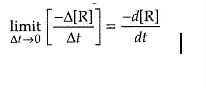First order reaction

Only one reactant’s concentration determines the outcome of a first-order reaction. As a result, a first-order reaction is often known as a unimolecular reaction. Other reactants may be present, but they will all be zero-order because their concentrations have no effect on the rate. As a result, the rate law for a first-order elementary reaction with regard to a reactant A is:

         The rate constant, k, must have units of concentration/time, which it does in this example with units of 1/s.

Chemical Reactions Calculated by Reaction Rate:

1.Reactions that are quick or instantaneous: Fast reaction is a chemical reaction that takes less than Ips (10-12 s) to complete. The speed of such processes, such as ionic reactions, is almost hard to measure. Reactions of organic substitution

2. Slow responses: Slow reactions are chemical reactions that take a long time to complete, ranging from minutes to years. Consider the corrosion of iron. Diamond metamorphosis, for example.

3. Reactions that are moderately slow: Moderately slow reactions are chemical reactions that fall between slow and fast reactions.

Unit of rate constant for first order  reaction:

For a first-order reaction, the rate constant (k) Equals min–1 or s-1.

• A first order reaction’s rate constant has only one time unit. It doesn’t have a concentration unit.

• This means that for a first order reaction, the numerical value of k is independent of the unit in which concentration is expressed.

• The numerical value of k for a first order reaction does not change if the concentration unit is modified.

• It would, however, change if the time unit was changed. If k is  6.0 x 10–3 min, it can also be expressed as 1 x 10–4 s–1, which means that if the time unit is changed from minute to second, the numerical value of k will decrease 60 times.

Half-Time or Half-Life Period of a First order Reaction:

A reaction’s half-time is defined as the time it takes to lower the reactant’s concentration to half of its initial value. The symbol t1/2 is used to represent it.Thus,for 1st order reaction we know that,

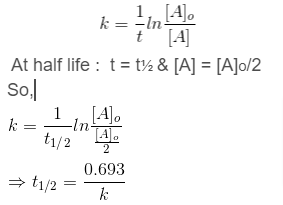
 At half life :  t = t½ & [A] = [A]o/2

So,

• Because k is constant for a given reaction at a given temperature and the expression does not include a concentration term, the half-time of a first order reaction is constant regardless of the initial concentration of the reactant.

• This indicates that if we start with 4 mol/L of a reactant reacting by first-order kinetics and reduce it to 2 mol/L  after 20 minutes, it will take another 20 minutes.

• That is, after 20 minutes from the start of the reaction, the reactant concentration will be 2 mol/L, and after 40 minutes, the concentration will be 1 mole/L. The reactant concentration will be lowered to 0.5 mol/L after 60 minutes from the commencement of the reaction. In other words, if 50% of the reaction completes in 20 minutes, then 75% completes in 40 minutes, 85.5 percent completes in 60 minutes, and so on, as illustrated in the graph below.

Examples of first order reaction:

1.Nitrogen Pentoxide Decomposition Examples (N2O5)

                    2N2O5 → 2NO2 + ½ O2

2.In aqueous solution, ammonium nitrite decomposes.

               NH4NO2 → N2 + 2H2O

3.H2O2 decomposition in aqueous solution.

               H2O2 → H2O + ½ O2

4.Ethane hydrogenation.

           C2H4 + H2 → C2H6

5.Diazo derivatives hydrolysis.

      C5H5N = NCl + H2O → C6H5OH + N2 + HCl

How to solve first order reactions:

                      2N2O54NO2 + O2

The balanced chemical equation for dinitrogen pentoxide breakdown is shown above. The rate rule for this reaction has the following general form because there is just one reactant:

                            Rate=k[N2O5]m

The value of the exponent m must be determined in order to determine the overall order of the reaction. We can achieve this by measuring the rate at which N2O5decomposes once an initial concentration of N2O5 is measured in a flask. The reaction can then be repeated, but with a different beginning concentration ofN2O5. The new rate at which the N2O5decomposes is then measured. We can determine the order of the decomposition reaction by comparing these speeds.

For Example:Assume that the rate of decomposition of N2O5at 25 °C is 1.4 10-3M/s and that the initial concentration of N2O5is 0.020 M. Let’s imagine we repeat the experiment at the same temperature, but this time we start with a different concentration of N2O5(0.010 M). We find that the rate of breakdown of N2O5is 7.0 10-4M/s in the second trial. We can now calculate the first rate’s ratio to the second rate:

                                 Rate1=k[N2O5]i1mRate2=k[N2O5]i2m

                                  1.4 10-3=k[0.020]m7.0 10-4=k[0.010]m

The rate constants cancel on the right side of the equation, while the left side of the equation is simply equal to 2. Everything boils down to:

                  2.0=2.0m

The decomposition is clearly a first-order reaction, with m=1.

Important points:

• The rate of a first order reaction is influenced by the concentration of only one ingredient.

• Any first-order reaction has a single reaction order.

• For first order reactions, the rate constant (k) is measured in seconds.

• t1⁄2 = 0.693/k is the half-life period of a first-order reaction.

Conclusion:

The index, or exponent, to which the concentration term in the rate equation is elevated in chemical kinetics is defined as the order of reaction with regard to a specific material.The reaction orders diverge from the stoichiometric coefficients, as they do for many reactions. Only via experimentation can reaction orders be discovered. Their information helps them to draw conclusions about the reaction mechanism and may even aid in the identification of the rate-determining step. Reaction orders for elementary reactions are equal to the stoichiometric coefficients for each reactant, whereas reaction orders for complicated reactions are not always equal to their stoichiometric coefficients.