Introduction to Refraction
Refraction is the bending of a light wave as it transitions from a single medium to another. Like reflections, refraction occurs in different types of waves such as sound and water. Refraction of sunshine is the foremost typically observed phenomenon, but other waves like sound waves and water waves also experience refraction. The index of refraction is the ratio of the light speed in a vacuum to the speed of light in a medium, µ = c/v.
Refraction through a prism occurs when light passes through a glass prism and refracts both entering and exiting the prism. The emergent and incident rays are not parallel because the entry and exit (refracting surfaces) are not parallel to each other. Let’s read about refraction of light, refraction index, and so on.
Laws of Refraction
The law of refraction simply governs the overall light behaviour as it passes through two different media. It is also known as Snell’s law.
Willebrord Snell, a Dutch scientist, developed a law stating that the ratio of sines of angle of incidence i and angle of refraction (r) is identical to the ratio of velocities (v1 / v2) in the two mediums, or equivalently, to the opposite ratio of indices of refraction (n2 / n1):
n = sin i/sin r
Refraction in Rarer Medium
When a wave bends and moves from one medium to another, its speed varies. Refraction is the bending of a wave when it enters a medium with a different speed. The refractive index of the medium and the angle between the ray and the line perpendicular (normal) to the surface separating the two media determine how a wave bends.
The medium with a higher refractive index is denser, while the media with a lower refractive index is rarer. Wave speed is slower in a denser medium than in a rarer medium. When a wave moves from a denser to a rarer medium, it bends away from the normal, resulting in an angle of refraction higher than the angle of incidence. The refracted ray bends towards the normal when the wave moves from a rarer to a denser medium, and the angle of refraction is less than the angle of incidence.
Sign Conventions for Refraction at Spherical Surfaces
We use the following sign convention when researching refraction at spherical surfaces:
- All distances are calculated from the spherical refracting surface’s pole.
- Distances measured in the direction of light incidence are considered positive, while distances measured in the opposite direction of light incidence are considered negative.
- Positive distances are those measured in a perpendicular direction above the primary axis. Distances measured in the perpendicular direction below the principal axis are given negative values.
When considering refraction at spherical surfaces, we make the following assumptions:
- The spherical refracting surface has a tiny aperture.
- The incident and refracted rays produce tiny angles with the spherical surface’s primary axis, resulting in sini≈i and sinr≈r.
When considering refraction from a rarer to a denser medium, two instances may arise: refraction from a rarer to denser medium at a convex spherical surface and refraction from a rarer to denser medium at a concave spherical surface. Similarly, when examining refraction from a denser to a rarer medium, there are two possibilities: refraction from a denser to rarer medium at a convex spherical surface and refraction from a rarer medium at a concave spherical surface.
What happens in a prism?
The “dispersion of light rays” is the main phenomenon in a prism. The main source of dispersion is a change in media. When a light beam changes medium, it is how it is refracted based on its wavelength and frequency. When the light ray passes through the prism, it is refracted and distributed into the band of 7 different colours known as the Rainbow colours or VIBGYOR when it emerges from the prism medium.
Applications of Refraction at Spherical Surfaces
Refraction at spherical surfaces is useful in a variety of scenarios. Here are a few of them:
- It aids in the estimation of the behaviour of light rays travelling through various lenses.
- It explains how light rays behave when they enter the second medium, which has a different refractive index.
- The formula for a lens as a whole entity is obtained by combining the refraction relations at spherical surfaces of both lens surfaces.
Conclusions
With this, we come to the end of the topic of refraction and its laws. Refraction is the change in direction of a wave caused by a change in wave speed. We hope that we were able to assist you in fully comprehending the notion of refraction at spherical surfaces. We discussed here the derivation of the relationships that control refraction at spherical surfaces, as well as their application in solving numerical problems. We also discussed here the refraction of light and refraction index.
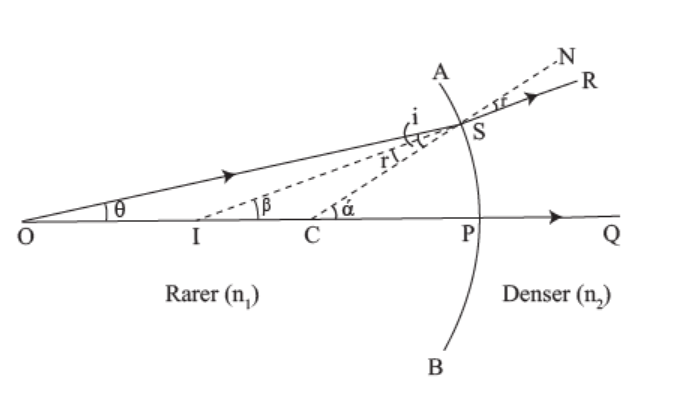
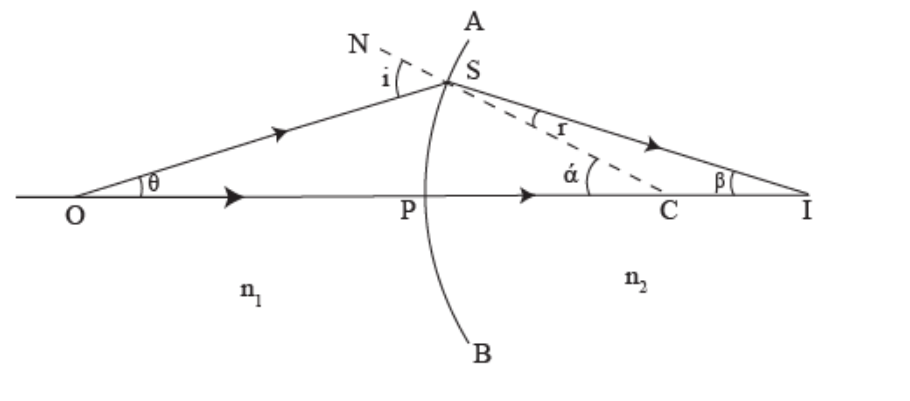
At point O, a point object is placed on the principal axis of the concave surface.
The ray OS, incident at the point S of the concave surface, travels along SR after refraction. As n2>n1, the refracted ray bends towards the normal CSN. Another ray OP, incident normally on the concave surface, is undeviated. The two refracted rays do not intersect in reality but appear to meet at point I in Medium 1.
Thus, I is the virtual image of the object placed at O.
In Figure,i=∠OSC,r=∠NSR=∠ISC,
PO= object distance =-u,
PI= image distance =-v
and radius of curvature =PC=-R.
Let SCP=,∠SIP= and SOP=. Snell’s law is applied at the point of refraction S,
n1sini=n2sinr.
For paraxial rays or small aperture, i and r will be small. Hence, sinii and sinrr. Making this assumption, we can write as
n1i=n2r.
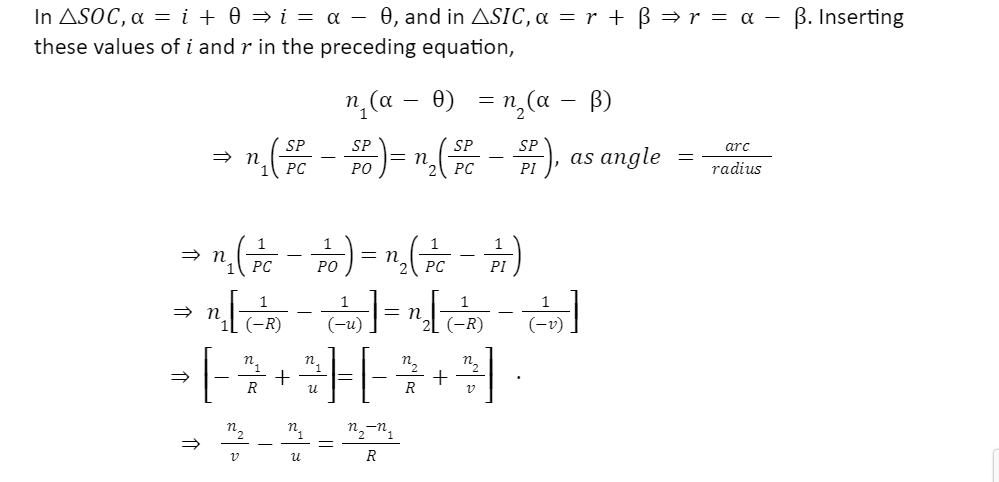
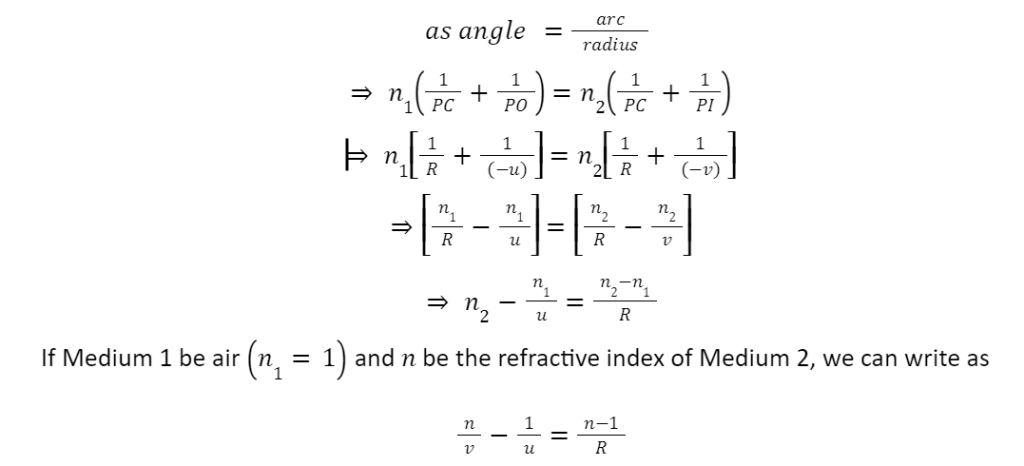
If Medium 1 be air n1=1 and Medium 2 has a refractive index n2=n, then we can write the above Equation as
n/v – 1/u = n-1 / R.
When an object is placed in the denser medium, the image of the object is formed by a concave surface dividing the two mediums. This is shown in the Figure below.
Refraction at a convex surface:
When light refracts on a convex surface, it creates a real or virtual image. We will discuss the two cases separately.
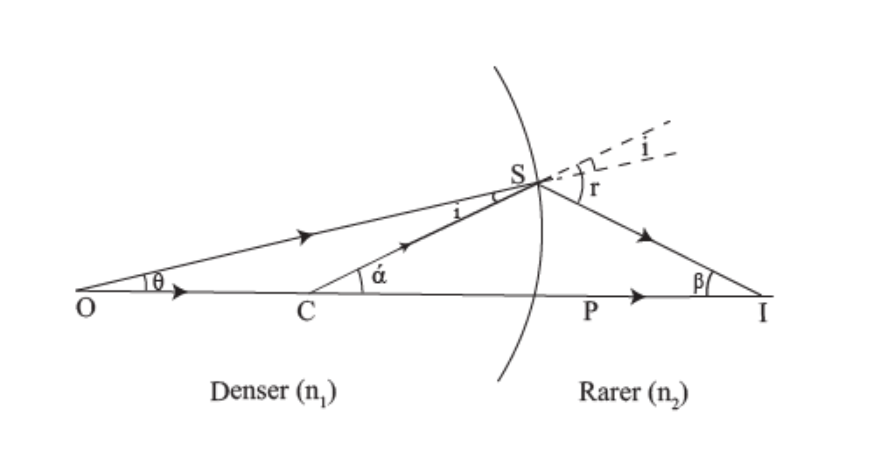
When the image is virtual: Consider a convex surface AB separating two media of refractive indices. When n2n2>n1, as shown in Figure.
P is pole, and C is the centre of curvature of the convex surface.
At the point 0, point objects are placed on the principal axis of the curved surface. The ray OS, incident at the point S on the convex surface, travels along ST after refraction.
As n2>n1, the refracted ray bends towards the Normal CSN.
Another ray OP incident, normally on the convex surface, is undeviated.
The two refracted rays do not intersect in reality but appear to meet at point I.
Thus, I is the image of the object placed at O.
OSN=i,∠CST= NSI =r
PO=-u,PI=-v ,PC=+R.
Let SCP=,∠SIP= and SOP=.
Applying Snell’s law at the point of refraction, n1sini=n2sinr.
For paraxial rays, i and r will be small. Hence, sini=i and sinr=r. Making this substitution in Equation n1sini=n2sinr.
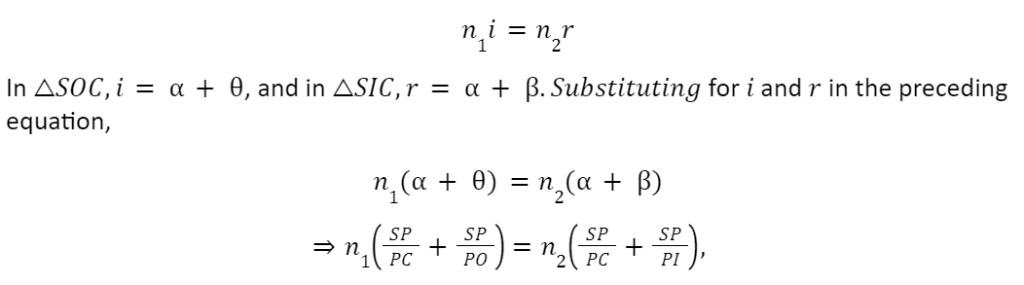
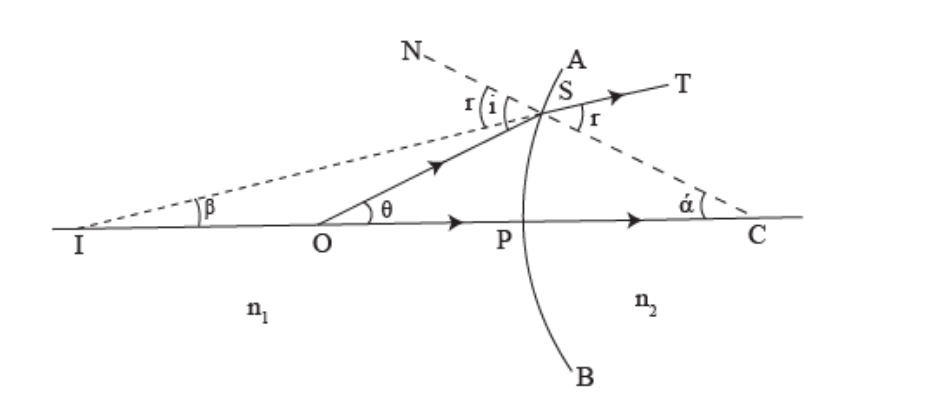
When the image is real: Consider a convex surface AB separating two media of refractive indices n1 and n2 (n2>n1), as shown in Figure.
Let P be the pole and C be the centre of curvature of the convex surface. Let a point object be placed at point O on the principal axis of the convex surface. The ray OS, incident at S, goes along SI after refraction at S. As n2>n1, the refracted ray bends towards the normal CSN. Another ray OP, undeviated. The two refracted rays intersect at I in Medium 2 . Thus, I is the real image of the object placed at O.

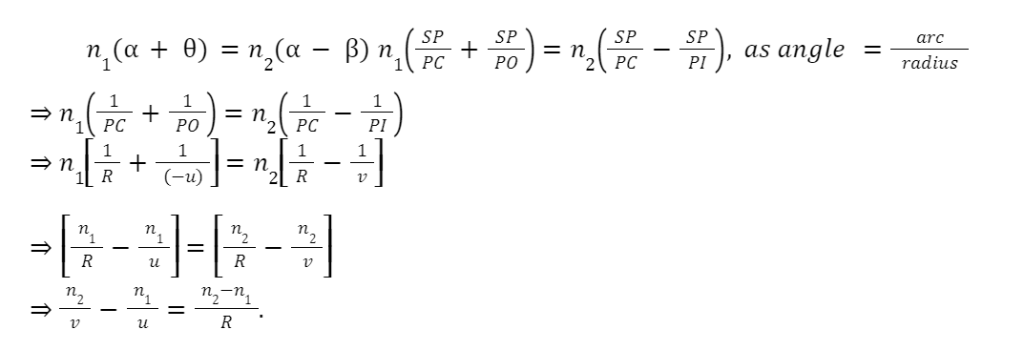
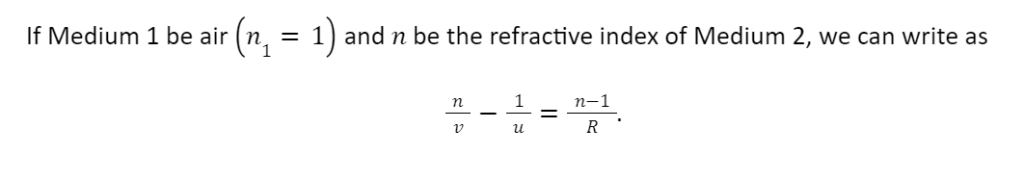
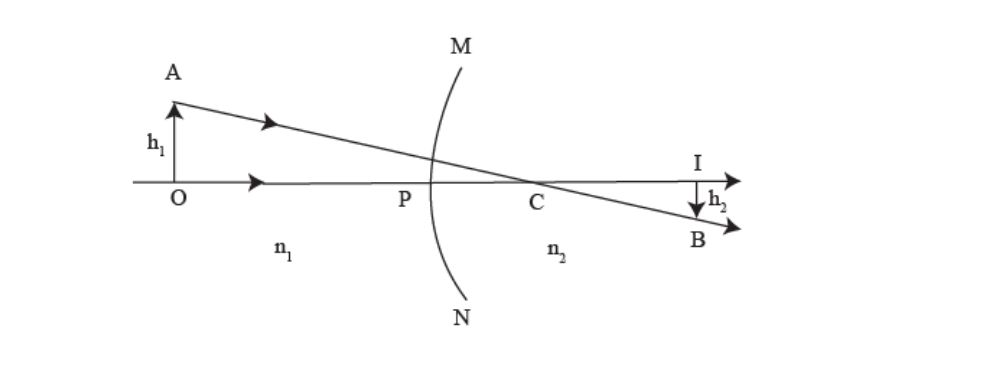
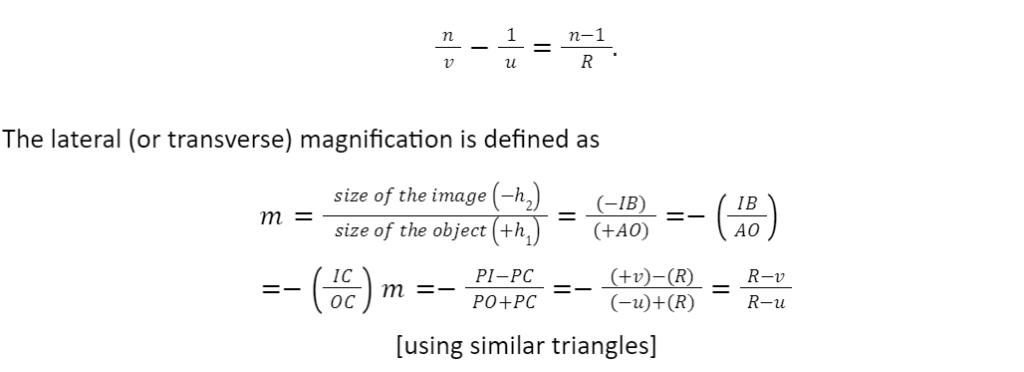
Lateral magnification:
Consider an extended object AO placed in Medium 1 (refractive index n1 ) facing a convex spherical surface MPN of Medium 2 (refractive index n2 ). Rays originating from A and O directed towards centre C will travel undeviated into Medium 2. Let the image formed be BI, as shown in Figure.
Its position may be located by using Equation
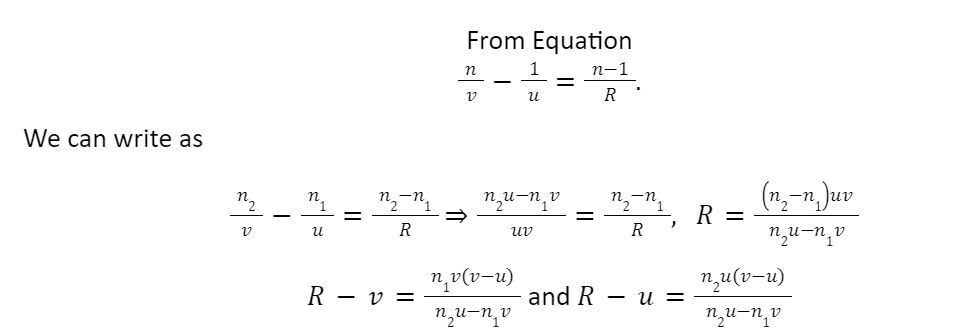
Thus,
Hence,
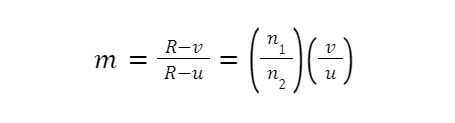
Conclusion
With this, we come to the end of the topic of Refraction at Spherical Surfaces and by Lens. Refraction is the bending of light (it also happens with sound, water and other waves) because it passes from one item into another. We hope the topic is clear to everyone.
 Profile
Profile Settings
Settings Refer your friends
Refer your friends Sign out
Sign out



